Why is this continuum circle-like?

Question

A continuum is a compact connected metrizable space.

A continuum $X$ is called arc-like if for every $\varepsilon>0$ there is an open cover $U_1,\ldots,U_n$ of $X$ such that the diameter of $U_i$ is smaller than $\varepsilon$ for every $i$ and $U_i\cap U_j\neq\varnothing$ iff $|i-j|\leq 1$.

A continuum $X$ is called circle-like if it satisfies the same property with the additional requirement of $U_1\cap U_n\neq\varnothing$.

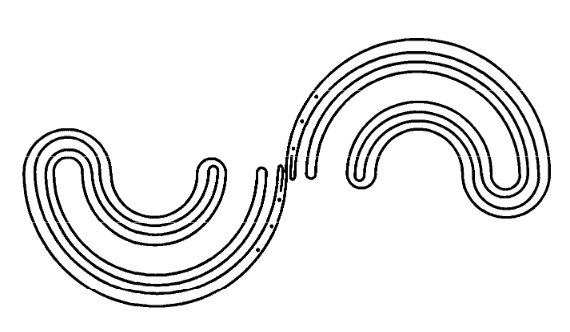
I was recently surprised by the fact that a continuum $X$ can be both arc-like and circle-like, with the example given by Illanes and Nadler in Hyperspaces being the following continuum, obtained by attaching together two copies of the bucket handle continuum at their endpoints:

I can more or less see that this space is arc-like, but why is it also circle-like?

Note that, since this space is arc-like, its Čech cohomology must be trivial, which implies that even though every open cover is refined by one whose nerve is a circle, the maps induced by circle-like refinements of circle-like covers on the cohomology of their nerves must eventually be trivial. In other words given $\varepsilon>0$ and an open circle-like cover $U=\{U_1,\ldots,U_n\}$ with Lebesgue number $\delta$ and $\mathrm{diam}(U_i)<\varepsilon$ for every $i$, we can find $\varepsilon'<\min\{\varepsilon,\delta\}$ sufficiently small, so that any circle-like cover $U'$ consisting of pieces with diameter smaller than $\varepsilon$ is such that $U'$ is "contractible" in $U$, that is it doesn't go all the way around the "hole" of $U$. An answer illustrating this behaviour for some pair of $\varepsilon'<\varepsilon$ would be particularly appreciated.

Answer 1

Yes, this continuum is circle-like.

You say you can already see why the continuum is arc-like. For a given $\varepsilon > 0$, find an open cover $U_1,U_2,U_3,\dots,U_n,U_{n+1}$ witnessing, in the normal way, that the continuum is arc-like for this $\varepsilon$. The key observation is that $U_1$ and $U_{n+1}$ are very close together, so that their union has a diameter of something like $4\varepsilon$. Then consider the new open cover $U_1 \cup U_{n+1}, U_2, U_3, \dots, U_n$. This new cover witnesses that the continuum is circle-like for $4\varepsilon$ instead of $\varepsilon$.

In the picture below, I've drawn what I think of as the standard way of seeing this continuum is arc-like. The two darkly shaded balls are then combined to get a cover showing it is circle-like.