5
$\begingroup$

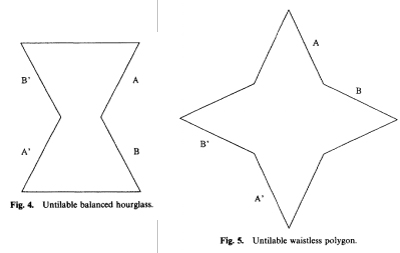
I saw this problem some years ago, don't remember the source:

Let $P$ be a Jordan polygon (i.e. the only points of the plane belonging to two edges are the polygon vertices) that can be tiled with parallelograms.

  1. Does it follow that the sum of the areas of all the rectangles among those parallelograms is the same independent of the tiling?
  2. Does it follow that the sum of the areas of all parallelograms with angles $\alpha$ and $\beta$ is the same independent of the tiling?

I couldn't find a solution, but I believe the answer to both questions is positive.
Does anyone know how this problem can be solved?

Improve this question
$\endgroup$
5
  • $\begingroup$ Isn't the sum of all areas the area of P? What am I missing? $\endgroup$
    – Wolfgang
    Commented Aug 14, 2021 at 18:56
  • 1
    $\begingroup$ @Wolfgang No, it's not. The parallelograms are not necessarily equiangular. $\endgroup$
    – jack
    Commented Aug 14, 2021 at 19:12
  • $\begingroup$ @Wolfgang: I misread in the same way. Perhaps clearer would be: "of all the rectangles among the parallelograms in the tiling"---Only some (perhaps none) of the parallelograms are rectangles. $\endgroup$
    – Joseph O'Rourke
    Commented Aug 14, 2021 at 23:00
  • $\begingroup$ Thank you. I have taken the freedom to edit the question. $\endgroup$
    – Wolfgang
    Commented Aug 15, 2021 at 5:44
  • $\begingroup$ The ideas used there may be useful here too: mathoverflow.net/questions/381781 $\endgroup$
    – Yaakov Baruch
    Commented Aug 15, 2021 at 8:24

2 Answers 2

Reset to default
4
$\begingroup$

When you tile a simple polygon by parallelograms, the tiling can be partitioned into "zones" of rectangles with parallel sides, meeting end-to-end, and forming a path from one edge of the polygon to a parallel edge of the opposite orientation. Two parallel zones cannot cross, so the pairing of opposite edges into zones is uniquely determined. Additionally, two non-parallel zones cross either zero times or one time, according to whether the edges at the ends of the zones appear in nested or alternating order around the polygon. That is, the number of crossings is again uniquely determined by the polygon.

You get exactly one rectangle for each two zones that are determined by perpendicular edges of the polygons and that cross each other. The shape of this rectangle is just the Cartesian product of the two perpendicular edges, so it is also determined. So the shapes of all rectangles in the tiling, and not just the sum of their areas, is equal for all tilings. The same goes for parallelograms with other angles.

Improve this answer
$\endgroup$
3
$\begingroup$

Not every simple polygon can be tiled with parallelograms.

Kannan, Sampath, and Danny Soroker. "Tiling polygons with parallelograms." Discrete & Computational Geometry 7, no. 2 (1992): 175-188. DOI.

Kenyon, Richard. "Tiling a polygon with parallelograms." Algorithmica 9, no. 4 (1993): 382-397. DOI.

Here are two untilable examples from the Kannan et al. paper:

     UnTilable

Improve this answer
$\endgroup$
4
  • 1
    $\begingroup$ Thanks for the reference. However in this problem it's assumed that this specific simple polygon $P$ can be tiled with parallelograms. It's an initial condition. $\endgroup$
    – jack
    Commented Aug 14, 2021 at 16:28
  • $\begingroup$ Ah, then the phrase "a Jordan polygon can be tiled with parallelograms," should be rephrased because it is false. What you meant is "Let $P$ be a Jordan polygon that can be tiled with parallelograms." $\endgroup$
    – Joseph O'Rourke
    Commented Aug 14, 2021 at 16:52
  • 2
    $\begingroup$ Of course not every: a triangle can not. I guess this is example when for each direction there are more than one sides parallel to this direction, and none of them is longer than the others together. $\endgroup$
    – Fedor Petrov
    Commented Aug 15, 2021 at 7:13
  • $\begingroup$ @IlkkaTörmä: Thanks for catching that; fixed now. $\endgroup$
    – Joseph O'Rourke
    Commented Aug 26, 2021 at 23:55

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .