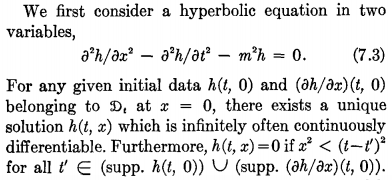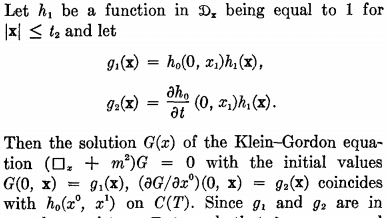Propagation of Klein-Gordon solutions in extra dimensions

Question

In his paper "Von Neumann Algebras of Local Observables for Free Scalar Field" Araki used the solutions of the equation

$$\frac{\partial ^{2}h}{\partial x^2}-\frac{\partial ^{2}h}{\partial t^2}-m^2h=0$$

where he put the suport of the initial data $h(t, 0)$ and $\frac{\partial h}{\partial x}(t, 0)$ at $x=0$.

Then He used this solution (noted as $h_{0}$) to pose another Klein-Gordon Problem in $\mathbb{R}^{4}$: $$(\Box+m^2)G=0 $$ with initial conditions

$G(0,\vec{x})=h_{0}(0,x_{1})h_{1}(\vec{x})$ and $\frac{\partial G}{\partial x^0}(0,\vec{x})=\frac{\partial h_{0}}{\partial t}(0,x_{1})h_{1}(\vec{x})$

where $h_{1}$ is a function wich is equal to 1 in a ball (of radius $t_{2}$) which contains the suports of the initial conditions for $h_{0}$ and then goes to zero smoothly.

Araki asserts that $G(x^0,x^1,0,0)=h_{0}(x^0,x^1)$ for $|x^0|+|x^1|<t_{2}$, where $t_2$ satisfies $h_{1}(\vec{x})=1$ for $\|\vec{x} \|<t_{2}$.

My question is why the extra dimensions doesn't affect the equality, at least for near points. I know the initial contitions for $G$ has translation simmetry in $x^3$ and $x^4$ and I think this would be important.

Some parts of Araki's paper

And the problematic part for my

Many thanks in advance.

Answer 1

Klein-Gordon equation has finite speed of propagation, which implies that if two solutions have initial data agreeing on the set $\{|\vec{x}| < t_2\}$, then the two solutions agree on the set $\{|x^0| + |\vec{x}| < t_2\}$.

Your solution $G$ has initial data that agrees with the solution $H(x^0, \vec{x}) = h(x^0, x^1)$, and the result follows.

($H$ is a solution by symmetry reduction.)