I would like to know the convergence radius of the following two double power series of $(x,y) \in \mathbb{C}^2$:
\begin{align} \sum_{m,n=0}^\infty \frac{(d-a)_{n+m}(d+b)_{n+m}(d+a)_n(d-b)_n}{n!m!(2d-c)_n(d)_{2n+m}}x^ny^m \end{align}
\begin{align} \sum_{m,n=0}^\infty \frac{(n+m)!(n+m)!}{(2n+m)!m!}x^ny^m \end{align}
where $(u)_v:=\Gamma(u+v)/\Gamma(u)$ is the Pochhammer symbol, and $a,b,c,d$ are complex numbers.
This is to give an explicit description of the domain of convergence of your second series provided in the answer by Alexandre Eremenko.
In the $(s,t)$-plane, the domain is the interior of the set \begin{equation} \{(s,t)\in\mathbb R^2\colon s<g(t)\}, \end{equation} where \begin{equation} g(t):=\inf_{a\in(0,1)}h(a,t) \end{equation} and \begin{equation} h(a,t):=\frac{(1-a) \ln (1-a)+(1+a) \ln (1+a)-(1-a) t}a. \end{equation} The partial derivative of $h(a,t)$ in $a$ is \begin{equation} \frac{t-\ln(1-a^2)}{a^2}. \end{equation} So, if $t>0$, then $h(a,t)$ is increasing in $a\in(0,1)$ from $h(0+,t)=-\infty$ and hence $g(t)=-\infty$. If $t<0$, then $h(a,t)$ attains its minimum in $a\in(0,1)$ when $a$ is the root of the equation $t-\ln(1-a^2)=0$, that is, when $a=\sqrt{1-e^t}$. Thus, the domain of convergence for your second series is \begin{equation} \{(s,t)\in\mathbb R^2\colon t<0\ \&\ s<h(\sqrt{1-e^t},t)\}. \end{equation}
Part of this region (for $t>-8$) is shown here:
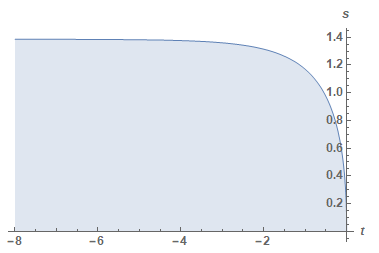
Note that $h(\sqrt{1-e^t},t)\to\ln4=1.386\dots$ as $t\to-\infty$.
The region of convergence is the union of polydisks $\{(x,y):|x|<r_1,|y|<r_2\}$ such that $$\limsup_{m+n\to\infty}|c_{m,n}r_1^nr_2^m|^{1/(m+n)}\leq 1,$$ which is the generalization of the Cauchy-Hadamard formula. Computation for your second series leads to the following description of this region. In the plane $(s,t)=(\log r_1,\log r_2)$ the region is the intersection of half-planes $H_\alpha,\; 0\leq\alpha\leq 1$, where $$H_\alpha=\{(s,t):\alpha s+(1-\alpha)t<(1+\alpha)\log(1+\alpha)+(1-\alpha)\log(1-\alpha)\}.$$ I do not see how to describe this intersection more explicitly. One can do a similar computation for the first series.
The reference for the Cauchy-Hadamard formula is B. A. Fuks, Theory of analytic functions of several complex variables, AMS, Providence, R.I. 1963, Ch. I, Sect. 3, Theorem 3.7.
