Let $D$ be a nonempty compact convex plane region whose boundary is a smooth curve whose radius of curvature is at most 1 everywhere. Can the boundary of $D$ intersect a circle of radius 1 in more than two points?
-
1$\begingroup$ Some related questions: Do you have an example of a curve with radius of curvature $<1$ and diameter $>2$? Do you have an example of a curve with radius of curvature $<1$ and width $>2$? I've been playing with curves of constant width, and also with curves in polar form $r(\theta) = r_0 + \sum_{j=1}^3 a_j \cos(j \theta)$ with $r_0$ a little less than $1$ and $a_j$ small, and I can't seem to achieve any of these. $\endgroup$– David E SpeyerCommented Feb 11, 2021 at 16:09
-
2$\begingroup$ PS Really nice question! $\endgroup$– David E SpeyerCommented Feb 11, 2021 at 16:10
-
1$\begingroup$ I initially somehow missed "radius of" in front of "curvature". Resulting question seemed to be also interesting. $\endgroup$– მამუკა ჯიბლაძეCommented Feb 11, 2021 at 16:34
-
1$\begingroup$ $R=D$, is that right? $\endgroup$– Deane YangCommented Feb 11, 2021 at 20:07
-
4$\begingroup$ The trick, I believe, to solving this is to use the support function of $R$, defined as follows: $$ h(\theta) = \sup_{x \in R} x\cdot(\cos\theta,\sin\theta)$$ You can show that, if $\rho(\theta)$ is the radius of curvature at the point on $\partial R$, where the outer unit normal is $(\cos\theta,\sin\theta)$, then $h$ satisfies $h'' + h = \rho$. At this point, the question reduces to a comparison result between a solution to $h''+h \le 1$ and a solution to $h'' + h = 1$. $\endgroup$– Deane YangCommented Feb 11, 2021 at 20:20
2 Answers
David Speyer writes "the only way this strategy could work is if we improve the $2$ in the previous paragraph to $\pi$." That improvement follows.
Suppose $f < 0$ and $f + f'' \leq 0$ on $(0,c)$, with $f(0)=f(c)=0$. We show $c \geq \pi$, with equality if and only if $f(\cdot)$ is a negative multiple of $\sin(\cdot)$.
Let $\alpha = \tan^{-1}(f'/f)$, so $\alpha(0) = \pi/2$ and $\alpha(c) = -\pi/2$. Note that if $f(x) = A\sin x$ then $\alpha(x) = \pi/2 - x$. In general we compute $$ \alpha' = \frac{f\!f''-{f'}^2}{f^2+{f'}^2}, $$ whence $$ \alpha' + 1 = \frac{f(f+f'')}{f^2+{f'}^2} > 0. $$ Therefore $\alpha' \leq -1$ on $(0,c)$. Since $\alpha$ decreases by $\pi$ on that interval, the interval must have length at least $\pi$. Moreover if $c=\pi$ then $f+f''=0$ on all of $(0,\pi)$, which together with $f(0)=f(\pi)=0$ makes $f$ a multiple of the sine function. $\Box$
-
2$\begingroup$ It seems that this result can also be obtained from the Sturm(-Picone) comparison theorem. $\endgroup$ Commented Feb 12, 2021 at 5:58
-
$\begingroup$ Very nice! At some point, I'll rewrite my answer to remove the digressions, and then we'll have a complete solution. $\endgroup$ Commented Feb 12, 2021 at 13:37
-
1$\begingroup$ Yes. Using Sturm comparison is what I had in mind. $\endgroup$ Commented Feb 12, 2021 at 15:22
-
$\begingroup$ David: Thanks. Maybe keep the "digressions", which could have independent interest, and end with a pointer to a new (possibly CW) answer. $\endgroup$ Commented Feb 12, 2021 at 15:38
-
1$\begingroup$ I don't really think any of the digressions are useful here. I wanted to be able to assume that the center of the circle was in $R$ so that I could parametrize $\partial R$ in polar coordinates; that didn't turn out to matter. I proved a bound of $2$ that Noam improved to $\pi$; I don't see that argument as helping. I spent some time realizing that there wasn't going to be a useful bound for how long $\partial R$ spends in the arcs outside the circle; that was very helpful in figuring out where to focus but I don't see why to keep it in the final answer. $\endgroup$ Commented Feb 12, 2021 at 18:00
Here is a proof that the answer is "no", building on Deanne Yang's excellent idea to use the support function. This function $h(\theta)$ is defined as $h(\theta) = \mathrm{sup}_{(x,y) \in R} ((\cos \theta) x + (\sin \theta) y)$.
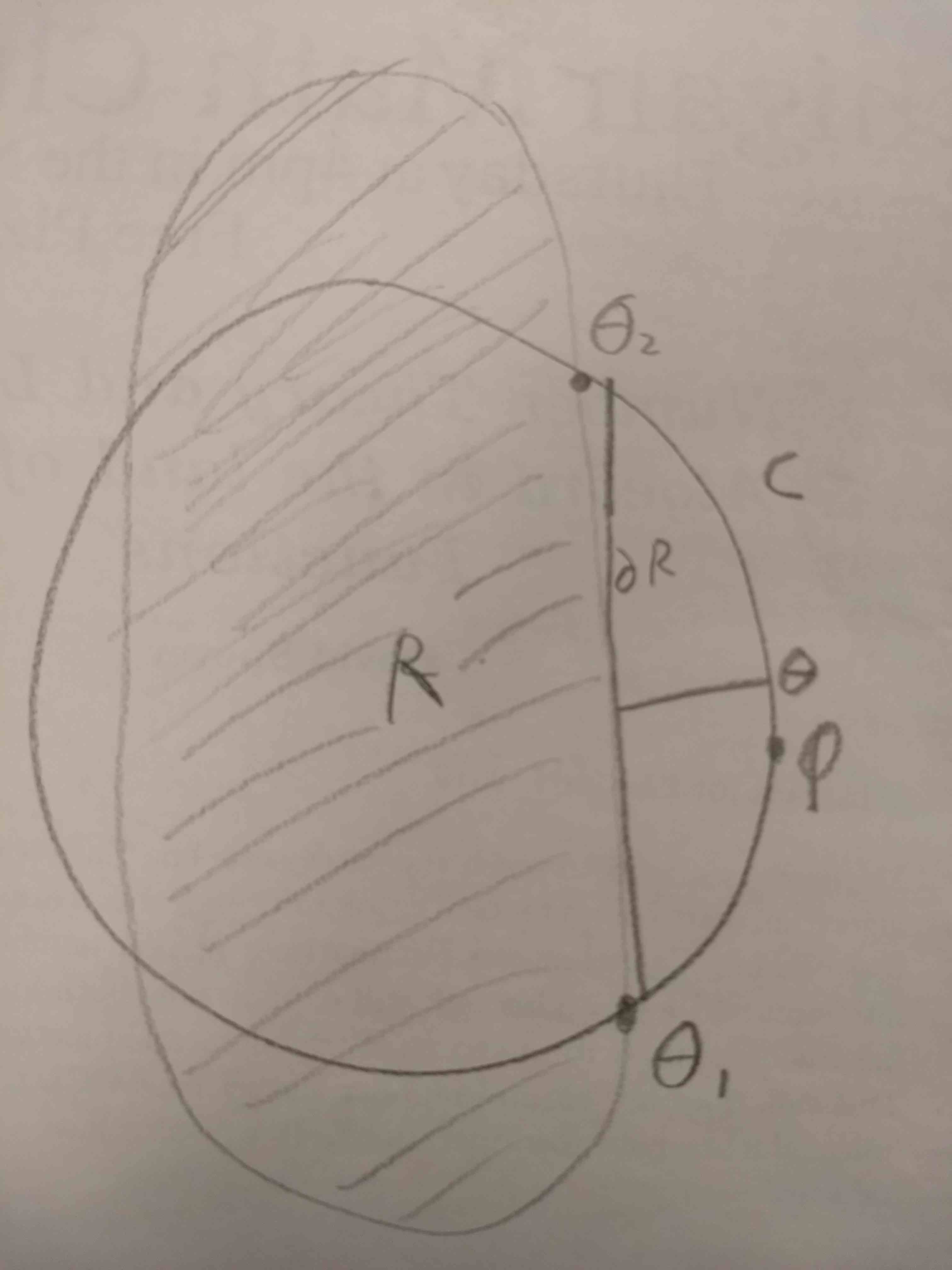
Let our hypothetical unit circle $C$ be centered at the origin. If $\partial R$ met $C$ more than twice, then there would be at least two arcs of $\partial R$ that pass through the interior of $C$, and therefore at least one of these arcs would meet $C$ at points which are less than $\pi$ apart. We will show that this cannot happen. Suppose, to the contrary, that $\partial R$ meets $C$ at angles $\theta_1 < \theta_2 < \theta_1 + \pi$, with $R$ on the other sides of $\partial R$ from the arc between these points. The image below depicts the hypothetical positions of $R$, $C$, $\theta_1$ and $\theta_2$:
Any curve with a flex has radius of curvature $\infty$ at the flex, so $\partial R$ has no flexes and thus $R$ is convex. (This follows from a theorem of Tietze that I learned about here.)
Choose $\phi$ in the interval $(\theta_1, \theta_2)$. Since $R$ is convex, some supporting hyperplane $(\cos \theta) x + (\sin \theta) y = h(\theta)$ separates $R$ from $(\cos \phi, \sin \phi)$. As shown in the image above, $\theta$ is the midpoint of the arc subtended by this separating hyperplane, and so $\theta \in (\theta_1, \theta_2)$. We deduce that, for this $\theta$, we have $h(\theta) < (\cos \theta) (\cos \phi) + (\sin \theta) (\sin \phi) = \cos (\theta - \phi) \leq 1$.
On the other hand, $h(\theta_1) \geq (\cos \theta_1) (\cos \theta_1) + (\sin \theta_1) (\sin \theta_2) = 1$, and similarly for $\theta_2$. So $h>1$ at the endpoints of $(\theta_1, \theta_2)$ and drops to $<1$ somewhere in the interior.
Deanne tells us that $h+h''$ is the radius of curvature, so we have $h+h'' < 1$. Putting $f=h-1$, we have the following situation: $f+f''<1$, $f(\theta_1)>0$, $f(\theta)<0$ and $f(\theta_2)>0$, and $\theta_1 < \theta < \theta_2 < \theta_1+\pi$.
Noam Elkies's answer shows that this is impossible.
-
$\begingroup$ I think your proof of Propp's question also solves my problem as stated for 2D: math.stackexchange.com/questions/4014421/… or mathoverflow.net/questions/383659/… . The question for higher than 2D is still out but if you write this up there I will accept it. $\endgroup$ Commented Feb 14, 2021 at 19:52