Here are some ideasis a proof that the answer is "no", building on Deanne Yang's excellent idea to use the support function. This function $h(\theta)$ is defined as $h(\theta) = \mathrm{sup}_{(x,y) \in R} ((\cos \theta) x + (\sin \theta) y)$.
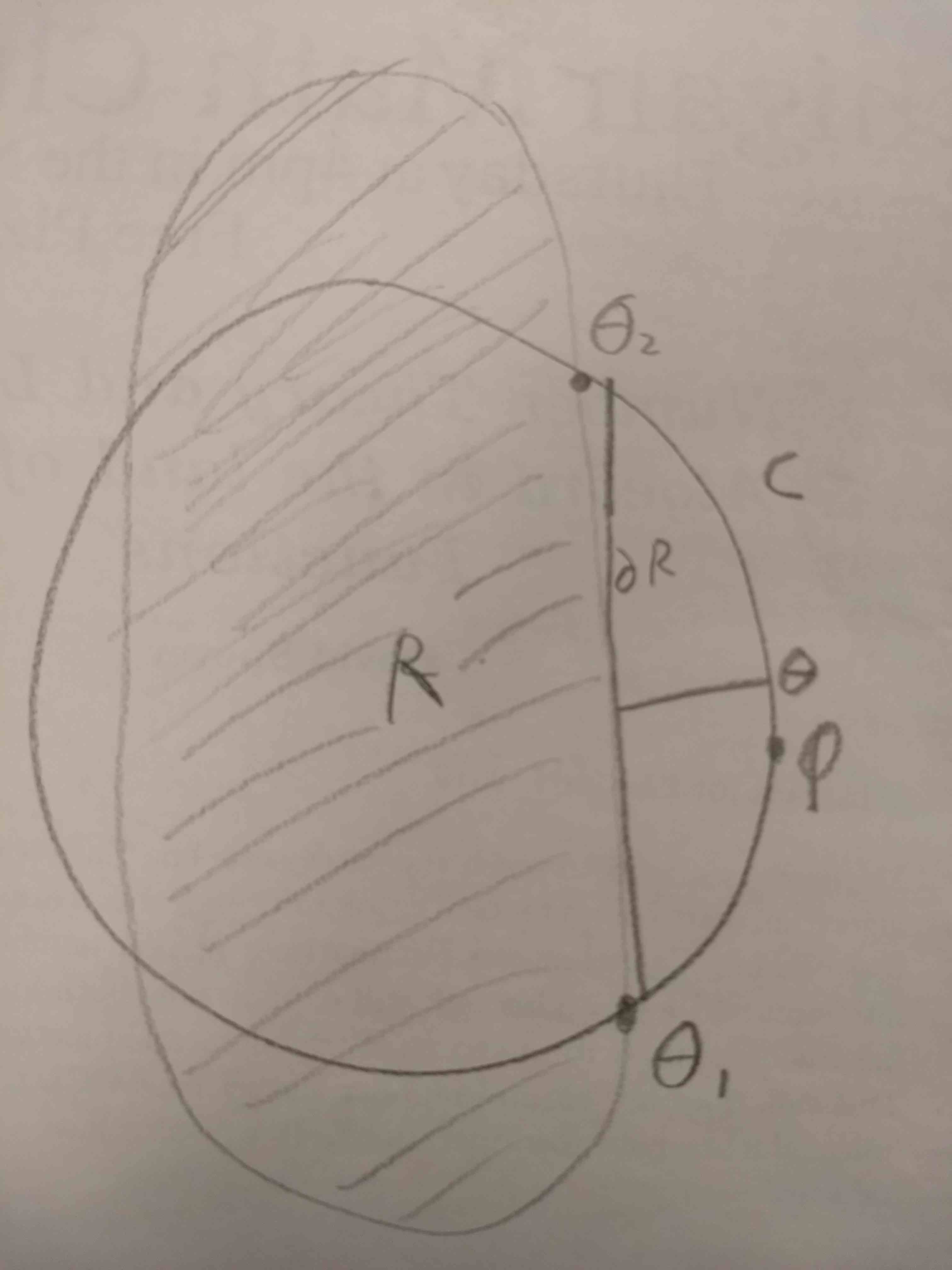
Let our hypothetical unit circle $C$ be centered at the origin. I'll also imagineIf $\partial R$ met $C$ more than twice, then there would be at least two arcs of $\partial R$ that pass through the origin is ininterior of $R$$C$, maybe someone elseand therefore at least one of these arcs would meet $C$ at points which are less than $\pi$ apart. We will see how to removeshow that this cannot happen. Suppose, to the contrary, that $\partial R$ meets $C$ at angles $\theta_1 < \theta_2 < \theta_1 + \pi$, with $R$ on the other sides of $\partial R$ from the arc between these points. The image below depicts the hypothetical positions of $R$, $C$, $\theta_1$ and $\theta_2$:
Any curve with a flex has radius of curvature $\infty$ at the flex, so $\partial R$ has no flexes and thus $R$ is convex. (This follows from a theorem of Tietze that I learned about here.) Thus, any ray from the origin meets $\partial R$ at a unique point $r(\theta) (\cos \theta, \sin \theta)$. Letting $h$ be the sucpport function Deanne introduces, note that we have $h(\theta) \geq r(\theta)$.
Let's suppose we have $2m$ points $\theta_1$, $\theta_2$, ..., $\theta_{2m}$, such that $r(\theta)$ is $>1$ on $(\theta_{2j-1}, \theta_{2j})$ and $<1$ on $(\theta_{2j},\theta_{2j+1})$. Since $h \geq r$, we also have $h \geq 1$ onChoose $(\theta_{2j-1}, \theta_{2j})$. On$\phi$ in the other hand, chooseinterval $\phi \in (\theta_{2j},\theta_{2j+1})$$(\theta_1, \theta_2)$. Then, sinceSince $R$ is convex, there must be some $\theta$ such that the supporting hyperplane $(\cos \theta) x + (\sin \theta) y = h(\theta)$ separates $(\cos \phi, \sin \phi)$$R$ from $R$$(\cos \phi, \sin \phi)$. For this $\theta$As shown in the image above, we must have $h(\theta) < (\cos \theta)(\cos \phi) + (\sin \theta) (\sin \phi) = \cos (\theta-\phi) \leq 1$$\theta$ is the midpoint of the arc subtended by this separating hyperplane, and drawing a picture shows that we must haveso $\theta \in (\theta_{2j}, \theta_{2j+1})$$\theta \in (\theta_1, \theta_2)$. SoWe deduce that, in eachfor this $(\theta_{2j}, \theta_{2j+1})$$\theta$, there is some place where $h$ iswe have $<1$$h(\theta) < (\cos \theta) (\cos \phi) + (\sin \theta) (\sin \phi) = \cos (\theta - \phi) \leq 1$.
We are thus reduced toOn the question: If $h$ is periodic modulo $2 \pi$ and $h+h'' < 1$other hand, can $h$ cross the value$h(\theta_1) \geq (\cos \theta_1) (\cos \theta_1) + (\sin \theta_1) (\sin \theta_2) = 1$, and similarly for $1$ more than twice in a period? One may as well put$\theta_2$. So $f = h-1$, so that$h>1$ at the equation isendpoints of $f+f''<0$,$(\theta_1, \theta_2)$ and ask about zeroes ofdrops to $f$ instead$<1$ somewhere in the interior.
One idea I had about how to approach this was to showDeanne tells us that, if $f+f''<0$ then two zeroes of $f$ couldn't be that close together. If $f$$h+h''$ is negative between the zeroes, then I can show that they are at least $2$ apart. Proof: Suppose for the sakeradius of contradiction that $f(0) = f(a) = 0$ for $0<a<2$ with $f+f''<0$ and $f$ negative on $(0,a)$. Let $f$ be minimized at $b$ and, WLOGcurvature, rescale $f$ so thatwe have $f(b) = -1$$h+h'' < 1$. By the mean value theorem, there are $0 < c < b < d < 0$ with $f'(c) = -1/b$ andPutting $f'(d) = 1/(a-b)$ so$f=h-1$, bywe have the mean value theorem again, there is $e \in (b,d)$ with $f''(e) = \tfrac{1/(a-b) + 1/b}{d-c} = \tfrac{a}{b(a-b)(d-c)} \geq \tfrac{a}{(a/2)^2 a} = 4/a^2$. But, also, $f(e) \geq -1$. So $4/a^2-1 < 0$ and $a \geq 2$.following situation: $\square$
Unfortunately$f+f''<1$, there is no comparable bound when $f$ is positive. For $m>1$$f(\theta_1)>0$, the function $\sin (mx)$ obeys $f+f''<0$$f(\theta)<0$ and $f>0$ on $(0,\pi/m)$$f(\theta_2)>0$, and we can make $\pi/m$ as small as we wish$\theta_1 < \theta < \theta_2 < \theta_1+\pi$. This means
Noam Elkies's answer shows that the only way this strategy could work is if we improve the $2$ in the previous paragraph to $\pi$impossible.