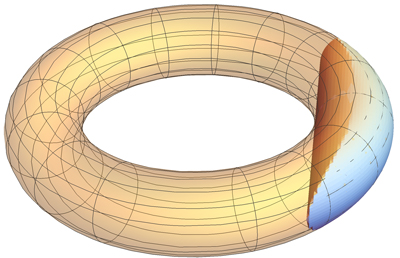
I want to say some for the question 2 :
(1) Construction of tubular surface of curve of const curvature $1$ :
If $e_i$ is a canonical basis in $\mathbb{R}^3$, then we define
\begin{align*} c(t)&
=c_0(t) + \frac{3}{2}e_3,\\
c_0&=\bigg(\cos (-t),0,\sin(-t) \bigg) \\
&\\
N(t)&=-c_0(t),\ b= (0,1,0)\\ \end{align*}
Hence $T=c'(t),\ N, \ b$ is Frenet-Serre frame so that we define
\begin{align*}
X(t,\theta )&= c_0(t) +
\frac{1}{2} (-c_0\cos\ \theta +b\sin\ \theta ) +
\frac{3}{2}e_3\\&=\bigg( \cos\ t\cdot (1-\frac{1}{2}\cos\ \theta ) ,
\frac{1}{2}\sin\ \theta,-\sin\ t\cdot (1-\frac{1}{2}\cos\ \theta )
\bigg)
+ \frac{3}{2} e_3\end{align*}
(2) Notation and Definition : If $X$ parametrize a surface
$\Sigma$, then we define
$$ \Sigma_{right}=\Sigma\cap \{y\geq 0 \} ,\
\Sigma_{left} = \Sigma\cap \{y\leq 0\},\ \Sigma_0=\Sigma\cap \{
z<\frac{1}{2}\} $$ and a line $l:=\{ (t,0,\frac{1}{2} )|t\in
\mathbb{R} \}$
(3) If $S_{rev}$ is a revolution surface of the curve $
X(t,\pi)$ along the line, then $\Sigma_0$ is a graph surface and
then we can show that $S_{rev}$ is contained in
$\Sigma$, by a direct computation.
(4) If $S$ is a sliding which can be obtained from the
intersection between $\Sigma$ and cylinder of radius $\frac{1}{2}$,
and if $S$ contains a circle $X(\frac{\pi}{2},\theta)$, then we
rotate $S$ along this circle. That is, $(0,0,1)$ goes through
$\Sigma_{right}$. If we define $S_{right},\ S_{left}$ wrt $y>0,\
y<0$, and if we do $\pi$-rotation, then $S_{right}$ can be cut as
much as possible, but $S_{left}$ not. Hence the resulting $S$ is
larger than $S_{rev}$.
(5) If $\alpha$ is a union of piecewise smooth space
curves $\alpha_i$ whose curvatures are less than $1$, and if
$\alpha$ has a continuous
unit tangent vector, then the resulting sliding can be $S_{rev}$
Proof : If $N_i^s,\ N_i^e$ are normal vectors at starting, end
points of $\alpha_i$, then we define $\theta_{i(i+1)}=\angle
(N_i^e,N_{i+1}^s)$.
When a sliding in tubular surface related to $\alpha_i$ goes to
tubular surface related to $\alpha_{i+1}$, if the sliding is cut
through $\theta_{i(i+1)}$-rotation, then the sliding goes back and
forth freely on two surfaces without further volume change.
Assume that $\theta_{12}=\pi$ and $N_i^s,\ N_i^e$ are orthogonal to
a fixed unit vector for all $i$. Hence we map $N_i^s,\ N_i^e$ into
$N_i$ in unit circle s.t. distance between $N_i$ and $ N_{i+1}$ is
$\theta_{i(i+1)}$.
If sliding is in second tubular surface, then $N_1$ goes to $N_2$ by
using half of the circle. Hence if we take $N_3$ in the not used
part of the circle, then $\pi$-rotation is not sufficient, which
complete the proof.