How to show continuity and monotonicity of solutions to this parametrized equation?

Question

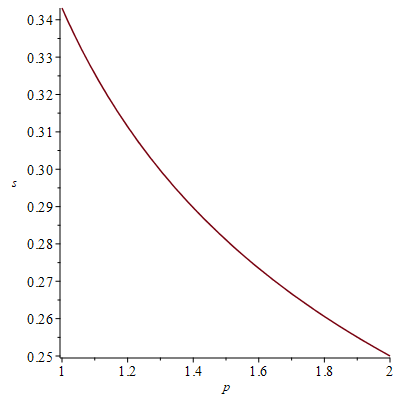
Let $1 \le p <2$ be a parameter. Consider the equation

$$ \frac{2^{p/2} (1-\sqrt{s})^p-1}{\sqrt{s}}=-2^{p/2-1}p(1-\sqrt{s})^{p-1}. \tag{1} $$

I am rather certain that for each $1 \le p <2$, there is unique solution $s=s(p)$ in $(\frac{1}{4},1]$.

Question: Is $ p\to s(p)$ monotonically decreasing in $p$? Is it continuous in $p$? How can I prove this rigorously?

Mathematica doesn't give a closed-form formula for $s(p)$.

Motivation:

This question comes from trying to find a "point of contact" when a certain chord between $(0,H(0)), (s,H(s))$ coincides with the tangent to $H$ at $s$, where $H:=F^q$ and $$ F(s) := \begin{cases} 2(\sqrt{s}-1)^2, & \text{ if }\, s \ge \frac{1}{4} \\ 1-2s, & \text{ if }\, s \le \frac{1}{4} \end{cases} $$

The equation $\frac{H(s)-H(0)}{s-0}=H'(s)$ is nothing but equation $(1)$ above.

---

One idea is to assume that $s(p)$ is differentiable, and differentiate equation $(1)$ w.r.t $p$. Doing that, one gets the following (details here)

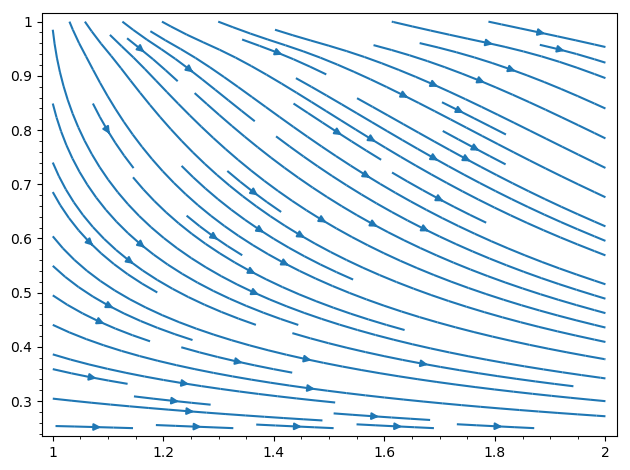
This is a stream line plot of $s(p)$: the function must follow one of these lines, depending on its initial condition. The horizontal-axis is the $p$-variable and the vertical-axis is $s$-variable. $s(p)$ seems monotonically decreasing on the interval, as required. This seems to suggest that there is a unique solution for every initial condition.

---

Analysis of $p=1,2$:

Let's prove that $s(2)=\frac{1}{4},s(1)=(2-\sqrt 2)^2 \simeq 0.343$.

For $p=1$ the equation reduces to $ \sqrt 2(1-\sqrt s)-1=-\frac{\sqrt s}{\sqrt 2}$. Setting $x=\sqrt s$, we obtain $ 1-\sqrt 2=x(1/\sqrt 2-\sqrt 2) \Rightarrow x=2-\sqrt 2.$

For $p=2$ the equation reduces to

$2(1-\sqrt s)^2-1=-2(1-\sqrt s)\sqrt s \Rightarrow -1=-2(1-\sqrt s)\big((1-\sqrt s)+\sqrt s \big) \Rightarrow 2(1-\sqrt s)=1 \Rightarrow s=\frac{1}{4}.$

---

Answer 1

With $r:=p/2\in[1/2,1)$ and $y:=1-\sqrt s\in[0,1/2)$, rewrite your equation (1) as $$G(r,y):=2^r y^{2 r-1} (r+y-r y)-1=0. \tag{2}$$ For any $r\in(1/2,1)$, $G(r,0)=-1\ne0$, so that $y=0$ is not a solution of equation (2). Also, $y^{2 r-1}$ is undefined for $r=1/2$ and $y=0$. So, in what follows let us assume $r\in(1/2,1)$ and $y\in(0,1/2)$ by default.

Clearly, $G(r,y)$ is strictly and continuously increasing in $y$ from $G(r,0+)=-1<0$ to $G(r,\frac12-)=2^{-r} (1+r - 2^r)>0$, for each $r$; here one may use the concavity of $1+r - 2^r$ in $r$. So, for each $r$, equation (2) has a unique root $$Y:=Y(r)\in(0,1/2). \tag{3}$$ Moreover, $$G'_y(r,y)=2^{r+1} r y^{2 r-2}(r-1/2 + (1-r)y)>0.$$ So, by the implicit function theorem, the function $Y$ is differentiable (and hence continuous). Moreover, $$Y'(r)=-\frac{G'_r(r,y)}{G'_y(r,y)}\Big|_{y=Y} \overset{\text{sign}}=H(r,Y)>H(1/2,Y)\overset{\text{sign}}=h(Y), $$ where $a\overset{\text{sign}}=b$ means $\text{sign}\, a=\text{sign}\,b$, $H(r,y):=-1 + y - (y + r (1 - y)) \ln(2 y^2)$, and $$h(y):= -\frac{1 - y}{1 + y} - \frac12\,\ln(2 y^2).$$ Note that $h(1/2)>0$ and $h'(y)=-\frac{1+y^2}{y (1+y)^2}<0$, whence $h>0$ and hence $Y'>0$.

Thus, $Y(r)$ is continuously increasing in $r$, which means that the root $s$ of your equation (1) is continuously decreasing in $p$, as you conjectured.

Answer 2

Put $t=1-\sqrt{s}\in[0,1/2)$ so the equation writes $$ \Big(1-\frac p2\Big)\, t^p+ \frac p2\, t^{p-1}=2^{-\frac p2}$$

Now if we put $u:=t^{p-1}$ the equation takes the form $$u+\Big( \frac2p -1\Big)\,u^q =\frac {2^{1-\frac p 2 }} p$$ with $q=\frac p{p-1} >1$, that can be solved by series (see e.g. here) (this way one covers an interval $1.57<p\le2$ if I'm not wrong. To cover the other values of $p$, close to $1$, one needs to put the equation in other forms).

Answer 3

This can be done with Maple and Mathematica as follows. First, let us look at the plot done with Maple

plots:-implicitplot((2^(p/2)*(1 - sqrt(s))^p - 1)/sqrt(s) =
 -2^(p/2 - 1)*p*(1 - sqrt(s))^(p - 1), p = 1 .. 2, s = 1/4 .. 1);

The result suggests that $s(p)$ changes from approximately $0.34$ to approximately $0.25$ as $p$ runs from $1$ to $2$. More exactly, making use of Mathematica, we have

NMaximize[{s, (2^(p/2)*(1 - Sqrt[s])^p - 1)/Sqrt[s] == -2^(p/2 - 1)*
 p*(1 - Sqrt[s])^(p - 1) && p >= 1 && p <= 2}, {p, s}]

$\{0.343146,\{p\to 1.,s\to 0.343146\}\}$ and

NMinimize[{s, (2^(p/2)*(1 - Sqrt[s])^p - 1)/Sqrt[s] == -2^(p/2 - 1)*
 p*(1 - Sqrt[s])^(p - 1) && p >= 1 && p <= 2}, {p, s}]

$\{0.25, \{p -> 2., s -> 0.25\}\}$

We can find the exact values by

solve(eval((2^(p/2)*(1 - sqrt(s))^p - 1)/sqrt(s) = -2^(p/2 - 1)*p*(1 - sqrt(s))^(p - 1), p = 1), s);

$-4\,\sqrt {2}+6$

and

solve(eval((2^(p/2)*(1 - sqrt(s))^p - 1)/sqrt(s) = -2^(p/2 - 1)*p*(1 - sqrt(s))^(p - 1), p = 2), s);

$\frac 1 4$

Now we find the implicit derivative of $s$ with respect to $s$ by

a := implicitdiff((2^(p/2)*(1 - sqrt(s))^p - 1)/sqrt(s) = -2^(p/2 - 1)*p*(1 - sqrt(s))^(p - 1), s, p):

and its maximum value when $p$ runs from $1$ to $2$ by

DirectSearch:-GlobalOptima(a, {(2^(p/2)*(1 - sqrt(s))^p - 1)/sqrt(s) =
 -2^(p/2 - 1)*p*(1-sqrt(s))^(p - 1), 1<= p, p<=2, s<=-4*sqrt(2) + 6, 1/4 <= s}, maximize);

$[-0.0482867952575873, [p = 1.99999990682054, s = 0.250000000105689], 358]$ Because the default absolute error of the GlobalOptima command equals $10^{-6}$, this does the job.