Two versions of Sylvester identity

Question

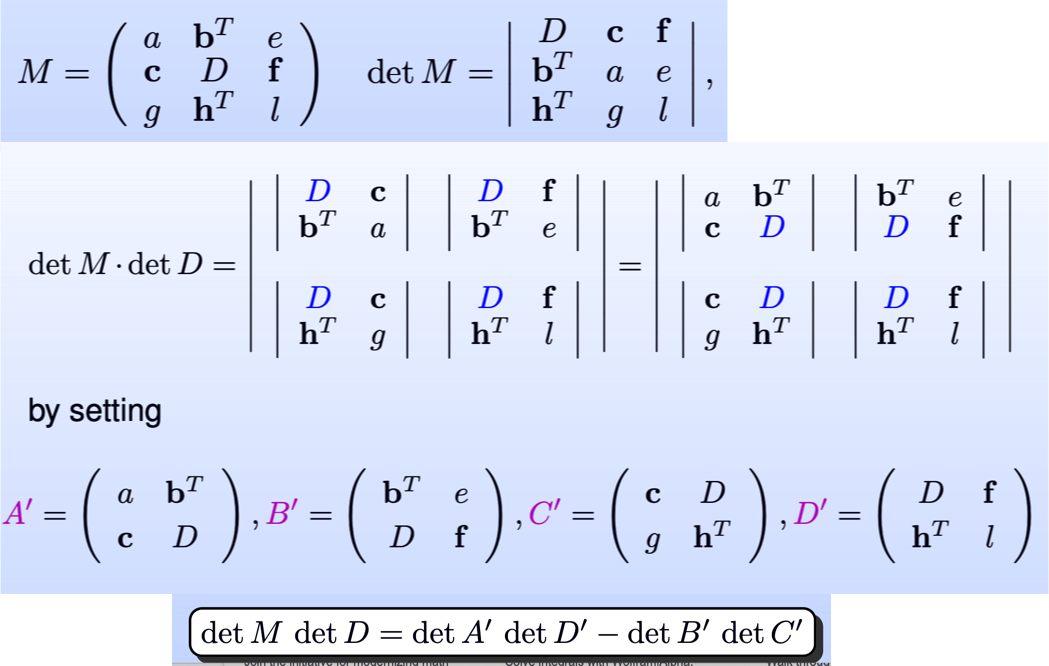
MathWorld presents the following two versions of Sylvester's determinant identity, relating to an $n\times n$ matrix $\mathbb{A}$:

First: $$ |\mathbb{A}||A_{r\,s,p\,q}| = |A_{r,p}||A_{s,q}| - |A_{r,q}| |A_{s,p}| $$

where $r$ and $s$ ($p$ and $q$) are sets that indicate which rows (columns) of $\mathbb{A}$ are to be deleted (correcting MathWorld's typo).

Second:
$$ |\mathbb{A}|\left[ a_{k\,k}^{(k-1)}\right]^{n-k-1} = \left| \begin{matrix} a_{k+1\, k+1}^{(k)} & \cdots & a_{k+1\, n}^{(k)} \\ \vdots & \ddots & \vdots \\ a_{n\, k+1}^{(k)} & \cdots & a_{n\, n}^{(k)} \\ \end{matrix}\right| $$

where

$$ a_{i\, j}^{(k)} = \left| \begin{matrix} a_{11} & \cdots & a_{1\,k} & a_{1 \, j} \\ \vdots & \ddots & \vdots& \vdots \\ a_{k\ 1} & \cdots & a_{k\,k}& a_{k\, j} \\ a_{i\ 1} & \cdots & a_{i\, k} & a_{i\, j} \\ \end{matrix} \right| $$

for $k<i$, $j \leq n$.

Would anyone help me prove that these two versions are indeed equivalent.

Note: As has been pointed our below, MathWorld's claim is obviously (using a counterexample) incorrect; the second version implies only a special case (when r,s,p,q are single numbers) of the first 'version'.

Answer 1

For the special case that $r,s,p,q$ are single elements, it is shown in these notes (page 7) how the first identity (known as the Desnanot-Jacobi identity) follows from the second identity.

Apply the second identity to the matrix

we thus arrive at the first identity, illustrated graphically as

_source

---

Update: Since I could not find the first identity in the literature, for the more general case when $r,s,p,q$ each contain more than a single element, I tried to check it for an example. I took $n=6$, $r=1,2$, $s=5,6$, $p=1,2$, $q=5,6$. For the $6\times 6$ matrix $A$ I took _{$$A=\left( \begin{array}{cccccc} 0 & 1 & 0 & 0 & 1 & 1 \\ 0 & 0 & 1 & 0 & 1 & 0 \\ 2 & 0 & 2 & 2 & 0 & 0 \\ 2 & 1 & 0 & 0 & 2 & 1 \\ 2 & 0 & 2 & 1 & 0 & 2 \\ 1 & 0 & 0 & 0 & 2 & 2 \\ \end{array} \right)$$} The left-hand-side of the first identity is 0,
_{$\det A \det \left( \begin{array}{cc} 2 & 2 \\ 0 & 0 \\ \end{array} \right) = 24\cdot 0 = 0$,}
but for the right-hand-side I find a nonzero answer:

_{$$ \det \left( \begin{array}{cccc} 0 & 1 & 0 & 0 \\ 0 & 0 & 1 & 0 \\ 2 & 0 & 2 & 2 \\ 2 & 1 & 0 & 0 \\ \end{array} \right) \det \left( \begin{array}{cccc} 2 & 2 & 0 & 0 \\ 0 & 0 & 2 & 1 \\ 2 & 1 & 0 & 2 \\ 0 & 0 & 2 & 2 \\ \end{array} \right) - \det \left( \begin{array}{cccc} 2 & 0 & 2 & 2 \\ 2 & 1 & 0 & 0 \\ 2 & 0 & 2 & 1 \\ 1 & 0 & 0 & 0 \\ \end{array} \right) \det \left( \begin{array}{cccc} 0 & 0 & 1 & 1 \\ 1 & 0 & 1 & 0 \\ 2 & 2 & 0 & 0 \\ 0 & 0 & 2 & 1 \\ \end{array} \right)=$$ $$\qquad\qquad= (-4) \cdot 4 - (-2)\cdot (-2)=-20\neq 0$$}

Incidentally, I did find a determinantal identity of a somewhat similar form in Tao's blog (last equation, Karlin's identity). But it is not quite of the form of the first identity in the OP.

So unless I have made a mistake, my conclusion is that the first identity in the OP only holds when $r,s,p,q$ are single elements, but not more generally.