For the simplestspecial case that $r,s,p,q$ are single elements, it is shown in these notes (page 7) how the first identity (known as the Desnanot-Jacobi identity) follows from the second identity.
Apply the second identity to the matrix
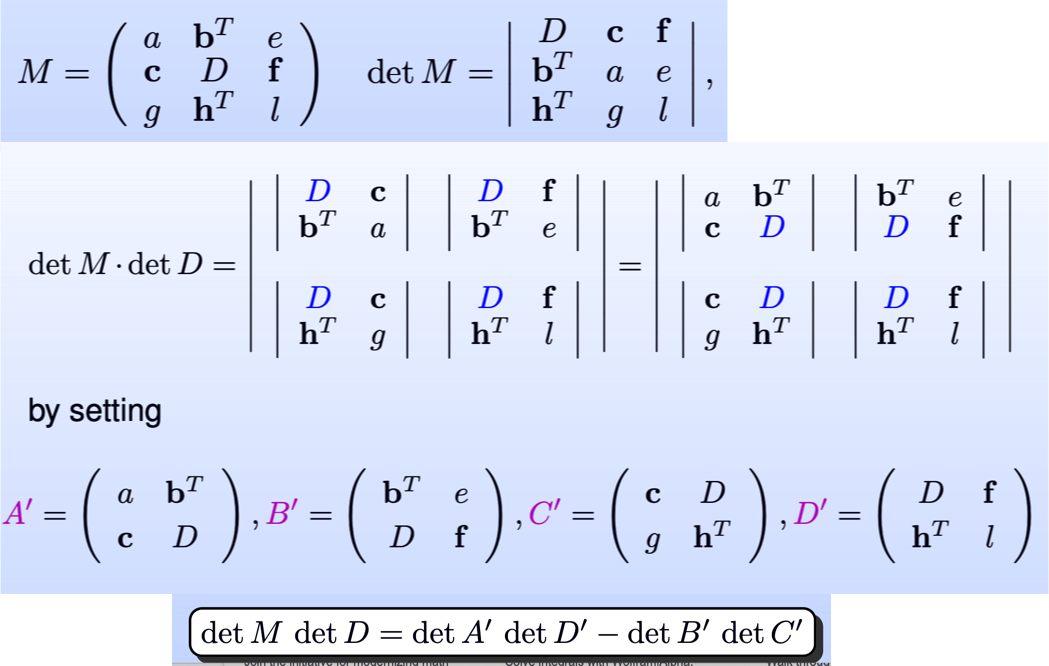
we thus arrive at the first identity, illustrated graphically as
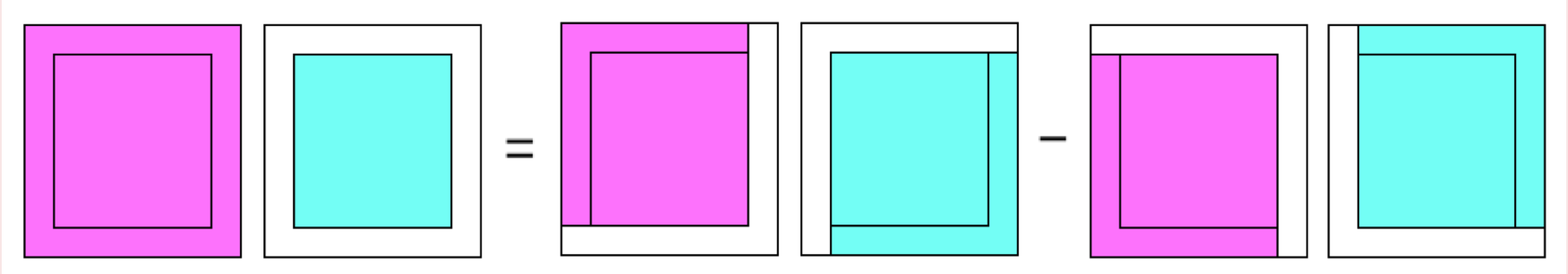
source
Update: Since I could not find the first identity in the literature, for the more general case when $r,s,p,q$ each contain more than a single element, I tried to check it for an example. I took $n=6$, $r=1,2$, $s=5,6$, $p=1,2$, $q=5,6$. For the $6\times 6$ matrix $A$ I took
$$A=\left(
\begin{array}{cccccc}
0 & 1 & 0 & 0 & 1 & 1 \\
0 & 0 & 1 & 0 & 1 & 0 \\
2 & 0 & 2 & 2 & 0 & 0 \\
2 & 1 & 0 & 0 & 2 & 1 \\
2 & 0 & 2 & 1 & 0 & 2 \\
1 & 0 & 0 & 0 & 2 & 2 \\
\end{array}
\right)$$
The left-hand-side of the first identity is 0, but
$\det A \det \left(
\begin{array}{cc}
2 & 2 \\
0 & 0 \\
\end{array}
\right) = 24\cdot 0 = 0$,
but for the right-hand-side I find a nonzero answer:
Incidentally, I did find a determinantal identity of a somewhat similar form in Tao's blog (last equation, Karlin's identity). But it is not quite of the form of the first identity in the OP.
So unless I have made a mistake, my conclusion is that the first identity in the OP only holds when $r,s,p,q$ are single elements, but not more generally.
