6
$\begingroup$

When does an $a\times b$ rectangle fit inside an $x\times y$ rectangle? I have an algebraic condition which I can diagram geometrically, and I'd like a good geometric argument.

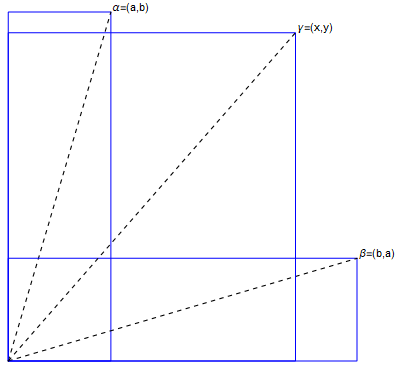
Assume $0<a<b$, $0<x<y$. Then the one rectangle fits in the other iff $a\le x$ and either: $$b\le y$$ or: $$(b^2-a^2)^2 \le (ax-by)^2+(ay-bx)^2$$ which I found algebraically, with help from Mathematica. One possible diagram is

enter image description here

and then the last condition is equivalent to $$ |\boldsymbol{\alpha \times \beta}|^2 \le |\boldsymbol{\alpha \times \gamma}|^2 + |\boldsymbol{\beta \times \gamma}|^2 $$ This can also be interpreted in terms of the lengths of these three vectors and the sines of the angles between them.

Is there a nice geometric argument from this diagram to fitting the one rectangle inside the other?

Improve this question
$\endgroup$
5
  • $\begingroup$ Could you specify the meaning of "fits"? By translation or by translation and rotation? $\endgroup$
    – Alexandre Eremenko
    Jul 15, 2020 at 20:02
  • $\begingroup$ Yes, by translation and rotation. $\endgroup$
    – user44143
    Jul 15, 2020 at 20:09
  • $\begingroup$ Also posted to mathoverflow.net/questions/282158/… $\endgroup$
    – Gerry Myerson
    Jul 16, 2020 at 5:24
  • 1
    $\begingroup$ @GerryMyerson, I don’t think “also posted” is fair — they are related, but that was a partial answer to a request for an algorithm, and this is a question which explicitly links there and asks for a geometric explanation of that answer. $\endgroup$
    – user44143
    Jul 16, 2020 at 9:02
  • $\begingroup$ Sorry, Matt, I missed that you had linked this question to that one. $\endgroup$
    – Gerry Myerson
    Jul 16, 2020 at 9:20

1 Answer 1

Reset to default
7
$\begingroup$

The sense of this inequality is that it turns out into equality when one of rectangles is inscribed in another as on the picture. enter image description here

We get $ax-by=a(m+n)-b(p+q)=am-bp=a^2\cos \varphi-b^2\cos \varphi=(a^2-b^2)\cos \varphi$, analogously $ay-bx=a(p+q)-b(m+n)=aq-bn=(a^2-b^2)\sin \varphi$, and your relation $(a^2-b^2)^2=(ax-by)^2+(ay-bx)^2$ reads as $\cos^2\varphi+\sin^2 \varphi=1$.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Do you how to extend this reasoning to justify the inequality? The difference of the two sides is not obviously monotonic in any of the variables. $\endgroup$
    – user44143
    Jul 19, 2020 at 18:26
  • $\begingroup$ RHS is monotonic in $y$ when $y\geqslant x$ (take the derivative). Does not it help? $\endgroup$
    – Fedor Petrov
    Jul 19, 2020 at 18:40
  • $\begingroup$ Now I see it, I got confused by the 2s and 4s. $\endgroup$
    – user44143
    Jul 19, 2020 at 19:29

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.