I read this as a conjecture in the paper by Ballmann-Brin-Burns, titled "On Surfaces with No Conjugate points" JDG 25(249-273), 1987.
What is current status of this conjecture?
$\begingroup$
$\endgroup$
1
-
1$\begingroup$ see front.math.ucdavis.edu/1309.6539 for a recent partial result. $\endgroup$– Igor BelegradekCommented Oct 29, 2016 at 13:28
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
This is an open question. Actually it is still not known if a compact non-flat surface with non-positive curvature has an ergodic geodesic flow (with respect to the Liouville measure).
$\begingroup$
$\endgroup$
I cannot resist posting their $6$-legged dinosaur(?) Fig.2:
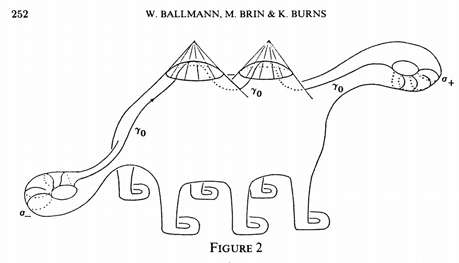
"[W]e arrange that the geodesic $\gamma_0$ passing through the centers of the caps is positively and negatively asymptotic to closed geodesics $\sigma_{+}$ and $\sigma_{-}$ which do not meet the caps (see Figure 2).
answered Oct 11, 2016 at 1:22