3
$\begingroup$

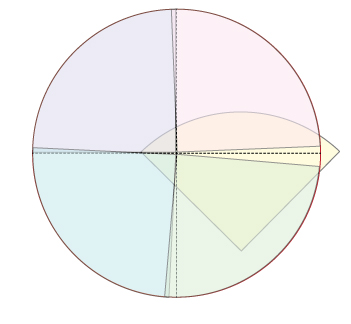
Define an open $k$-sector of a disk as the portion between two radii separated by an angle of $2\pi/k$, but open along the two radii (and closed along the circle boundary). Call a set a sub-$k$-sector if it is a closed set and a subset of an open $k$-sector. So an open $2$-sector is a half-disk minus the diameter, and a sub-$2$-sector is a closed set that fits inside this set.

  SubSectorCovers
          Left: Coverage by three sub-$2$-sectors. Right: Four sub-$4$-sectors. Diameters are dotted lines.
It is clear that one can position three sub-$2$-sectors so that they cover a disk (above, left).

Q1. Is it correct that three sub-semiballs suffice to cover a ball in $\mathbb{R}^3$? What is the generalization to $\mathbb{R}^d$?

By a sub-semiball I mean the natural analog: a closed subset of a half-ball minus its bounding plane.

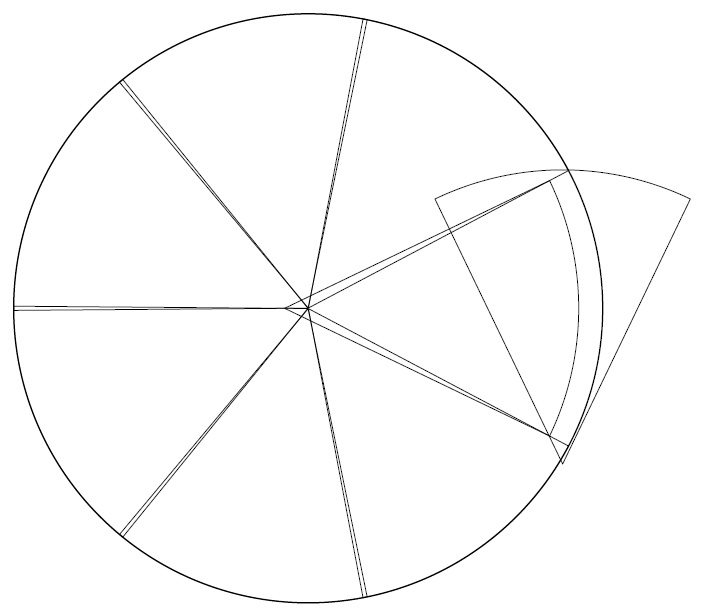
Q2. Does it require six sub-$4$-sectors to cover a disk?

I would like to believe that $k{+}1$ sub-$k$-sectors suffice—-so little of the quadrant is missing!—but the drawing above suggests otherwise.

Update. Here is my attempt to illustrate Douglas Zare's answer to Q2.
          Sub4Sectors

Improve this question
$\endgroup$
4
  • $\begingroup$ How about the center? Perhaps the definitions need minor adjustments? $\endgroup$
    – John B
    Commented Dec 24, 2015 at 14:56
  • $\begingroup$ @JohnB: The center is not part of an open sector, because the two bounding radii are subtracted from the sector. For the half-disk, the entire diameter is subtracted, including the center. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 24, 2015 at 15:00
  • $\begingroup$ Understood, but you say that "It is clear that three sub-2-sectors suffice to cover a disk". However, no sub-2-sector contains the center. $\endgroup$
    – John B
    Commented Dec 24, 2015 at 15:11
  • $\begingroup$ @JohnB: Ah. I meant that one can position three sub-$2$ sectors so that they cover the disk, as in the drawing. I will try to clarify. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 24, 2015 at 15:15

2 Answers 2

Reset to default
3
$\begingroup$

On Q1. The intersection of sub-semiball with the boundary sphere of the ball cannot contain two opposite points. Thus, bu the Borsuk--Ulam(--Lusternik--Shnirelman) theorem it is impossible to cover this sphere by three such closed sets.

Let me now finish the consideration of Q2 started by Douglas. For $k\geq 7$, again $k+1$ sub-$k$-sectors suffice!

Take $k-1$ (not sub!) $k$-sectors centered at the center of the disk with very small overlaps. Taking their appropriate sub-$k$-sectors we cover everything except a very small "star" around the center and, roughly speaking, a $(k-\varepsilon)$-sector of a bit larger radius. Now put the $k$th sub-$k$-sector so as to cover the star and most of the $(k-\varepsilon)$-sector, without some part around its boundary arc. This arc, together with its boundary, can now be covered by the last sub-$k$-sector.

You may see an example for $k=7$ below. I tried to do my best, but it seems that one needs to be very precise in order to cover the central star; still, it's obviously possible.

Eight sub-7-sectors

Improve this answer
$\endgroup$
7
  • $\begingroup$ Edited, so as to address the rest of Q2. $\endgroup$
    – Ilya Bogdanov
    Commented Dec 24, 2015 at 17:26
  • $\begingroup$ Good point about sub-semiball; I took the liberty of adopting your terminology. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 24, 2015 at 18:04
  • $\begingroup$ OK, I've deleted this part of text. $\endgroup$
    – Ilya Bogdanov
    Commented Dec 24, 2015 at 18:12
  • $\begingroup$ After placing the $k-1$ sub-sectors, what remains has angular extent $2\pi/k + \epsilon$. The $k$-th subsector can cover the center star, but will leave a neighborhood of a radius, and a neighborhood of $2\pi/k + \epsilon$ circular arc uncovered. I don't see how this can be covered by one more sub-sector. It seems two are needed...? $\endgroup$
    – Joseph O'Rourke
    Commented Dec 25, 2015 at 14:55
  • $\begingroup$ No, if we shift it towards the center, it will leave just a neighborhood of the arc. OK, I'll try to make a corresponding picture by myself (sorry, perhaps with a worse quality...). $\endgroup$
    – Ilya Bogdanov
    Commented Dec 25, 2015 at 15:25
3
$\begingroup$

I'll address question $2$: Since the distance between $(1,0)$ and $(0,1)$ is $\sqrt{2} \gt 1$, you can cover a neighborhood of a radius with a sub-$4$-sector. The remainder of the disk can be broken into four sub-$4$-sectors by dividing it radially. The same idea lets you cover a disk with four sub-$3$-sectors or six sub-$5$-sectors.

When $k \ge 6$, a sub-$k$-sector has diameter less than the radius of the circle. Any sub-$6$-sector containing the center can't contain a point on the boundary. Any sub-$6$-sector contains less than $2\pi/6$ radians of the boundary, so it takes at least seven to cover the boundary. So, at least eight sub-$6$-sectors are needed to cover the disk.

This does not prove that it requires $k+2$ sub-$k$-sectors to cover a disk for $k \gt 6$, but that many suffices by covering a neighborhood of a radius using two sub-$k$-sectors, then dividing the remainder radially.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .