The answer is yes.
Indeed, let $R$ be the convex hull of the set $\{A,B,C\}$, where $A:=(-1,0)$, $B:=(1,0)$, and $C:=(0,h)$, where $h\in(0,1)$. Let $n=2$. Let $D_1$ and $D_2$ be two closed disks, of respective radii $r_1$ and $r_2$, such that $R\subseteq D_1\cup D_2$.
The intersections $D_1\cap AB$ and $D_2\cap AB$ of $D_1$ and $D_2$ with the segment $AB$ are closed intervals of length $l_1\le2r_1$ and $l_2\le2r_2$ such that $l_1+l_2\ge2$. So, $r_1+r_2\ge l_1/2+l_2/2\ge1$.
Moreover, $r_1+r_2>1$ if at least one of inequalities $l_1\le2r_1$, $l_2\le2r_2$, $l_1+l_2\ge2$ is strict. The inequality $l_1\le2r_1$ will be strict if $D_1\cap AB$ is not a diameter of $D_1$. Similarly, the inequality $l_2\le2r_2$ will be strict if $D_2\cap AB$ is not a diameter of $D_2$. Also, the inequality $l_1+l_2\ge2$ will be strict if $D_1\cap AB$ is a diameter of $D_1$, $D_2\cap AB$ is a diameter of $D_1$, and the interiors of $D_1$ and $D_2$ are not disjoint.
So, $r_1+r_2>1$ unless $D_1\cap AB$ is a diameter of $D_1$, $D_2\cap AB$ is a diameter of $D_1$, and the interiors of $D_1$ and $D_2$ are disjoint. But, if the latter three conditions hold, then $R\not\subseteq D_1\cup D_2$ unless $r_1=0$ or $r_2=0$, as illustrated in the following picture (with $h=1/2$):
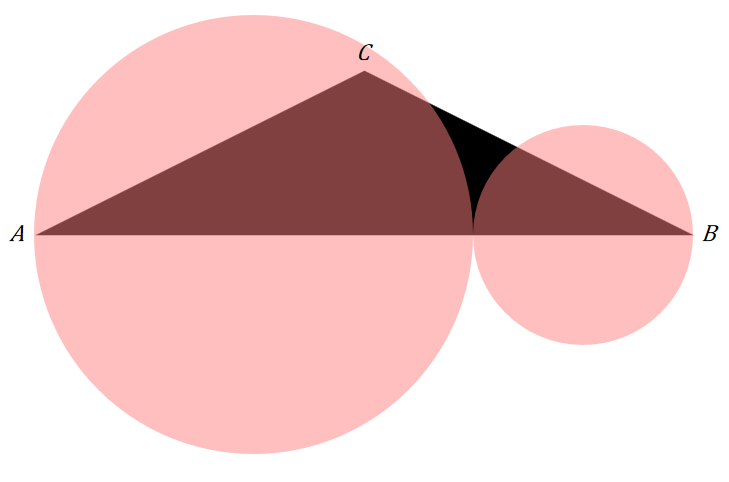
On the other hand, if one of the disks $D_1$ and $D_2$ is of radius $0$ and the other one is of radius $1$ and centered at $(0,0)$, then $R\subseteq D_1\cup D_2$. So, the smallest sum $r_1+r_2$ of the radii of closed disks $D_1$ and $D_2$ covering $R$ is $1$, and hence the smallest sum of the perimeters of closed disks $D_1$ and $D_2$ such that $R\subseteq D_1\cup D_2$ is $2\pi\times1$, and such a sum is attained if only if one of the disks $D_1$ and $D_2$ is of radius $0$ and the other one is of radius $1$ and centered at $(0,0)$.
On the other hand, if $d_1$ and $d_2$ are the closed disks such that the segments $AC$ and $BC$ are diameters of $d_1$ and $d_2$, then $R\subseteq d_1\cup d_2$, as illustrated in the following picture (again with $h=1/2$):
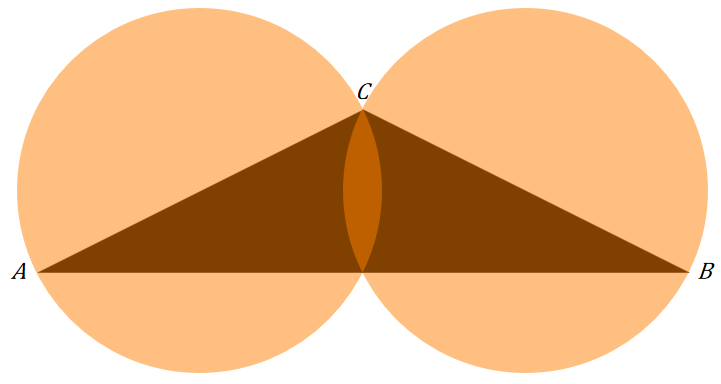
The sum of the areas of these disks $d_1$ and $d_2$ is $2\pi(\frac{\sqrt{1+h^2}}2\,)^2=\pi(1+h^2)/2$, which is strictly less than the sum $\pi\,0^2+\pi\,1^2=\pi$ of the areas of the disks $D_1$ and $D_2$ optimally covering $R$ in terms of the sum of the perimeters.
Thus, the optimal covering of $R$ by two closed disks in terms of the sum of the perimeters is different from any optimal covering of $R$ by two closed disks in terms of the sum of the areas. $\quad\Box$


