Let's assume that we have a simplex $G = \{x\in R^d|\sum_{i=1}^d x_i=1, x_i\ge 0 , i = 1, 2, .., d\}$ and a polyhedral convex subset $H \subseteq G$.
Is it possible to prove that for any extremal point $x^*\in H$, the tangential surface of $x^*$ is a supporting hyperplane of $H$?
In a simple 2D case, it is somewhat trivial. However, for general high dimensional cases, it wasn't..
Thank you in advance. :)
Doesn't seem to be true in higher dimension. I assume that by "supporting hyperplane" you mean that the hyperplane doesn't intersect the interior of $H$.
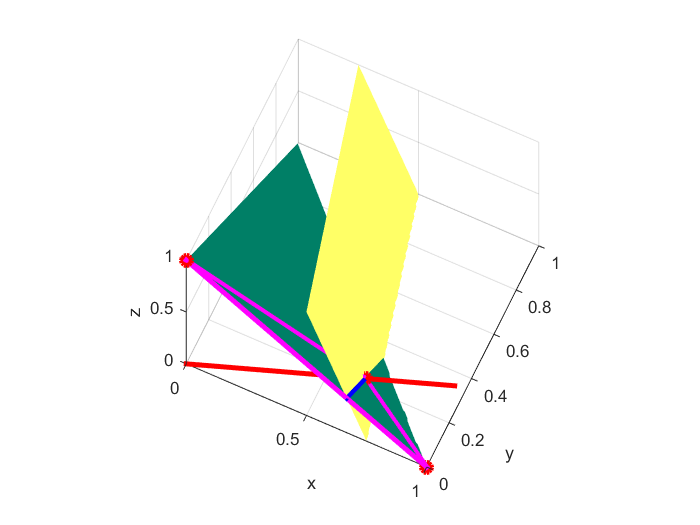
Look at the situation in $\mathbb{R}^3$, so you have a 2-simplex $x+y+z=1$ (a triangle). Take as $H$ the triangle on the simplex with vertices $(1,0,0), (0,0,1), (\frac{2}{3},\frac{1}{6},\frac{1}{6})$ (the third point is the midpoint between the barycenter and the first point). I suggest drawing a picture.
The point $p=(\frac{2}{3},\frac{1}{6},\frac{1}{6})$ is one of the extremal points. The equation of the plane orthogonal to the vector $\langle \frac{2}{3},\frac{1}{6},\frac{1}{6} \rangle$ and that passes through $p$ is $4x+y+z=3$. Its intersection with the simplex is described by $x=\frac{2}{3}$ and $y+z=\frac{1}{3}$, and this intersects $H$ in a segment passing through its interior. For example the point $(\frac{2}{3},0,\frac{1}{3})$ is on this hyperplane and on (the boundary of) $H$.
Indeed, you are right.. The motivation behind this question is posted in 'https://math.stackexchange.com/questions/1563904/tangential-surface-of-an-extreme-point-of-a-convex-subset-of-a-simplex'. Thank you very much.
