The space of polynomials with all real roots

Question

The question stems from an attempt to answer a question of David Speyer. Let $R \subseteq \mathbb{R}^n$ be the space of coefficients of all polynomials of degree $n$ whose all roots are real, i.e.

$R := \lbrace (a_0,\ldots, a_{n-1}): x^n + a_{n-1}x^{n-1} + \cdots + a_0$ has only real roots$\rbrace$

Let $R_+$ be the intersection of $R$ with the all-non-negative orthant. If $R_+$ has more than one connected component, the answer to David's question would be negative.

As I describe below, for $n=3$, $R$ and $R_+$ are connected, and it 'looks' from the plot that David's question has affirmative answer (which, in this special case, can surely be proved in a more straightforward way). However, I would think the spaces $R$ and $R_+$ are interesting in their own right, and would like to know if they have been studied. The answers to even the simplest questions, e.g. if they are connected, seem not very obvious.

Edit: As Richard shows below, $R_+$ is connected. It follows by an even easier version of the same argument that $R$ is connected as well.

Below follows a description of $R$ for the case $n=3$, following the excellent answer to this question.

The computation outlined in the above answer shows that the interior $R^0$ of $R$ is the region of positive definiteness of some symmetric matrix whose entries are polynomials in $a_j$'s. For $n=3$, using the characterization of positive definiteness in terms of minors, it follows that

$R^0 = \lbrace (a_0, a_1, a_2): f_i(a_0, a_1,a_2) > 0,\ 1 \leq i \leq 2\rbrace$

where $f_1 = a_2^2 - 3a_1$, $f_2 = -(27a_0^2 - a_0(18a_1a_2 - 4a_2^2) + 4a_1^3 - a_1^2a_2^2)$. The discriminant of $f_2$ (as a quadratic equation in $a_0$) is $16(a_2^2 -3a_1)^3 = 16f_1^3$ which is positive on $R$. For each $(a_1, a_2)$ such that $f_1(a_1, a_2) > 0$, the admissible $a_0$ values are therefore those belonging to the interval between the minimum $r_m(a_1, a_2)$ and the maximum $r_M(a_1, a_2)$ of the two roots of $f_2(\cdot,a_1,a_2)$. Setting $t := (a_2^2 - 3a_1)^{1/2}$ gives

$r_M(a_1, a_2) = \frac{1}{27}(a_2^3 - 3a_2t^2 + 2t^3) $

$r_m(a_1, a_2) = \frac{1}{27}(a_2^3 - 3a_2t^2 - 2t^3) $

It follows that

$R^0 = \lbrace (a_0, a_1, a_2): a_2^2 - 3a_1 > 0,\ r_m(a_1, a_2) < a_0 < r_M(a_1, a_2) \rbrace$

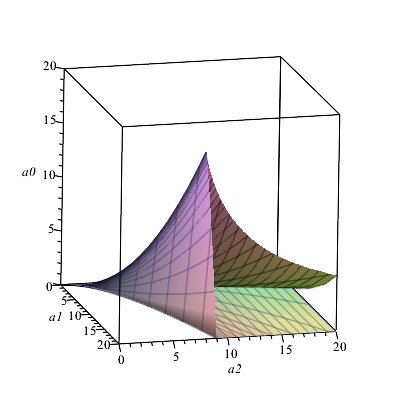
Here is a plot of the boundary of $R$ ($R$ is the region between the two surfaces):

And here is the boundary of $R_+$:

Answer 1

I must be missing something. A monic polynomial with real roots has nonnegative coefficients if and only if all roots are nonpositive. If we have two vectors $u,v\in \mathbb{R}^n$ with nonpositive entries, then there is a continuous path (e.g., a line segment) from $u$ to $v$ that keeps the entries nonnegative. This induces a continuous path between the polynomials with roots $u$ and $v$ that keeps the roots nonpositive.

Answer 2

Space $R$ of hyperbolic(i.e. real-rooted) polynomials of one variable, have actually been studied quite a lot, mostly in papers by V.I. Arnold and his students at the end of 80's-beginning of 90's

I would recommend a book by V.P. Kostov Topics in hyperbolic polynomials of one variable. Societe Mathematique de France, 2011, especially it's chapter 2.

It has a lot of references.

Moreover, Section 2. of a recent preprint http://arxiv.org/abs/1512.08645 has some review on this and some other close questions.

In particular, one can prove that $R$ is homeomorphic to ${\mathbb R}\times{\mathbb R_+^{n-1}}.$

This follows from the fact that the correspondence between roots and coefficients of polynomials is morphism of symmetric product of topological spaces (i.e. quotient by $S_n$ acting by permutations of roots -- see http://www.ams.org/journals/tran/1954-077-03/S0002-9947-1954-0065924-2/S0002-9947-1954-0065924-2.pdf P.538 or http://link.springer.com/article/10.1007%2FBF01094483) and $n$-th symmetric product of real line is exactly ${\mathbb R}\times{\mathbb R_+^{n-1}}$ (see e.g. Proposition 3 there: https://ncatlab.org/nlab/show/symmetric+product+of+circles).