Structure of sign changes under the heat flow

Question

Let $f$ be a smooth function on $R^2$, and define $N_f$ to be the set of points $p$ such that the nodal set of $f$ ($\{x\in R^2: f(x)=0\}$) divided every neighborhood of $p$ into four regions. Indeed, the nodal set of $f$ looks like $"\times"$ around $p$ and $f$ changes sign four times on every small enough circle centered at $p$.

Let $u(t,x)\in C^{\infty}(R\times R^2)$ be a solution of the heat equation $u_t=\Delta u$ with $u(0,x)=u_0(x)$, and assume that $N_{u_0}=\emptyset$. I wonder if the heat flow can stably generate such points in time, i.e. can $ \cup_{t>0} N_{u(t,x)}$ contain an unbounded continuous curve $C$ in $R^3$ such that $C \cap N_{u(t,x)} \neq \emptyset$ for all $t>t_1$ for some $0<t_1\in R$.

Answer 1

Yes, this is possible (at least if you require only that $u$ be defined for $t>0$, which is the usual context for the heat equation, not for all $t \in {\bf R}$ as implied by "$u(t,x) \in C^\infty({\bf R} \times {\bf R}^2)$"):

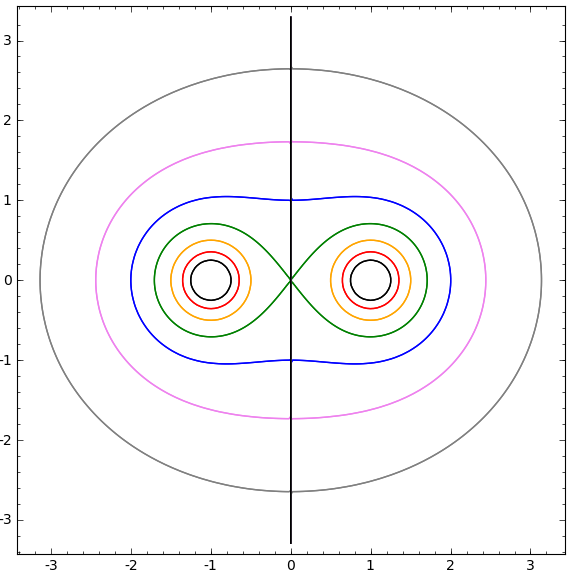
_{(source: harvard.edu)}

Here $u(t,x)$ is antisymmetric about the vertical axis $x_2=0$; thus the zero-set $V_{u(t,\cdot)} = \{x: u(t,x) = 0\}$ is always symmetric about that axis and contains it. The curved contours of that Sage plot, in black, red, orange, green, blue, purple, and gray, show the other component(s) of $V_{u,(t,\cdot)}$ for $t = t_1/8$, $t_1/4$, $t_1/2$, $t_1$, $2t_1$, $4t_1$, $8t_1$. The zero set $V_{u,(t,\cdot)}$ contains a $\ast$-shaped triple point for $t=t_1$, and two $+\,$-shaped double points for all $t > t_1$, at height proportional to $\pm \sqrt{t-t_1}$.

To obtain this function, start from the usual heat kernel $g(t,x) = (4\pi t)^{-1} \exp (-|x|^2/4t)$, and set $u(t,x) = \Delta_x(g(t,x-e_1)-g(t,x+e_1))$ where $e_1$ is the unit vector $(1,0)$. (To check that $u$ is a solution of the heat equation $u_t = \Delta_x u$, note that $g$ satisfies the heat equation and that the differential operators $\partial / \partial t$ and $\Delta_x$ commute with $\Delta_x$ and with translations by $\pm e_1$.) The nodal set of each term $\Delta_x(g(t,x\mp e_1))$ of $u$ is the circle of radius $2t^{1/2}$ about $\pm e_1$. For small $t$, the nodal set $V_{u(t,\cdot)}$ of the difference consists of the vertical axis and very close approximations to those circles. As $t$ increases, the circles grow and distort, eventually meeting to form a figure-eight at $t=t_1$ and a single closed curve for all $t > t_1$. The two double points for $t>t_1$ can be located as the zeros on the vertical axis $x_2=0$ of the partial derivative of $u(t,x)$ with respect to $x_1$.

Answer 2

Let me try to reformulate (too long for a comment) your question by specializing it to a more particular (and more stable) case. Let $u_0:\mathbb R^2\rightarrow \mathbb R$, be a (smooth) Morse function with index 0, i.e such that $$ du_0(x)=0\Longrightarrow u_0''(x) \text{ positive or negative definite}\Longleftrightarrow \det u''_0(x)>0. $$ The solution of the heat equation is $$ u(t,x)=(\Gamma(t)\ast u_0)(x),\quad \Gamma(t)(x)=(4πt)^{-1} \exp {-\frac{\vert x\vert^2}{4t}}. $$ A simple obvious result is that $u_0''(x)$ non-negative (as a two by two matrix) everywhere implies $u(t)_{xx}$ non-negative everywhere (as a two by two matrix), simply because the Gaussian kernel is non-negative. This gives a complete answer for initial data polynomial with degree less or equal than two. The same would work with a convexity (resp. concavity) assumption on $u_0$.

Now that does not prove that a Morse function with index 0 will not develop a saddle structure by the heat flow, but this reformulated question could be more tractable in this 2D setting.

Answer 3

If you by "can stably generate such points in time" asks if there exists some $u_0$ such that your statement holds, then just take the stationary solution $$u(t,x)=u_0(x):=x^2-y^2.$$ Then your curve $C$ is given by $(t,0,0)$, but I think that I have misunderstood your question since interpreting it as if there for any $u_0$ such that $N_{u_0}\ne \varnothing$ exists a curve $C$ such that..., it makes much more sense, and for which I don't know the answer.