(30Oct13: Now solved; see Addendum.)
Define a curve, a ratchet spiral, $S(r_0,\epsilon)$ as follows, where $r_0 > 0$ and $\epsilon < 1$.

$S(r_0,\epsilon)$ begins with the arc of an origin-centered circle of radius $r_0$, $C(r_0)$, starting from the point $p_0=(r_0,0)$. The arc follows $C(r_0)$ until it can jump radially by less than $\epsilon$ to a lattice point $p_1$ exterior to $C(r_0)$. Let $r_1=||p_1||$. The process continues, always jumping to a larger radius $r_{i+1}=||p_{i+1}||$ from an arc of $C(r_i)$ that enters a disk of radius $\epsilon$ around a lattice point $p_{i+1}$.
In the above example, $p_1=(3,1)$, $p_2=(3,2)$, and so on. Notice that the curve does not jump to $(3,4)$ because the distance is $5-3\sqrt{2} > \frac{3}{4}$.
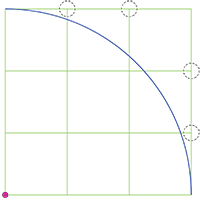
For sufficiently small $\epsilon$, the curve remains a bounded distance from the
origin. E.g., $S(3,\frac{1}{8})$ is just a circle:
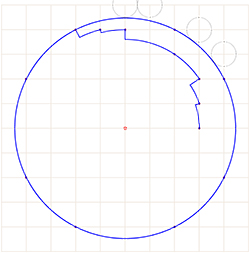
(Added.) Answering a question raised by Sam Hopkins in the comments, $S(3,\frac{1}{2})$
is not just a circle but remains bounded when the radius reaches $2\sqrt{5}$:
My questions are:
Q1. For which $r_0$ and $\epsilon$ (if any) does $S(r_0,\epsilon)$ spiral to infinity?
Q2. If any $S(r_0,\epsilon)$ spirals to infinity, how fast does $r_i$ grow as a function of its winding number?
Continuing the same example, after $59$ steps, the spiral has
wound once around the origin, and the radius exceeds $30$:
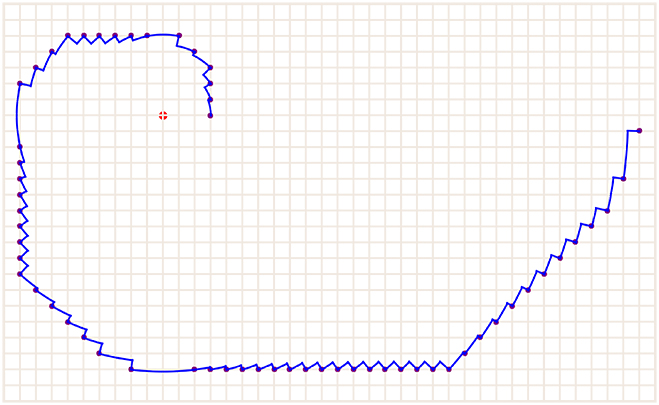
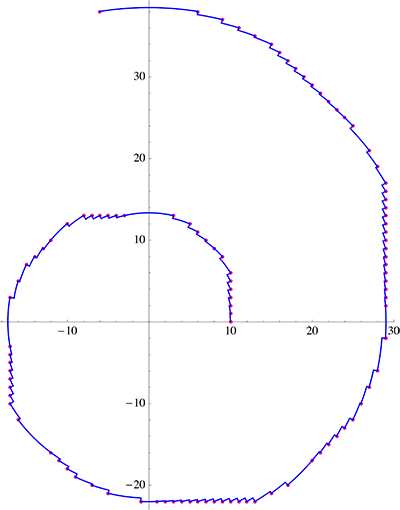
(Added.) And here is $S(10,\frac{1}{2})$,
an interesting contrast to $S(3,\frac{1}{2})$ above:
This question occurred to me while thinking about the Gauss circle problem
and Rational points on circular spirals.
Addendum. The main question (Q1) is now solved by Abhinav Kumar's answer to the related question, Lattice-point-free buffers around circles: For every $\epsilon > 0$, there is an $r_0$ such that the rachet spiral $S(r_0,\epsilon)$ is unbounded. The reason is that, for sufficiently large $r_0$, for all $r > r_0$, the "buffer" between $C(r)$ and the next exterior lattice point is smaller than $\epsilon$. Thus the spiral will always jump radially, since the jump is smaller than $\epsilon$. Conversely, for any $\epsilon > 0$, $S(r_0,\epsilon)$ might only be bounded (terminating in a circle) for "small" $r_0$, $r_0$ less than some finite bound. See Abhinav's answer for details.
