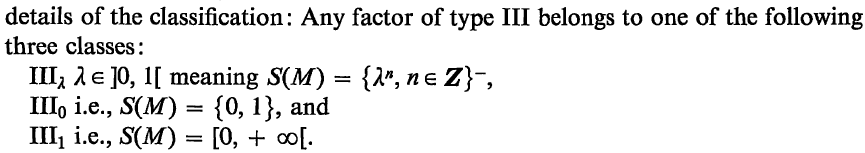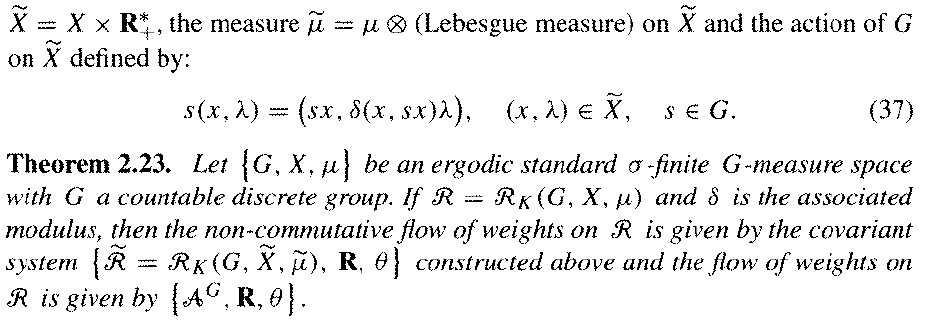8
$\begingroup$

We define below a von Neumann algebra $\mathcal{M}$ from an action of the free group on the circle, and we prove that $\mathcal{M}$ is a non-hyperfinite type ${\rm III}$ factor.

Question : Is $\mathcal{M}$ of type ${\rm III}_{0}$, ${\rm III}_{\lambda}$ or ${\rm III}_{1}$ ?

Definition : Let $s, r_{\theta}: \mathbb{R} / \mathbb{Z} \to \mathbb{R} / \mathbb{Z} $, defined by $s( x) = x^{2}$ (choosing representatives in $[0,1[$) and $r_{\theta} (x) = x+\theta$. Now, identifying $ \mathbb{R} / \mathbb{Z}$ and $\mathbb{S}^{1}$, we define the action $\alpha$ of $\mathbb{F}_{2} = \langle a, b \vert \ \rangle$, generated by $\alpha (a) = s$ and $\alpha (b) = r_{\theta}$ in Homeo($\mathbb{S}^{1}$).

Lemma: If $\theta$ is transcendental, the action $\alpha$ is faithful.
Proof: A relation $s^{n_{1}}r_{\theta}^{m_{1}}...s^{n_{k}}r_{\theta}^{m_{k}} = e $ can be translated into an algebraic equation in $x$ and $\theta$, where $\theta$ has to be a root $\forall x$. Then, if $\theta$ is transcendental, we are sure that there is no relation. $\square$

Remark: For a fixed transcendental $\theta$, each non-trivial relations can be realized for at most finitely many $x \in \mathbb{R} / \mathbb{Z}$, i.e. roots of the related algebraic equation.

Theorem: $\mathcal{M} = L^{\infty}(\mathbb{S}^{1}, Leb) \rtimes_{\alpha} \mathbb{F}_{2} $ is a non-hyperfinite type III factor.
Proof : The action $\alpha$ of $\mathbb{F}_{2}$ on $\mathbb{S}^{1}$ is:

  • (a) Measure class preserving: the set of null measure subspaces is invariant.
  • (b) Essentially free: a fixed point set for $\gamma \ne e$ is at most finite, so with null measure.
  • (c) Properly ergodic: ergodicity comes from irrational rotation, next, every $\mathbb{F}_{2}$-orbit have null measure.
  • (d) Non-amenable (Edit, Aug. 2018): for any $\eta > 0$, there is $n \in \mathbb{Z}_{>0}$ such that $\lfloor n\theta \rfloor < \eta$. Now, for $\lambda=Leb$ and $g = r_{\theta}^n$, $\partial (\lambda g)/\partial \lambda = 1$ because $\lambda$ is $g$-invariant. It follows that the action $\alpha$ is indiscrete, and then by the proposition below, it is non-amenable.

  • (e) Non equivalent measure preserving: by ergodicity, an equivalent invariant measure $m$ is proportional to $Leb$. Then $m([1/4 , 1/2]) = 2m([1/16 , 1/4])$, and by $\alpha(a)$ invariance, $m([1/4 , 1/2]) = m([1/16 , 1/4])$. In fact, the only invariant measure are $0$ or $\infty$.

    (a), (b), (c) give a factor, (d) gives non-hyperfinite, (e) gives a type ${\rm III}$. $\square$

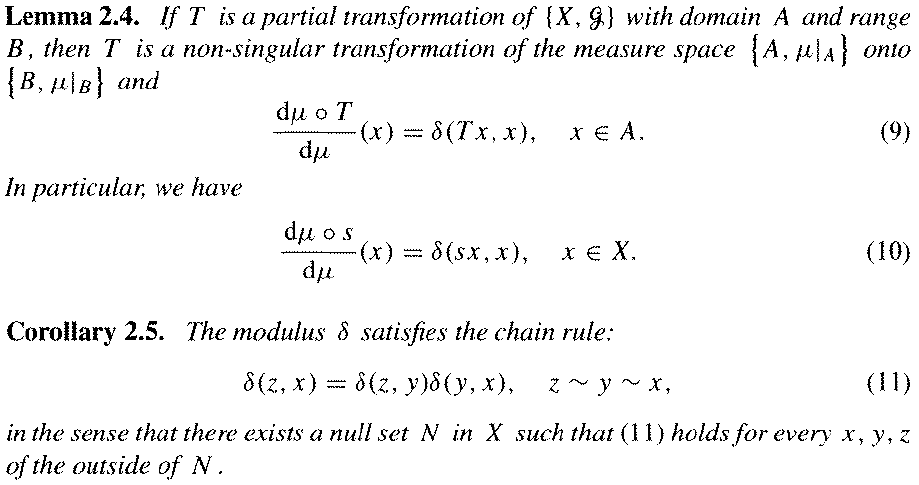
Here are two extracts of the following recent paper (April 2018):

Bartholdi, Laurent. Amenability of groups and $G$-sets. Sequences, groups, and number theory, 433--544, Trends Math., Birkhäuser/Springer, Cham, 2018.

enter image description here

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ I'm pretty sure that it's type $III_1$, because the following set is dense in $\mathbb R_{>0}$: $\{f'(x) : x\in \mathbb S^1, f\in F_2, f(x)=x\}$. $\endgroup$
    – André Henriques
    Commented Sep 8, 2013 at 15:09
  • 1
    $\begingroup$ I don't know the literature, so I can't point to a reference. But here's how things go: given an (let's say a.e. smooth) action of a group $\Gamma$ on a manifold $M$, you can form the bundle of densities $\Omega^{top}_{>0}M$, which is a principal bundle with structure group $\mathbb R_{>0}$. The action of $\Gamma$ on $M$ induces an action on $\Omega^{top}_{>0}M$, and the vN algebra $L^\infty(M)\rtimes \Gamma$ is a type $III_1$ factor iff the action of $\Gamma$ on $\Omega^{top}_{>0}M$ is ergodic. If that action is not ergodic, the vN algebra $L^\infty(\Omega^{top}_{\>0}M)^\Gamma$... $\endgroup$
    – André Henriques
    Commented Sep 8, 2013 at 16:58
  • 1
    $\begingroup$ ... is equipped with an action of $\mathbb R_{>0}$ (coming from the action on $\Omega^{top}_{>0}(M)$). This corresponds to a action of $\mathbb R_{>0}$ on some measure space $X$. If that action is transitive, it is equivalent to $\mathbb R_{>0}$ acting on $\mathbb R_{>0}/\mathbb Z^\lambda$ for some $\lambda\in(0,1)$, and the factor $L^\infty(M)\rtimes\Gamma$ is of type $III_\lambda$. Otherwise, $L^\infty(M)\rtimes\Gamma$ is of type $III_0$. $\endgroup$
    – André Henriques
    Commented Sep 8, 2013 at 17:05
  • $\begingroup$ I find the terminology "essentially free" confusing: it suggests that the action must be close in some sense to a free action, i. e. to an action with trivial stablizers. But the definition is such that it does not in principle exclude the case when there is a point stabilized by the whole group. $\endgroup$
    – მამუკა ჯიბლაძე
    Commented Aug 28, 2018 at 11:09
  • 1
    $\begingroup$ @მამუკაჯიბლაძე: Right. It is important to understand the word "essentially" as "up to a subset of null measure". Recall that an action is essentially free if $\lambda$-almost every point has a trivial stabilizer, namely $\lambda(\{ x \in X \ | \ G_x \neq 1 \}) = 0$. $\endgroup$
    – Sebastien Palcoux
    Commented Aug 28, 2018 at 12:39

1 Answer 1

Reset to default
2
$\begingroup$

Theorem: $\mathcal{M}$ is of type ${\rm III}_{1}$.

Proof: By Corollaire 3.3.4 below, we need to show that the ratio set of the action $\alpha$ is $[0, \infty)$. By the classification below, it is enough to prove that the interval $[0,2)$ is included in the ratio set.

Consider $r_{\theta}$ and $s$ on $[0,1)$ directly, i.e., $r_{\theta}(x) = \lfloor x+\theta \rfloor$ and $s(x) = x^2$.
Let $r \in [0,2)$, $\epsilon > 0$ and $A \subseteq [0,1)$ be a Borel set with $\mu(A)>0$.

Let $x_0 \in A$ such that for any neighborhood $V$ of $x_0$ in $[0,1)$ we have $\mu(V \cap A)>0$.
Consider integers $n,m$ and let $f:=r^n_{\theta} \circ s \circ r^m_{\theta}$.

Then $f(x) = \lfloor \lfloor x + m\theta \rfloor^2 + n\theta \rfloor$ and $f'(x) = 2\lfloor x + m\theta \rfloor$. Note that $f$ depends on the integers $n$ and $m$, whereas $f'$ depends on $m$ only (and is well-defined up to a finite set).

By ergodicity, we can choose $m$ such that $|2r^m_{\theta}(x_0)-r|<\epsilon$, so that for a sufficiently small neighborhood $V$ of $x_0$, $|f'(x)-r|<\epsilon$ for all $x \in V$. Let $C:=V \cap A$ and $D:=s \circ r^m_{\theta}(C)$. Then $\mu(C)>0$ and $\mu(D)>0$, and by definition of ergodicity, we can choose $n$ such that $\mu(C \cap r^n_{\theta}(D))>0$. Thus, for $B= f^{-1}(C) \cap C = f^{-1}(C \cap r^n_{\theta}(D))$ we have:

  • $\mu(B)>0$,
  • $B \cup f(B) \subset A$,
  • $|f'(x)-r|<\epsilon$, for all $x \in B$.

The result follows. $\square$

Remark: If we replace $s:x \mapsto x^2$ by $s_n: x \mapsto x^n$ with $n \ge 2$, we generate a von Neumann algebra $\mathcal{M}_n$ which is also a non-hyperfinite ${\rm III}_1$ factor (the proof is similar). Are they isomorphic?
It should work as well if we replace $s$ by any bijection of $[0,1)$ polynomial of degree $\ge 2$.

An extract of the following paper of Alain Connes (page 88):
Connes, A. Structure theory for Type III factors. Proceedings of the International Congress of Mathematicians (Vancouver, B. C., 1974), Vol. 2, pp. 87--91. Canad. Math. Congress, Montreal, Que., 1975.

enter image description here

An extract of the following paper of Alain Connes (page 195):
Connes, Alain. Une classification des facteurs de type ${\rm III}$. (French) Ann. Sci. École Norm. Sup. (4) 6 (1973), 133--252.

enter image description here

Conclusion: this action $\alpha$ of $\mathbb{F}_2$ on $\mathbb{S}^1$ generates a non-hyperfinite ${\rm III}_1$ factor $\mathcal{M}$.

Let $\Omega$ be a cyclic-separating vector (i.e. $\mathcal{M}\Omega$ and $\mathcal{M}'\Omega$ dense in $H$) and $\sigma=(\sigma_t^{\Omega})$ be the one-parameter modular group. Then $\widetilde{\mathcal{M}}:=\mathcal{M} \rtimes_{\sigma} \mathbb{R} $ is a ${\rm II}_{\infty}$ factor called the core of $\mathcal{M}$.
It is independent (up to isomorphism) of the choice of $\Omega$, and is isomorphic to $\mathcal{N} \otimes B(H)$, with $\mathcal{N}$ a non-hyperfinite ${\rm II}_1$ factor of fundamental group $\mathbb{R}_{+}^*$.

By Theorem 2.23 below, $$\widetilde{\mathcal{M}} = L^{\infty}(\mathbb{S}^1 \times \mathbb{R}_{+}^*, Leb) \rtimes_{\widetilde{\alpha}} \mathbb{F}_2$$ where the action $\widetilde{\alpha}$ of $\mathbb{F}_2$ on $\mathbb{S}^1 \times \mathbb{R}_{+}^*$ is given by $$\gamma(x,\lambda) = (\gamma(x), [\gamma'(x)]^{-1}\lambda),$$ with $x \in \mathbb{S}^1$, $\lambda \in \mathbb{R}_{+}^*$ and $\gamma \in \mathbb{F}_2$ identified with $\alpha(\gamma)$ or $\widetilde{\alpha}(\gamma)$ when appropriate.

Remarks and questions:
Note that $\widetilde{\mathcal{M}}$ has a Cartan subalgebra. Can we deduce that $\mathcal{N}$ has also a Cartan subalgebra?
If so, $\mathcal{N}$ cannot be isomorphic to $L(\mathbb{F}_2)$, because this last has no Cartan subalgebra, by this paper of Dan Voiculescu. Anyway, can we deduce that $L(\mathbb{F}_2)$ has also for fundamental group $\mathbb{R}_{+}^*$?
If so, it would solve the free group factors isomorphism problem, according to this paper of Florin Rădulescu.

Extracts of the following book of Masamichi Takesaki (pages 28 and 17):

Takesaki, M. Theory of operator algebras. III. Encyclopaedia of Mathematical Sciences, 127. Operator Algebras and Non-commutative Geometry, 8. Springer-Verlag, Berlin, 2003. xxii+548 pp.

The result below provides the core $\widetilde{\mathcal{R}}$ of the crossed-product $\mathcal{R}$.

enter image description here

From Corollary 2.5 below, $\delta(x,sx) = \delta(sx,x)^{-1}$, whereas Lemma 2.4 provides $\delta(sx,x)$.

enter image description here

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .