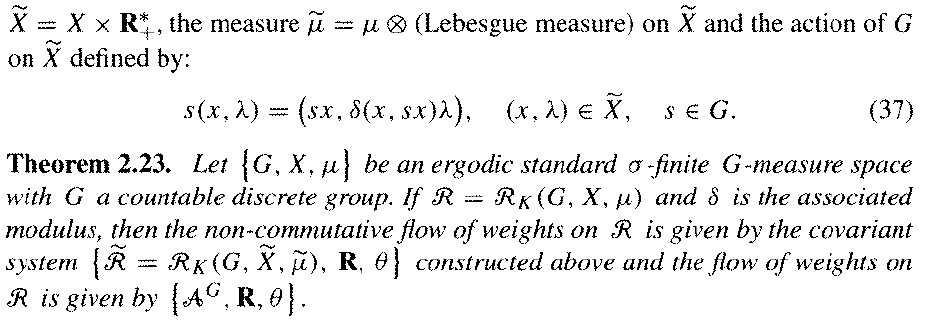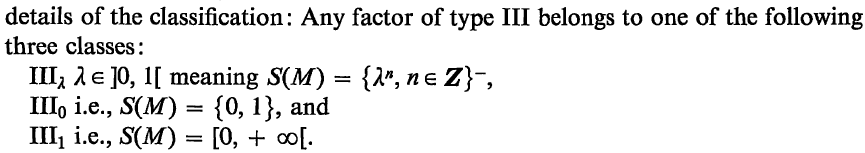Conclusion: this action of $\mathbb{F}_2$ on $\mathbb{S}^1$ generates a non-hyperfinite ${\rm III}_1$ factor $\mathcal{M}$.

Let $\Omega$ be a cyclic-separating vector (i.e. $\mathcal{M}\Omega$ and $\mathcal{M}'\Omega$ dense in $H$) and $\sigma=(\sigma_t^{\Omega})$ be the one-parameter modular map. Then $\mathcal{M} \rtimes_{\sigma} \mathbb{R} $ is isomorphic to $\mathcal{N} \otimes B(H)$, with $\mathcal{N}$ a ${\rm II}_1$ factor (independent up to iso. of the choice of $\Omega$) of fundamental group $\mathbb{R}_{+}^*$.

Question: What is $\mathcal{N}$? Is it isomorphic to $\mathcal{L}(\mathbb{F}_2)$?

If so, it would solve the free group factors isomorphism problem, according to this paper of Florin Rădulescu.

Remark: If we replace $s:x \mapsto x^2$ by $s_n: x \mapsto x^n$ with $n \ge 2$, we generate a von Neumann algebra $\mathcal{M}_n$ which is also a non-hyperfinite ${\rm III}_1$ factor (the proof is similar). Are they isomorphic?
It should work as well if we replace $s$ by any bijection of $[0,1)$ polynomial of degree $\ge 2$.

Remark: If we replace $s:x \mapsto x^2$ by $s_n: x \mapsto x^n$ with $n \ge 2$, we generate a von Neumann algebra $\mathcal{M}_n$ which is also a non-hyperfinite ${\rm III}_1$ factor (the proof is similar). Are they isomorphic?
It should work as well if we replace $s$ by any bijection of $[0,1)$ polynomial of degree $\ge 2$.

Conclusion: this action $\alpha$ of $\mathbb{F}_2$ on $\mathbb{S}^1$ generates a non-hyperfinite ${\rm III}_1$ factor $\mathcal{M}$.

Let $\Omega$ be a cyclic-separating vector (i.e. $\mathcal{M}\Omega$ and $\mathcal{M}'\Omega$ dense in $H$) and $\sigma=(\sigma_t^{\Omega})$ be the one-parameter modular group. Then $\widetilde{\mathcal{M}}:=\mathcal{M} \rtimes_{\sigma} \mathbb{R} $ is a ${\rm II}_{\infty}$ factor called the core of $\mathcal{M}$.
It is independent (up to isomorphism) of the choice of $\Omega$, and is isomorphic to $\mathcal{N} \otimes B(H)$, with $\mathcal{N}$ a non-hyperfinite ${\rm II}_1$ factor of fundamental group $\mathbb{R}_{+}^*$.

By Theorem 2.23 below, $$\widetilde{\mathcal{M}} = L^{\infty}(\mathbb{S}^1 \times \mathbb{R}_{+}^*, Leb) \rtimes_{\widetilde{\alpha}} \mathbb{F}_2$$ where the action $\widetilde{\alpha}$ of $\mathbb{F}_2$ on $\mathbb{S}^1 \times \mathbb{R}_{+}^*$ is given by $$\gamma(x,\lambda) = (\gamma(x), [\gamma'(x)]^{-1}\lambda),$$ with $x \in \mathbb{S}^1$, $\lambda \in \mathbb{R}_{+}^*$ and $\gamma \in \mathbb{F}_2$ identified with $\alpha(\gamma)$ or $\widetilde{\alpha}(\gamma)$ when appropriate.

Remarks and questions:
Note that $\widetilde{\mathcal{M}}$ has a Cartan subalgebra. Can we deduce that $\mathcal{N}$ has also a Cartan subalgebra?
If so, $\mathcal{N}$ cannot be isomorphic to $L(\mathbb{F}_2)$, because this last has no Cartan subalgebra, by this paper of Dan Voiculescu. Anyway, can we deduce that $L(\mathbb{F}_2)$ has also for fundamental group $\mathbb{R}_{+}^*$?
If so, it would solve the free group factors isomorphism problem, according to this paper of Florin Rădulescu.

Extracts of the following book of Masamichi Takesaki (pages 28 and 17):

Takesaki, M. Theory of operator algebras. III. Encyclopaedia of Mathematical Sciences, 127. Operator Algebras and Non-commutative Geometry, 8. Springer-Verlag, Berlin, 2003. xxii+548 pp.

The result below provides the core $\widetilde{\mathcal{R}}$ of the crossed-product $\mathcal{R}$.

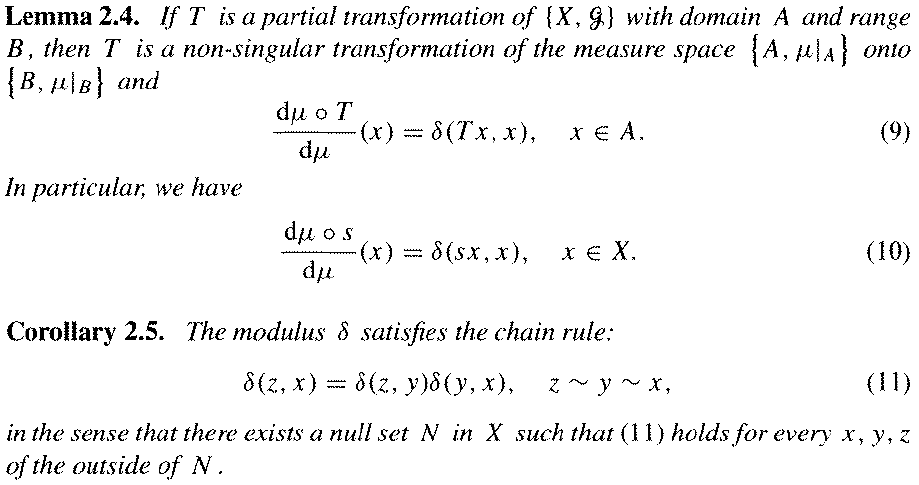
From Corollary 2.5 below, $\delta(x,sx) = \delta(sx,x)^{-1}$, whereas Lemma 2.4 provides $\delta(sx,x)$.

Theorem: $\mathcal{M}$ is of type ${\rm III}_{1}$.

Proof: By Corollaire 3.3.4 below, we need to show that the ratio set of the action $\alpha$ is $[0, \infty)$. By the classification below, it is enough to prove that the interval $[0,2)$ is included in the ratio set.

Consider $r_{\theta}$ and $s$ on $[0,1)$ directly, i.e., $r_{\theta}(x) = \lfloor x+\theta \rfloor$ and $s(x) = x^2$.
Let $r \in [0,2)$, $\epsilon > 0$ and $A \subseteq [0,1)$ be a Borel set with $\mu(A)>0$.

Let $x_0 \in A$ such that for any neighborhood $V$ of $x_0$ in $[0,1)$ we have $\mu(V \cap A)>0$.
Consider integers $n,m$ and let $f:=r^n_{\theta} \circ s \circ r^m_{\theta}$.

Then $f(x) = \lfloor \lfloor x + m\theta \rfloor^2 + n\theta \rfloor$ and $f'(x) = 2\lfloor x + m\theta \rfloor$. Note that $f$ depends on the integers $n$ and $m$, whereas $f'$ depends on $m$ only (and is well-defined up to a finite set).

By ergodicity, we can choose $m$ such that $|2r^m_{\theta}(x_0)-r|<\epsilon$, so that for a sufficiently small neighborhood $V$ of $x_0$, $|f'(x)-r|<\epsilon$ for all $x \in V$. Let $C:=V \cap A$ and $D:=s \circ r^m_{\theta}(C)$. Then $\mu(C)>0$ and $\mu(D)>0$, and by definition of ergodicity, we can choose $n$ such that $\mu(C \cap r^n_{\theta}(D))>0$. Thus, for $B= f^{-1}(C) \cap C = f^{-1}(C \cap r^n_{\theta}(D))$ we have:

• $\mu(B)>0$,
• $B \cup f(B) \subset A$,
• $|f'(x)-r|<\epsilon$, for all $x \in B$.

The result follows. $\square$

Conclusion: this action of $\mathbb{F}_2$ on $\mathbb{S}^1$ generates a non-hyperfinite ${\rm III}_1$ factor $\mathcal{M}$.

Let $\Omega$ be a cyclic-separating vector (i.e. $\mathcal{M}\Omega$ and $\mathcal{M}'\Omega$ dense in $H$) and $\sigma=(\sigma_t^{\Omega})$ be the one-parameter modular map. Then $\mathcal{M} \rtimes_{\sigma} \mathbb{R} $ is isomorphic to $\mathcal{N} \otimes B(H)$, with $\mathcal{N}$ a ${\rm II}_1$ factor (independent up to iso. of the choice of $\Omega$) of fundamental group $\mathbb{R}_{+}^*$.

Question: What is $\mathcal{N}$? Is it isomorphic to $\mathcal{L}(\mathbb{F}_2)$?

If so, it would solve the free group factors isomorphism problem, according to this paper of Florin Rădulescu.

An extract of the following paper of Alain Connes (page 88):
Connes, A. Structure theory for Type III factors. Proceedings of the International Congress of Mathematicians (Vancouver, B. C., 1974), Vol. 2, pp. 87--91. Canad. Math. Congress, Montreal, Que., 1975.

An extract of the following paper of Alain Connes (page 195):
Connes, Alain. Une classification des facteurs de type ${\rm III}$. (French) Ann. Sci. École Norm. Sup. (4) 6 (1973), 133--252.

Theorem: $\mathcal{M}$ is of type ${\rm III}_{1}$.

Proof: By Corollaire 3.3.4 below, we need to show that the ratio set of the action $\alpha$ is $[0, \infty)$. By the classification below, it is enough to prove that the interval $[0,2)$ is included in the ratio set.

Consider $r_{\theta}$ and $s$ on $[0,1)$ directly, i.e., $r_{\theta}(x) = \lfloor x+\theta \rfloor$ and $s(x) = x^2$.
Let $r \in [0,2)$, $\epsilon > 0$ and $A \subseteq [0,1)$ be a Borel set with $\mu(A)>0$.

Let $x_0 \in A$ such that for any neighborhood $V$ of $x_0$ in $[0,1)$ we have $\mu(V \cap A)>0$.
Consider integers $n,m$ and let $f:=r^n_{\theta} \circ s \circ r^m_{\theta}$.

Then $f(x) = \lfloor \lfloor x + m\theta \rfloor^2 + n\theta \rfloor$ and $f'(x) = 2\lfloor x + m\theta \rfloor$. Note that $f$ depends on the integers $n$ and $m$, whereas $f'$ depends on $m$ only (and is well-defined up to a finite set).

By ergodicity, we can choose $m$ such that $|2r^m_{\theta}(x_0)-r|<\epsilon$, so that for a sufficiently small neighborhood $V$ of $x_0$, $|f'(x)-r|<\epsilon$ for all $x \in V$. Let $C:=V \cap A$ and $D:=s \circ r^m_{\theta}(C)$. Then $\mu(C)>0$ and $\mu(D)>0$, and by definition of ergodicity, we can choose $n$ such that $\mu(C \cap r^n_{\theta}(D))>0$. Thus, for $B= f^{-1}(C) \cap C = f^{-1}(C \cap r^n_{\theta}(D))$ we have:

• $\mu(B)>0$,
• $B \cup f(B) \subset A$,
• $|f'(x)-r|<\epsilon$, for all $x \in B$.

The result follows. $\square$

Conclusion: this action of $\mathbb{F}_2$ on $\mathbb{S}^1$ generates a non-hyperfinite ${\rm III}_1$ factor $\mathcal{M}$.

Let $\Omega$ be a cyclic-separating vector (i.e. $\mathcal{M}\Omega$ and $\mathcal{M}'\Omega$ dense in $H$) and $\sigma=(\sigma_t^{\Omega})$ be the one-parameter modular map. Then $\mathcal{M} \rtimes_{\sigma} \mathbb{R} $ is isomorphic to $\mathcal{N} \otimes B(H)$, with $\mathcal{N}$ a ${\rm II}_1$ factor (independent up to iso. of the choice of $\Omega$) of fundamental group $\mathbb{R}_{+}^*$.

Question: What is $\mathcal{N}$? Is it isomorphic to $\mathcal{L}(\mathbb{F}_2)$?

If so, it would solve the free group factors isomorphism problem, according to this paper of Florin Rădulescu.

Remark: If we replace $s:x \mapsto x^2$ by $s_n: x \mapsto x^n$ with $n \ge 2$, we generate a von Neumann algebra $\mathcal{M}_n$ which is also a non-hyperfinite ${\rm III}_1$ factor (the proof is similar). Are they isomorphic?
It should work as well if we replace $s$ by any bijection of $[0,1)$ polynomial of degree $\ge 2$.

An extract of the following paper of Alain Connes (page 88):
Connes, A. Structure theory for Type III factors. Proceedings of the International Congress of Mathematicians (Vancouver, B. C., 1974), Vol. 2, pp. 87--91. Canad. Math. Congress, Montreal, Que., 1975.

An extract of the following paper of Alain Connes (page 195):
Connes, Alain. Une classification des facteurs de type ${\rm III}$. (French) Ann. Sci. École Norm. Sup. (4) 6 (1973), 133--252.