Fair cutting of the plane with lines

Question

An infinite countable family $\cal{L}$ of straight lines in the plane $\mathbb{R}^2$ forms a fair cutting of the plane if the following conditions are satisfied:

$\bullet$ No circle intersects infinitely many lines from the family $\cal{L}$;

$\bullet$ Let $L$ denote the union of the lines in $\cal{L}$. Each connected component of the complement of $L$ is a bounded set; the closure of each of these sets will be called a cell (observe that each cell is a convex polygon). This condition requires that the diameters of the cells formed by the cutting have a common upper bound;

Finally,

$\bullet$ All cells are of the same area.

We say that two fair cuttings are affinely equivalent if there exists an affine transformation of $\mathbb{R}^2$ sending the lines of one family onto the lines of the other. Also, we say that a fair cutting $\cal{L}$ is extra-fair, if, in at least one of its affine equivalents, all cells are congruent.

Four examples of mutually non-equivalent extra-fair cuttings are shown below.

Question 1. Is there a fair cutting whose at least one cell has more than four sides?

Question 2. Must all cells of a fair cutting have the same number of sides?

Question 3. Is every fair cutting extra-fair?

Question 4. Does there exist another example of a fair cuting, non-equivalent to any of the four pictured here?

Answer 1

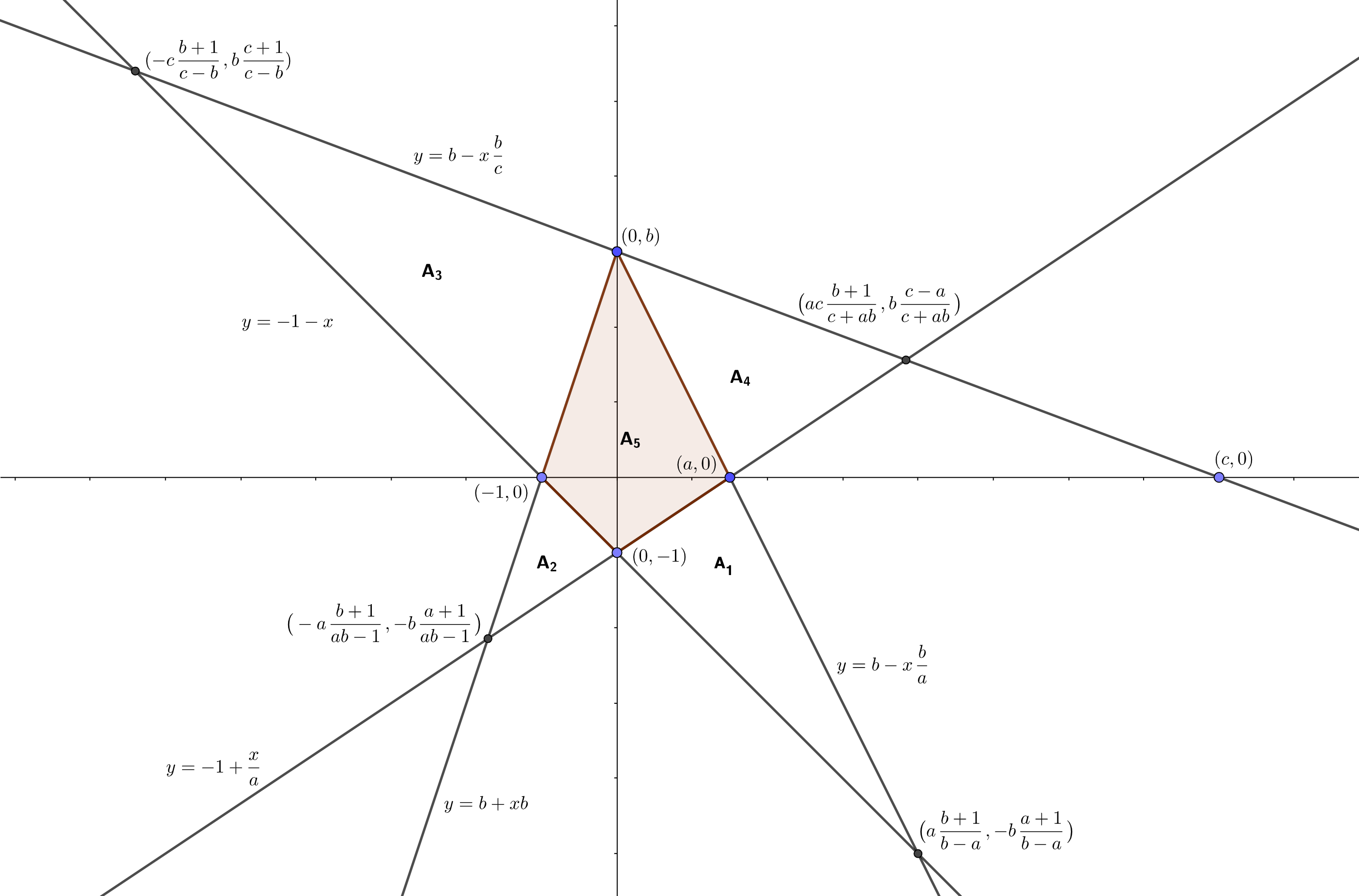
The answer to question 4 is no - every fair cutting is affinely equivalent to one of your four. This implies the answers to questions 1, 2, and 3 are no, yes, and yes, respectively. (Question 1 was already answered by Yaakov Baruch.)

My argument will use Yaakov Baruch's partial answer. Beyond what he does, there is one tricky algebraic calculation, and the rest is reasonably nice geometric arguments.

The general principle is that when one has inside a given fair cutting a large enough piece of one of the four cuttings on the list in the original post (i.e. one knows that certain cells from the cutting on the list are cells of the cutting, thus the lines through their edges are lines of the cutting and intersections of these lines are vertices of the cutting), one can extend this to get more of the cutting on the list.

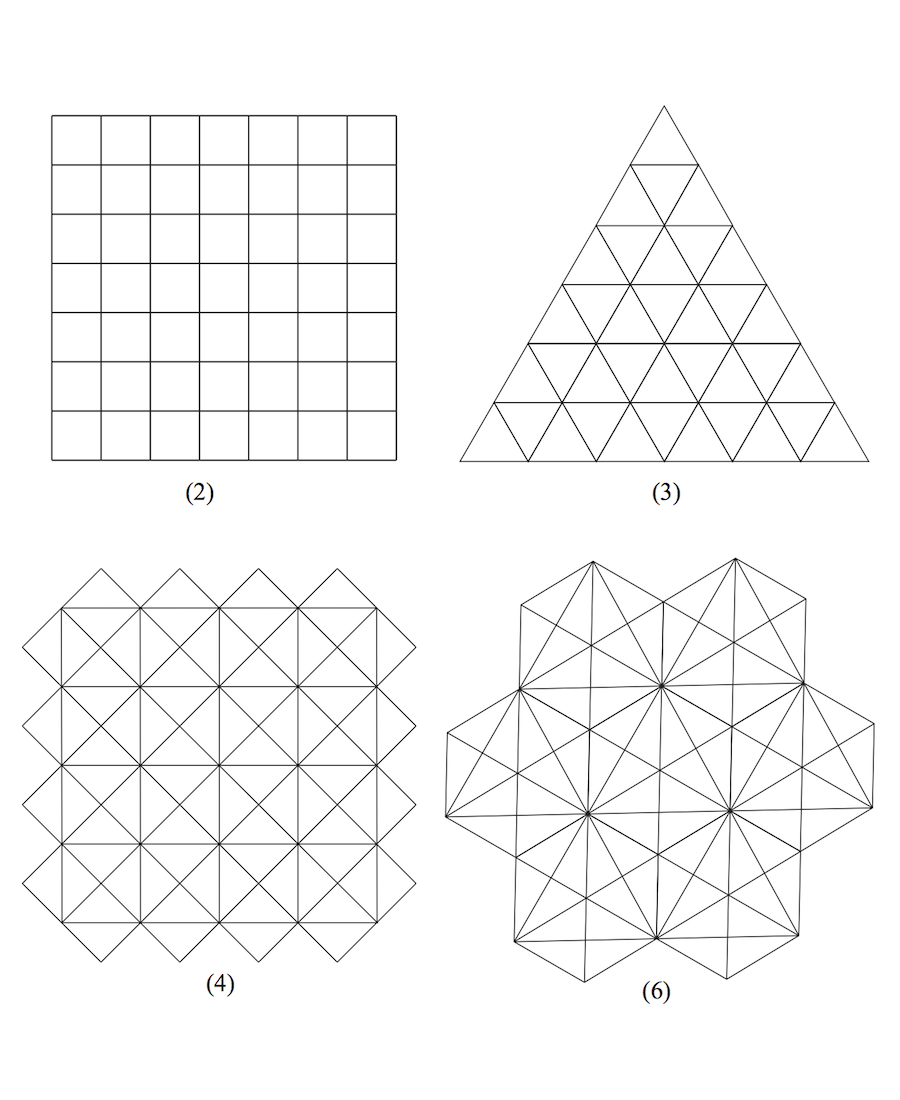
This gets easier the more one has, so the greatest difficulty is in starting out. However, finishing the argument requires getting to the point where the cutting repeats, allowing an inductive argument to fill the whole space. This is proportionally more difficult as the cutting gets less periodic (say, as the fundamental domain for the translation symmetries contains more and more cells), which is in increasing order from (2) to (3) to (4) to (6).

As a balancing force, as we get further in the argument we know certain configurations expand to a full cutting of the plane and therefore we can assume that those configurations don't appear, making the argument easier. Thus we want to go in increasing order - we proceed from proving that certain configurations produce (2), to proving that some other configurations produce (3), to proving that still other configurations produce (4), and finally prove that all other configurations produce (6).

I apologize for not including diagrams, which I am bad at drawing, in my answer, and trying to argue verbally / in coordinates as clearly as possible.

Step 1: Every fair tiling which contains a cell with four or more sides is equivalent to the square grid (2)

This was proven by Yaakov Baruch in his answer. It reduces us to considering tilings where every cell is a triangle.

Step 2: If two triangles in a fair tiling share an edge, their vertices are equidistant from that edge.

This follows from the area formula for triangles as one half base times height, i.e. one half the length of the edge times the distance of the vertex to the edge.

Step 3: A triangle in a fair tiling where every cell is a triangle such that every vertex is the intersection of three or more lines in the tiling, together with its three adjacent triangles, are affinely equivalent to four triangles from the equilateral triangular tiling (3).

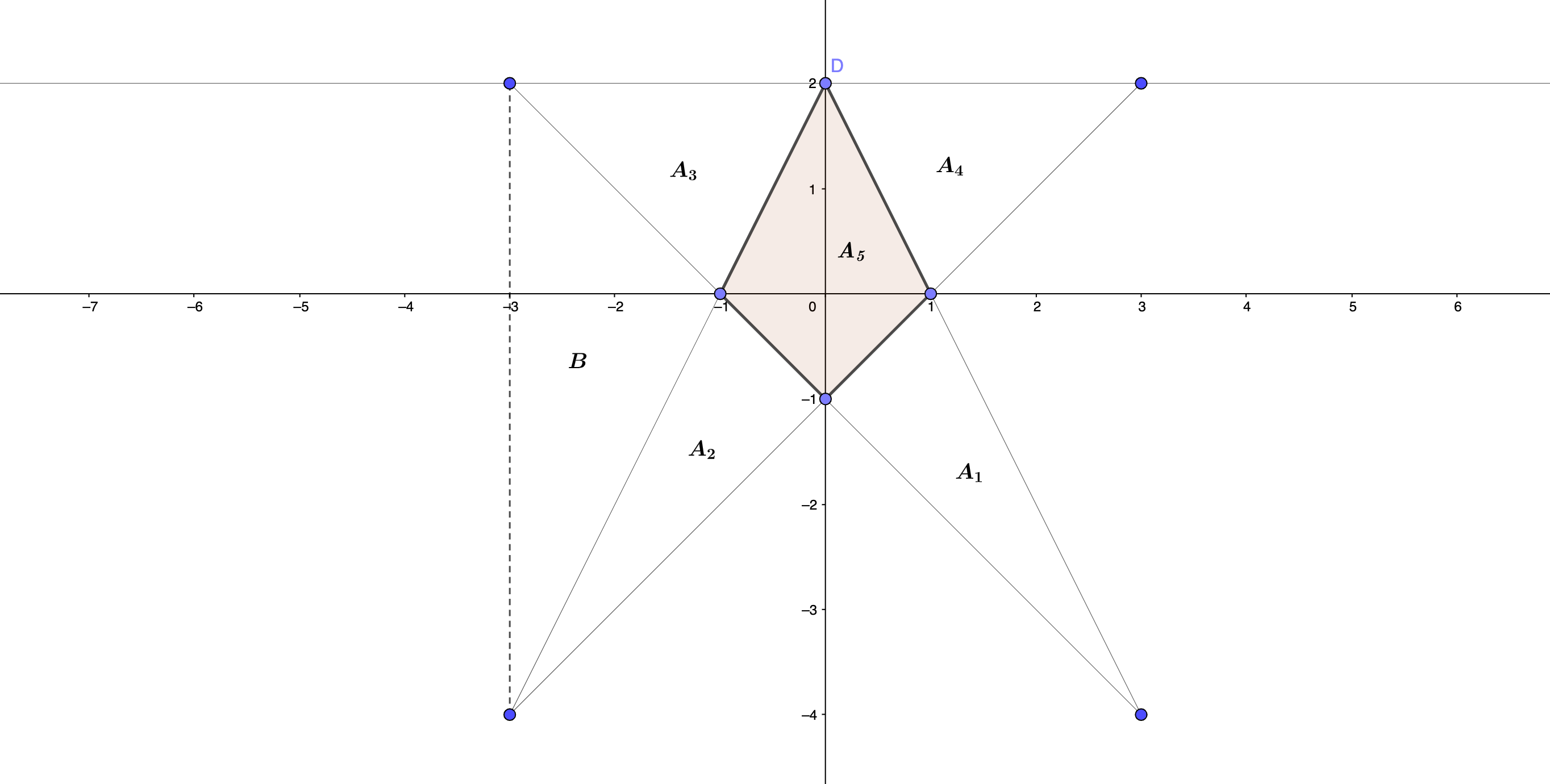
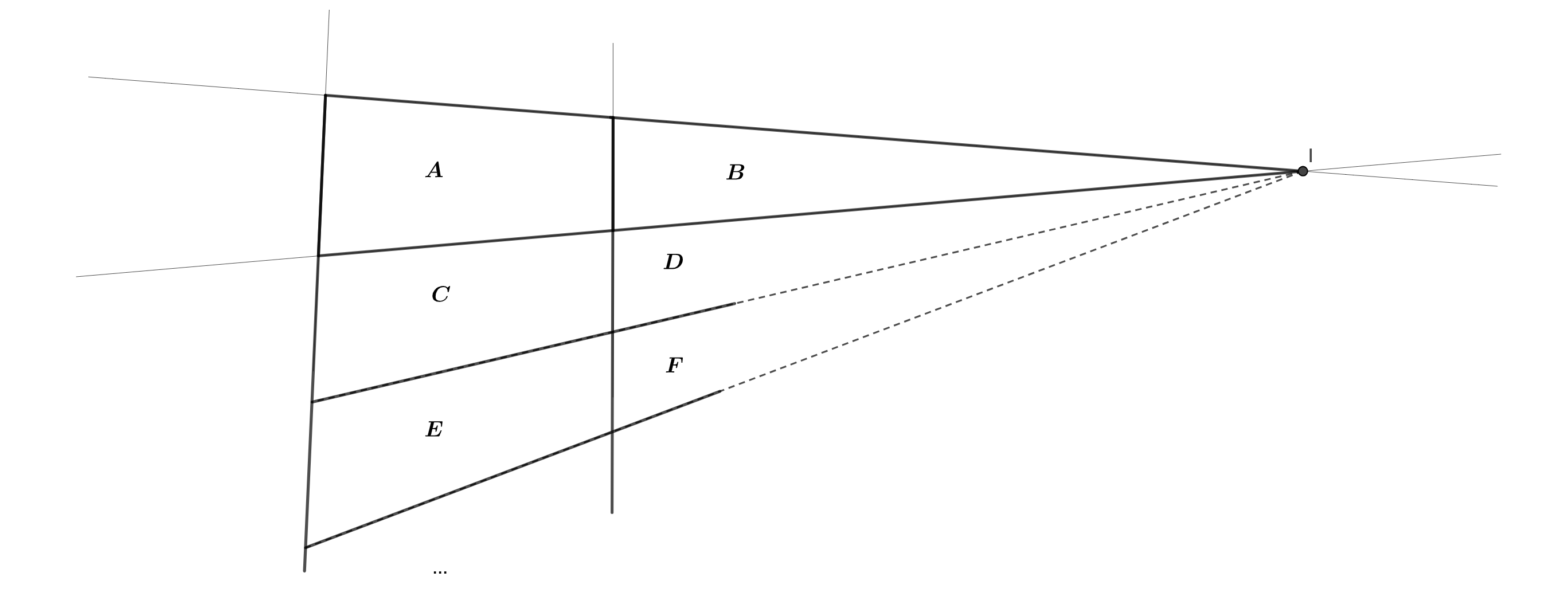
This is a mouthful, and is the hardest step. Let $P,Q,R$ be the vertices of the triangle in clockwise order. Because every cell is a triangle, each edge is shared with one other triangle. The triangle that shares the edge $PQ$, say, has a vertex $A_R$ whose distance from the line $PQ$ is equal to the distance of $R$ from $PQ$. This vertex $A_R$ therefore lies on the line $L_R$ parallel to $PQ$ and of that distance from $PQ$. The vertex $A_R$ must lie between the intersection of $PR$ and $L_R$ and the intersection of $QR$ and $L_R$, since otherwise those lines would intersect the triangle. Let $a_R$ be the distance from $A_R$ to to $PR \cap L_R$ divided by the distance from $PR \cap L_R$ to $QR \cap L_R$, so that $0 < a_R <1$. (If $a_R$ were exactly $0$, then $PR$ and $PQ$ would be the only lines through $P$.)

Define $a_P$ and $a_Q$ the same way, but rotated.

Let us now calculate in coordinates. After an affine transformation, we can take $P = (0,-1)$, $Q = (-1,0)$, $R = (0,0)$. Thus $L_P = \{x,y \mid y=1 \}$ and $L_Q = \{ x,y \mid x=1\} $. We have $A_P = ( 2 a_R - 2, 1)$ and $A_Q = (1, - 2 a_Q)$.

The triangle $Q R A_P$ contains the line $R A_P$ between $ ( 2 a_R - 2, 1)$ and $(0,0)$ given by the equation $x = (2a_R -2) y$. If $A_Q$ lies to the right of this line then this line intersects the triangle $PR A_Q$, which is impossible, so we must have $$1 \leq (2 a_R -2) ( -2 a_Q)$$ or $$ a_Q \geq \frac{1}{ 4 -4 a_R} = a_R + \frac{ (2 a_R -1)^2}{4 -4 a_R} \geq a_R $$ with equality only if $a_R =\frac{1}{2}$.

Symmetrically, we have $$ a_P \geq a_Q \geq a_R \geq a_P$$ so $$a_P = a_Q = a_R =\frac{1}{2}$$

so $A_P = (-1,1)$, $A_Q= (1,-1)$, $A_R = (-1,-1)$, which together with $P,Q,R$ are indeed affine equivalent to four triangles from the equilateral triangular tiling (3).

Step 4: A fair tiling where every cell is a triangle, containing one triangle such that every vertex is the intersection of at least three lines, is affinely equivalent to (3).

From Step 3, we may assume, up to affine transformation, that the tiling contains four triangles of the triangular tiling (3). For example, we can say it contains the vertices $(-1,1)$, $(0,0)$, $(1,-1)$, $(-1,0)$, $(0,-1)$, $(-1,-1)$, the lines $x+y=0$, $x+y=-1$, $x=0$, $x=-1$, $y=0$, $y=-1$, and the four triangles bounded by those lines.

We will show any fair tiling, where every cell is a triangle, containing those, contains also the reflection of those four triangles around the line $x=y=0$. Iterating this, reflecting in any desired direction, we get all the triangles of the triangular tiling.

To show this, consider the triangle which shares the edge between $(1,-1)$ and $(0,0)$ with the triangle $(1,-1),(0,0), (0,-1)$. By step 2, its third vertex $V$ must satisfy $x+y=1$. If the edge connecting $V$ to $(0,0)$ is not one of our existing lines, then there is another line through $(0,0)$, which intersects one of our existing triangles, contradicting the assumption that these are cells of the tiling. Therefore, $V$ must lie on one of the lines through $(0,0)$ - either $x=0$ or $y=0$. If $V$ lies on $x=0$ then this triangle is intersected by the line $y=0$ so $V$ lies on $x=0$, that is $V = (0,1)$. Thus our tiling contains the edge $x=1$ between $(1,-1)$ and $(0,0)$.

Symmetrically, our tiling contains the vertex $(1,0)$ and the edge $y=1$. The edges $x=0$, $x=1$, $y=0$, $y=1$ bound a square. Since our cells have area $1/2$, we must have another edge bisecting the square into two triangles. This can be either $x=y$ or $x+y=1$, but $x=y$ would bisect the triangle with vertices $(0,0)$, $(-1,0)$, $(0,-1)$ already assumed, so it must contain $(x+y=1)$.

It follows that the tiling contains the vertices $(-1,1), (0,0), (1,-1) , (0,1), (1,0), (1,1)$, the edges $x=0$, $x=1$, $y=0$, $y=1$, $x+y=0$, $x+y=1$, and the four triangles bounded by these edges. This is affine-equivalent to our starting configuration, so we may iterate, covering the plane.

Thus, we may assume every triangle has a vertex which is the intersection of only two lines.

Step 5: In a fair tiling where every cell is a triangle, a vertex which is the intersection of only two lines, together with the four triangles adjacent to it, form a parallelogram.

Let $L_1$ and $L_2$ be the two lines intersecting at the vertex $V$. Let $A$ and $C$ be the two vertices on the line $L_1$ closest to $V$, and let $B$ and $D$ be the two vertices on $L_2$ closes to $V$. Then $AVB, BVC, CVD, DVA$ must be the four triangles adjacent to $V$. By step 2, $A$ and $C$ are equidistant from $L_2$. Because they each lie on $L_1$, they are equidistant from $V$ - $V$ is the midpoint of $AC$. Similarly $V$ is the midpoint of $BD$. Thus ABCD is a parallelogram.

Step 6: The parallelograms constructed in Step 5 can't overlap each other. In a fair tiling where every cell is a triangle and every triangle has one of its four vertices the intersection of only two lines, the plane is tiled by such parallelograms.

If two parallelograms of this form overlapped, an internal edge of one would be an external edge of the other. The internal edges all touch the center vertex, which is the intersection of two lines, but the external edges only touch the external vertices, which are each the intersection of at least three lines - the two sides of the parallelogram plus the line through the center. So this is impossible.

By step 5, every triangle lies in a parallelogram, and by step 6, the parallelograms overlap, so indeed they cannot tile the plane.

Step 7: A fair tiling where every cell is a triangle and every triangle has a vertex which is the intersection of only two lines, which contains two parallelograms of step 5 which are equivalent under translation and share an edge, must be affine-equivalent to the quadrisected square tiling (4).

Every parallelogram is affine-equivalent to any given square, say the square with vertices $(0,0),(2,0), (0,2), (2,2)$. Without loss of generality, our two adjacent parallelograms are that one and the square with vertices $(2,0), (2,2), (4,0), (4,2)$.

Thus our tiling contains the vertices $(0,0), (0,2), (1,1) , (2,0) ,(2,2), (3,1), (4,0), (4,2)$, the lines $x=0, x=2, x=4, y=0, y=2, x+y=2, x+y=4, x=y, x=y+2$ and the eight triangles with vertices among those eight vertices bounded by those nine lines.

Using this, we will show that our tiling contains the same configuration with $y$ shifted vertically by $2$. Iterating, and rotating, we get the whole square lattice.

To do this, note that the triangles in our tiling have area $1$, and the lines $y=2$, $x=0$, $x+y=4$ of our tiling bound the triangle $(0,2),(0,4), (2,2)$ with area $2$, so this triangle must be the union of two triangular cells of the tiling. One of them must share the edge edge $(2,0),(2,2)$ with the triangle $(2,0),(2,2),(1,1)$. By step 2, its third vertex $V$ must be on the line $y=3$, hence be either $(1,3)$ or $(0,3)$, since when cutting a triangle into two triangles the only new vertices are on the edges of the triangle. If it's $(0,3)$ then the line $y = 3 -x/2$ lies in the tiling and intersects the already-existing triangle $(2,2), (4,2), (2,0)$, which is a contradiction, so the last vertex must be $(1,3)$.

Thus the edge $y=x+2$ lies in our tiling. The edges $x=2, x=0, y=2, y=x+2, y+x=2$ define the triangles $\{(0,4), (1,3), (0,2)\}$, $\{(0,2), (1,3), (2,2)\}$, and $\{(1,3), (2,2), (2,4)\}$, which all must be cells of our tiling. Any lines other than these which intersect the triangle $(0,4), (1,3), (2,4)$ would intersect one of those three tiles, contradicting the fact that they are cells of our tiling. Thus the triangle $(0,4), (1,3), (2,4)$ is contained in a cell of the tiling, and since it has area $1$, must be a cell of the tiling, so the edge $y=4$ lies in our tiling.

Symmetrically, the vertex $(3,3)$ and the edge $x+y=6$ lies in our tiling.

So our tiling contains the edges $y=2, y=4, x=0, x=2,x=4, x+y=4, x+y=6, y=x, y=x+2$, the vertices $(0,2), (0,4), (1,3), (2,2), (2,4), (3,3), (4,2),(4,4)$, and the eight triangles bounded by those lines and with vertices among those vertices, which indeed is the translation of our original configuration.

It also contains our original configuration with $x$ and $y$ swapped, allowing us to travel in the $x$ direction. Iterating, we fill out the tiling (4).

Step 8: In a fair tiling where every cell is a triangle and every triangle has a vertex which is the intersection of only two lines, if one of the parallelograms constructed in step 5 is $(0,0),(1,0),(0,1),(1,1)$, then the tiling contains the lines $x=n$ and $y=n$ for every integer $n$

By symmetry, it suffices to check this for the lines $y=n$ for positive integers $n$. Because, by step 6, the plane is tiled by parallelograms, the edge $(1,0), (1,1)$ is shared by another parallelogram. Since every parallelogram contains four cells, they all have the same area $1$. The parallelogram must have another edge parallel to this one, and because it has area $1$, that edge must lie on the line $y=2$. That edge is then shared by a third parallelogram, which must have a third parallel edge, which for the same reason must lie on the line $y=3$. Iterating, we have the claim.

Step 9: A fair tiling where every cell is a triangle and every triangle has a vertex which is the intersection of only two lines, which does not contain two parallelograms which are translates of each other and share an edge is affine-equivalent to the tiling (6).

Without loss of generality, one of the parallelograms is $(0,0),(1,0),(0,1), (1,1)$, so by step 8 the tiling contains the lines $x=n$ and $y=n$ for integer $n$. Now consider the parallelogram adjacent to the edge $(0,1), (1,1)$. Because it also has area $1$, its other vertices have the form $(x,2), (1+x,2)$ for some real $x$. By assumption, $x \neq 0$. By a reflection symmetry in $y$, we may assume $x \neq 0$. The line $x=1$ lies in our tiling and intersects this parallelogram. It therefore must pass through the center of the parallelogram, which implies $x=1$.

So our tiling contains the parallelogram $(0,1), (1,1), (1,2), (2,2)$. This one is affine-equivalent to $(0,0),(1,0),(0,1), (1,1)$ under the transformation $(x,y) \mapsto ( x+y, y+1)$ so by an affine-equivalent form of step 8, the tiling contains the edges of the form $x+y=n$ for an integer $n$.

The edges $x=n, y=n, x+y=n$ divide the plane into triangles of area $1/2$, each of which contains two cells of the tiling, and therefore each of which is bisected into two triangles by an edge. Thus each vertex of the tiling is either a vertex of this triangular grid or the midpoint of an edge of the triangular grid. the vertices of the triangular grid lie on at least three lines, so only the midpoints of edges may be centers of parallelograms - thus each parallelogram consists of two triangles from this grid.

There are three types of parallelograms formed by three triangles of this grid, up to translation. No two congruent ones can be adjacent. For each triangle adjacent to a fixed parallelogram, there are two possible parallelograms it can be contained in, and one is congruent to the original, so it must be the other. Iterating this, our one starting parallelogram forces a tiling of the other grid by parallelograms, which gives a fair tiling of the plane, which indeed is (6).

Answer 2

This is not a full answer to the question, but it is perhaps a start, and too long for a comment anyway.

If $n$ distinct lines intersect at a single point, let's say the intersection is regular if the angle between any two lines is a multiple of $\pi/n$. (That is, all the angles are evenly spaced.)

A fair cutting of the plane cannot contain a regular intersection of $n$ lines at a common point, unless $n = 2,3,4,6$ (as in your four pictures).

To see why this is true, let's argue that having a regular intersection of $n$ lines with $n \geq 7$ is impossible. Afterward we'll see how to modify the argument for $n = 5$. (The illustrations are all for $n=7$.)

$\ \ \ \ $(This is impossible.)

Suppose we have a fair cutting $\mathcal L$ containing $n$ lines, forming a regular intersection at some point $p$, as in the picture above.

Let $L$ be a line in $\mathcal L$, other than those going through $p$, whose distance to $p$ is minimal. Let $q$ be the point on $L$ with minimal distance to $p$. We consider two cases: either $q$ lies on one of our $n$ lines or not.

Drawing the line through $q$, in the first case we get an immediate contradiction: there is a cell containing $p$ and $q$ on its boundary, but the adjacent regions we've just created with our line through $q$ are not an integer multiple of its area. (The area is larger, but having $n \geq 7$, one can show that it is not twice as large.) This is absurd, because these regions should be a finite union of cells.

Now consider the second case, where $q$ is on one of our seven lines through $p$. In this case the line $L$ through $q$ is perpendicular to one of our lines through $p$. This creates two cells containing $p$ and $q$ on their boundary, each of area $A$. But it also creates another adjacent region, with area larger than $A$ but not larger than $2A$. Again, this is a contradiction, since this region should be a union of finitely many cells, each with area $A$.

For $n=5$, a similar argument works. The case where $q$ is on a line is exactly the same: we get bounded regions whose areas are not integer multiples of each other. The case where $q$ is not on a line requires just one extra step. In this case it is possible to make two adjacent regions with one double or triple the area of the other (but quadruple the area is impossible):

However, as you can see in the picture, this creates a third region too (the one to the right), and this region is not commensurable with the other two.

Answer 3

CLAIM. The only fair cutting with at least one quadrilateral is the square grid (2). It was already shown in the original answer (below) that there cannot be $n$-agons for $n\ge 5$.

LEMMA. No fair cutting can contain a non-simple quadrilateral (i.e. one with a non-simple vertex, intersection of more than 2 lines).

PROOF of Lemma. The proof in my original answer incidentally showed that, up to affine transformations, there is a unique quadrilateral with a non-simple vertex, such that none of its ears are smaller than the interior:

and in fact $A_1=A_2=A_3=A_4=A_5=3$. Consider then the region containing the triangle $B$, which has area $6$. The only way to cut that region into smaller tiles involves also cutting at least one of $A_3$, $A_5$ or $A_2$. Therefore the above pattern cannot exist in a fair cutting either.$\quad\quad\blacksquare$

PROOF of Claim.

Assume there is a quadrilateral with two opposite sides that are not parallel. By the Lemma the finite ear formed by those 2 sides will be decomposed into a sequence of quadrilaterals until the last piece, which is a triangle. Therefore we always must have a simple quadrilateral adjacent to a triangle:

Next consider the other 2 edges of $A$. On a side where they don't converge there can only be a sequence of simple quadrilaterals (by the Lemma again), starting with $C$:

In order for the tile at $D$ to not cut any of $A, B, C$ and to comply with the Lemma, the only possibility is for it to be a triangle, with its incomplete edge reaching exactly I:

The same reasoning can be repeated with $E$ and $F$, and so on.

The point I must be then be the intersection of infinitely many lines, contradicting the definition of a fair cutting. This proves that any quadrilateral in a fair cutting must be a simple parallelogram, and from there it's trivial to see that all parallelograms must be identical in order to have the same area. Such cutting is equivalent to the square grid by affine transformation. $\quad\quad\blacksquare$

NOTE. If the requirement that only finitely many lines intersect any circle were done away with, then a tiling satisfying all the other requirements would be given by this: all the lines through $(0,0)$ and $(0,n)$, together with all he lines through $(\sqrt{n},0)$ parallel to the $y$-axis. All its tiles have area $1/2$.

---

---

ORIGINAL ANSWER

This is a negative answer to Question 1.

As has been pointed out in Noam Elkies's comment, it suffices to prove that every convex polygon of $n\ge5$ sides has at least one side such that the external triangle (or ear) formed by it and the 2 adjacent sides has area less than that of the polygon.

---

It suffices to prove this for $n=5$ by considering the pentagon formed by any subset of 5 vertices of the polygon:

---

It's simpler to prove a stronger result.

CLAIM. A convex pentagon has at least two ears with areas strictly less than that of the pentagon. The result is optimal, in the sense that it's easy to construct pentagons with 3 ears larger than the interior.

COROLLARY: a convex $n$-agon has at most three ears with area $\ge$ interior area, and this is also optimal. (Hint: draw the chord spanning 2 consecutive ears, one $<$ and one $\ge$ interior area...)

PROOF of claim. First a few reductions, without loss of generality:

---

1) Assume each ear to be finite by rotating an edge around one of its endpoints in such a way that: - the infinite ear becomes finite, but still larger than any other finite ear, - a finite adjacent ear increases (and remains finite), - the pentagon's interior is reduced:

---

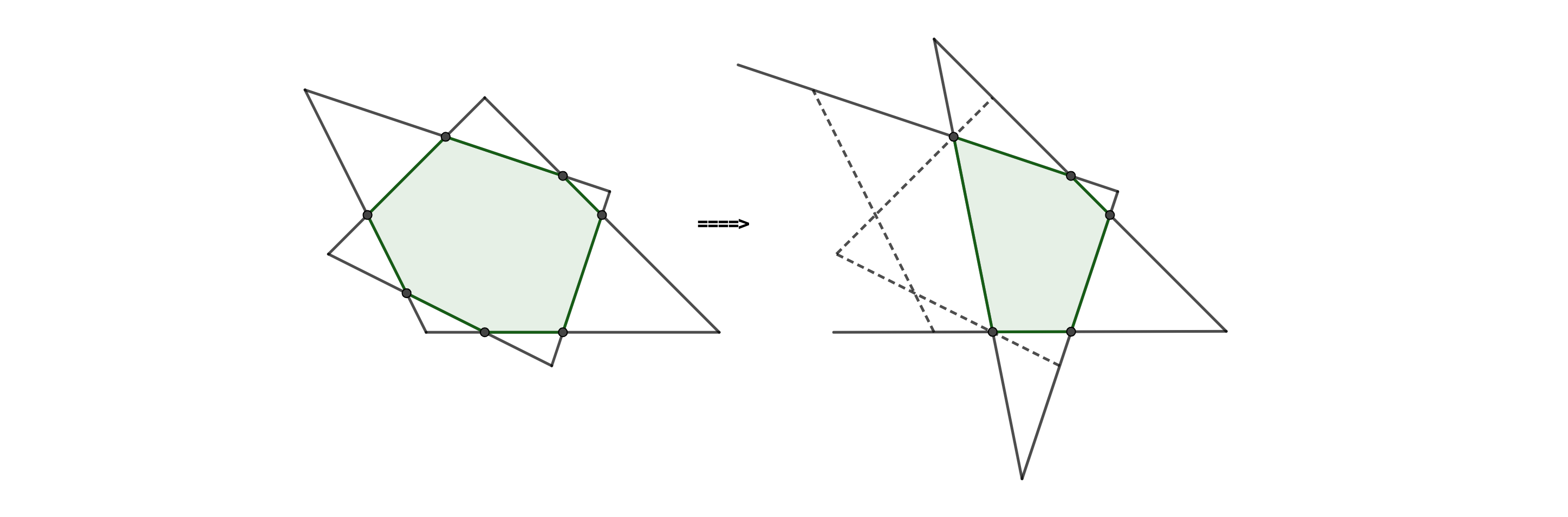
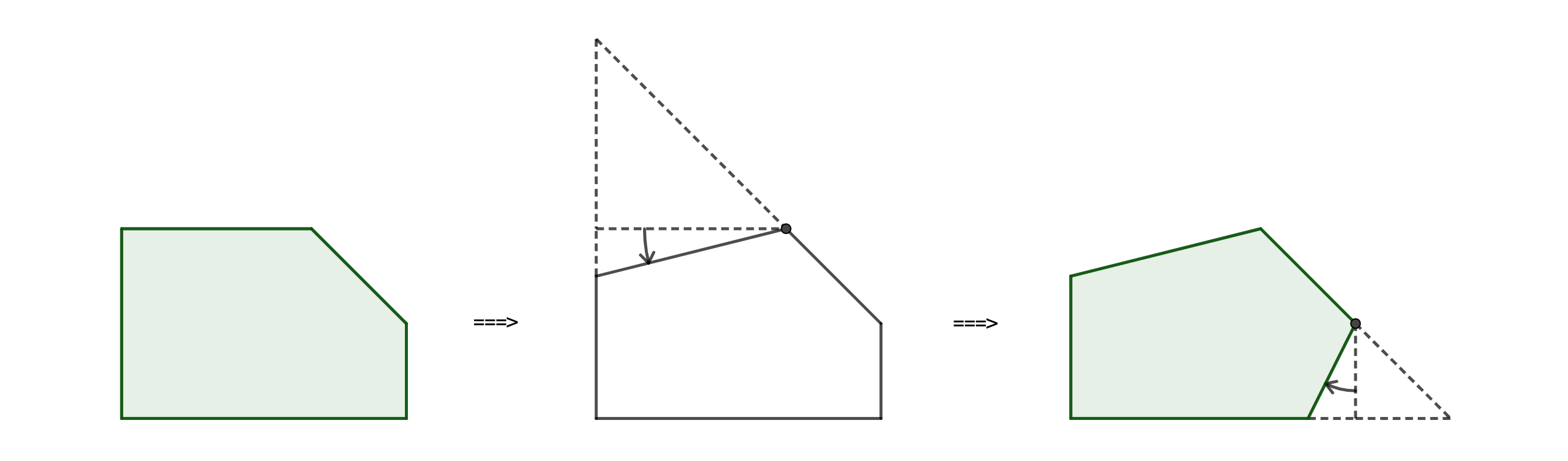
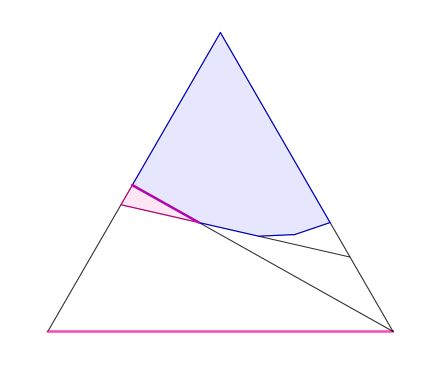
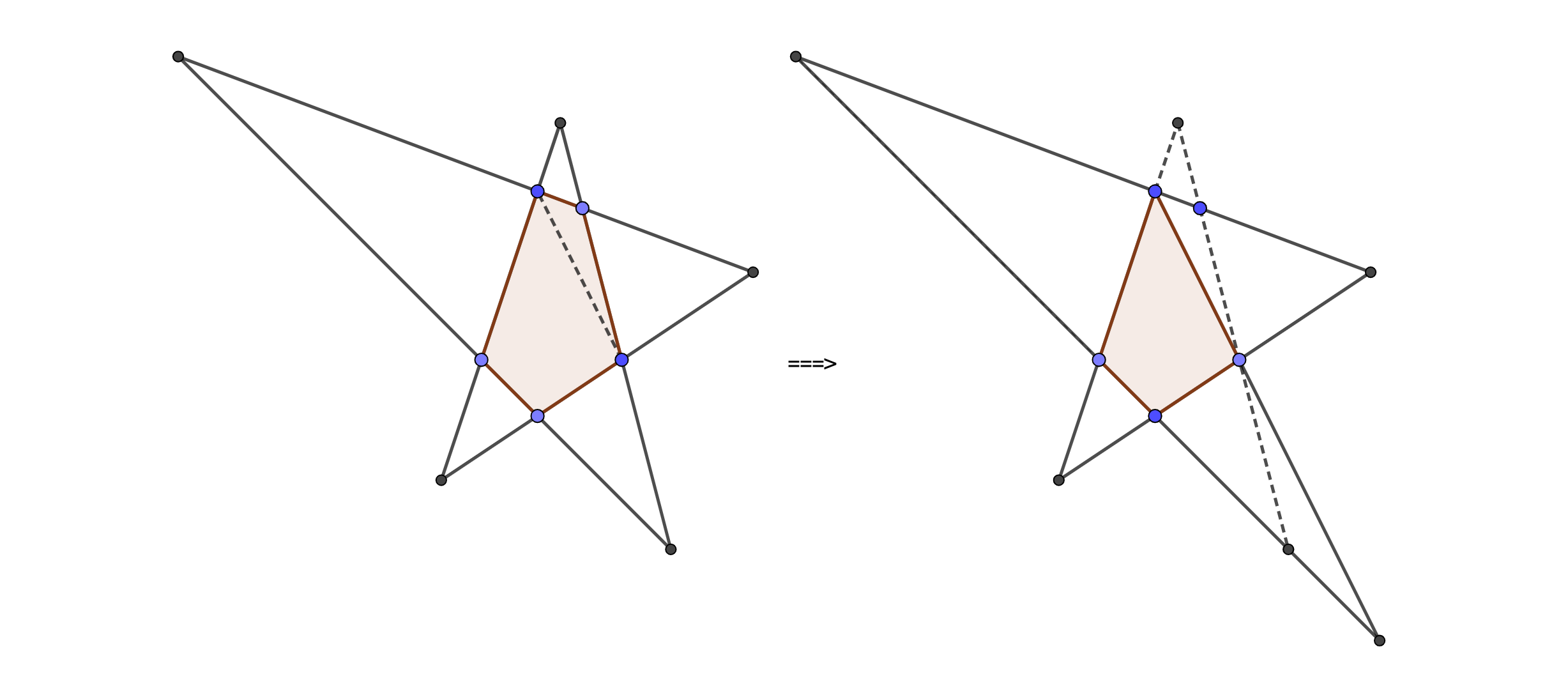2) The critical step: reduce the *smallest* ear to a point by again rotating one edge around its endpoint, thereby increasing the two ears near the rotation point, and *strictly* reducing the interior of the pentagon to a quadrilateral. (If necessary, repeat step 1) to make all ears finite again.) As a result of this step, it suffices now to prove that one of the new 4 non-degenerate ears has area less than, or equal to, that the quadrilateral's.

---

3) By an affine transformation one can assume that the diagonals of the quadrilateral lay on the $x$ and $y$ axes, their intersection in the origin and the end points at $(-1,0),(0,-1),(a,0)$ and $(0,b)$, with $ 1\le a<b$:

 

  Notice that $ a<b$ follows from $A_1<\infty$, and $c$ can be either $>a$, as in the figure, or $<-1$, or $\infty$.

---

  The 5 areas are now easily computed:

$$A_5=\frac{(1+a)(1+b)}{2}$$

$$A_1=A_5\frac{a}{b-a}$$

$$A_2=\frac{A_5}{ab-1}$$

$$A_3=A_5\frac{b|c+1|}{(1+a)|c-b|}$$

$$A_4=A_5\frac{ab|c-a|}{(1+a)|c+ab|}$$

From the third formula: $A_2\ge A_5\implies ab\le 2$.

Case $c>a$: $$ \displaystyle ab\le 2, a\ge 1 \implies A_4=A_5\frac{ab}{(1+a)}\cdot \frac{|c-a|}{|c+ab|}<A_5$$ and we are done.

Case $c<-1$: $$\displaystyle ab\le 2, a\ge 1 \implies A_3=A_5\frac{b|c+1|}{(1+a)|c-b|}\le A_5\frac{ab}{(1+a)}\cdot \frac{|c+1|}{|c-b|}< A_5$$ and again done.

Case $c=\infty$: $$\displaystyle ab\le 2, a\ge 1 \implies A_3=A_5\frac{b}{(1+a)}\le A_5\frac{ab}{(1+a)}\le A_5\quad \text{and}\quad A_4=A_5\frac{ab}{(1+a)}\le A_5$$

Here notice that the inequalities are not strict. In fact $A_1=A_2=A_3=A_4=A_5$ if and only if $a=1$, $b=2$. However this is sufficient since step 2) involved a strict decrease of the pentagon's area. $\quad\quad\blacksquare$

Answer 4

Here is, I think, a proof that the only fair cutting with no triangles is the one you call (2). Hopefully some ideas might help answer other questions.

I call “block” a finite union of cells.

Lemma. If, in a triangle-free fair cutting, there exists a triangular block such that one its (open) sides is not cut by any other line, then there exists a smaller such block inside of it.

This shows that such blocks in fact cannot exist in fair cuttings with no triangles, because then there would be infinitely many lines intersecting the block. I will show at the end that there is always such a block if one of the cells in not a parallelogram or a triangle. It means that if there are no triangles, all cells are parallelograms or triangles, so in fact they are all parallelograms (which means we are in the case (2)).

The proof of the lemma is essentially contained in the following picture.

Up to an affine transformation, the triangular block $T$ cannot be cut on its base, highlighted in pink. The top part of $T$ has to belong to some convex polygonal cell $C$, highlighted in blue (the apex cannot be cut, otherwise the base would be cut as well). This cell cannot use the base as part of its boundary, since the base cannot be cut, so the boundary defines a convex polygonal path from the left side of $T$ to the right one. Consider the two leftmost pieces of this path. Extending the second piece to the left, and using the left side of $T$, this defines a smaller triangular block, whose top side, the one touching $C$, cannot be cut. This concludes the proof of the lemma.

Now I want to show that any piece that is not a parallelogram or a triangle has to produce such a triangular block. First, consider any cell such that two consecutive (interior) angles sum to more than $\pi$. Then the three lines we consider (the ones defining the angles) form a triangle, and the side common to the two angles cannot be cut, otherwise the cell would actually be a block. This triangle is a block as in the lemma.

Let $C$ be a cell with $n$ sides and angles $\alpha_i$, indexed by $\mathbb Z/n\mathbb Z$ along the boundary. Then $$ \frac1n\sum_{i=0}^{n-1}(\alpha_i+\alpha_{i+1}) = \frac2n\sum_{i=0}^{n-1}\alpha_i = \frac{2n-4}n\pi. $$ If $C$ is a cell in a fair cutting with no triangles, then because of the previous reasoning, every pair of consecutive angles must sum to at most $\pi$. In particular, the average of all such possible sums has to be at most $\pi$, and $n\leq 4$. If $n$ is precisely $4$, then the average of all such sums is $\pi$, and all of them have to be at most $\pi$. This means that in fact, any two consecutive angles have to sum to $\pi$, so the sides are parallel, which is what we wanted to show. If one reverses the reasoning, we see that any cell that is not either a triangle or a parallelogram forces the existence of a triangular block as in the lemma.

Answer 5

I did not see the comment section under Yaakov Baruch's answer to this question. I think these ideas are interesting enough to be posted as an answer; I'm making it comminuty wiki. This provides a negative answer to your first question: all cells have to be triangles or quadrilaterals.

I call “block” a finite union of cells.

Consider a cell with at least 5 sides. It defines some triangular blocks based on each side, that I will call “ears”; see Yaakov Baruch's answer for pictures. Note that these ears can be degenerate: even if no two sides are parallel, they can be infinite, or, in a different mindset, they can contain the cell itself. This does not affect the proof, provided we consider by convention the area of such degenerate ears to be larger than that of the cell. The following fact is a rewriting of Yaakov Baruch's points a) and b).

Fact (Baruch, 2011). For any cell with at least 5 sides, at least one of the ears has area less than the cell itself.

I must admit I do not fully understand the proof of the pentagonal case (why would the wiggling of the line increase at least one of the changing areas?), but it is given in a comment to their answer, and the induction step is described in the answer itself, with some pictures. The fact implies directly that there is no cell with at least 5 sides.