Let $\cal C = \lbrace C_i \rbrace$ be a collection of rectifiable curves in the plane with the property that every unit-length segment meets at least one curve in at least one point. Call such a collection $\cal C$ a needle net: any unit-length "needle" is captured by the net.
I would like to find the sparsest needle net, sparse in the sense that the curves have minimum length per unit area. That is, the limit of $L/A$ of the ratio of the length $L$ of the curves within a region to that region's area $A$, as the region grows large, is as small as possible.
For example, a regular grid of orthogonal parallel lines
separated by $\sqrt{2}/2$ is a needle net: the diagonal of
each square cell of the grid has length $1$.
If I've calculated correctly, the length of its
curves (lines) within each unit area region $L/A$ is $2 \sqrt{2}$.
See left below, where a unit-length
diagonal is highlighted in red, and the region of the plane
I used to compute $L/A$ is marked.
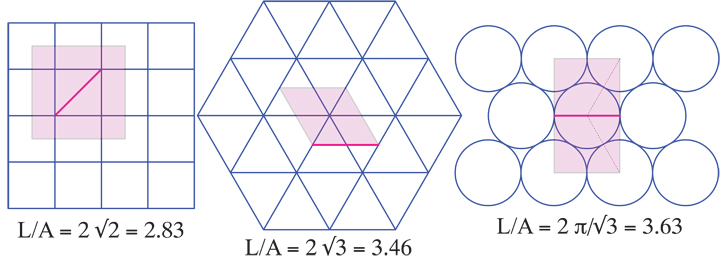
Again if I've calculated correctly, the equilateral-triangle tiling of
the plane obtained from three sets of parallel lines is less efficient,
and the packing arrangement of unit-diameter circles shown right above
is less efficient still.
Is the square-grid the sparsest needle net? This feels like a question that has been addressed before, perhaps in another guise. If so, a pointer would be welcomed. Thanks!
Update. Roland Bacher's more efficient needle net:

Is this the optimal net?
