CLAIM. The only fair cutting with at least one quadrilateral is the square grid (2).
LEMMA. No fair cutting can contain a non-simple quadrilateral (i.e. one with a non-simple vertex, intersection of more than 2 lines).
PROOF of Lemma. The proof in my original answer (below) incidentally showed that, up to affine transformations, there is a unique quadrilateral with a non-simple vertex, such that none of its ears are smaller than the interior:
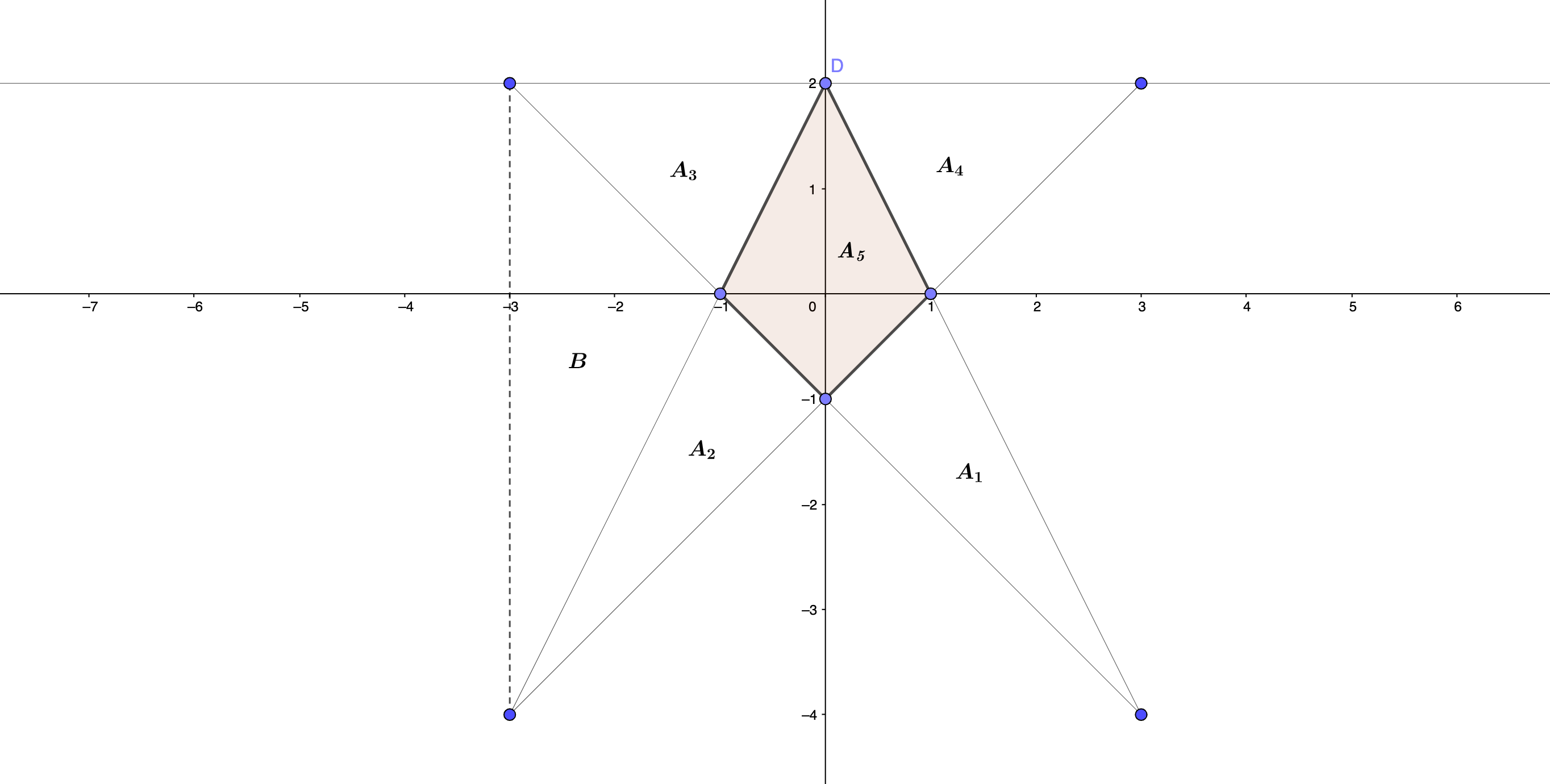
and in fact $A_1=A_2=A_3=A_4=A_5=3$. Consider then the region containing the triangle $B$, which has area $6$. The only way to cut that region into smaller tiles involves also cutting at least one of
$A_3$, $A_5$ or $A_2$. Therefore the above pattern cannot exist in a fair cutting either.$\quad\quad\blacksquare$
PROOF of Claim.
Assume there is a quadrilateral with two opposite sides that are not parallel. By the Lemma the finite ear formed by those 2 sides will be decomposed into a sequence of quadrilaterals until the last piece, which is a triangle. Therefore we always must have a simple quadrilateral adjacent to a triangle:
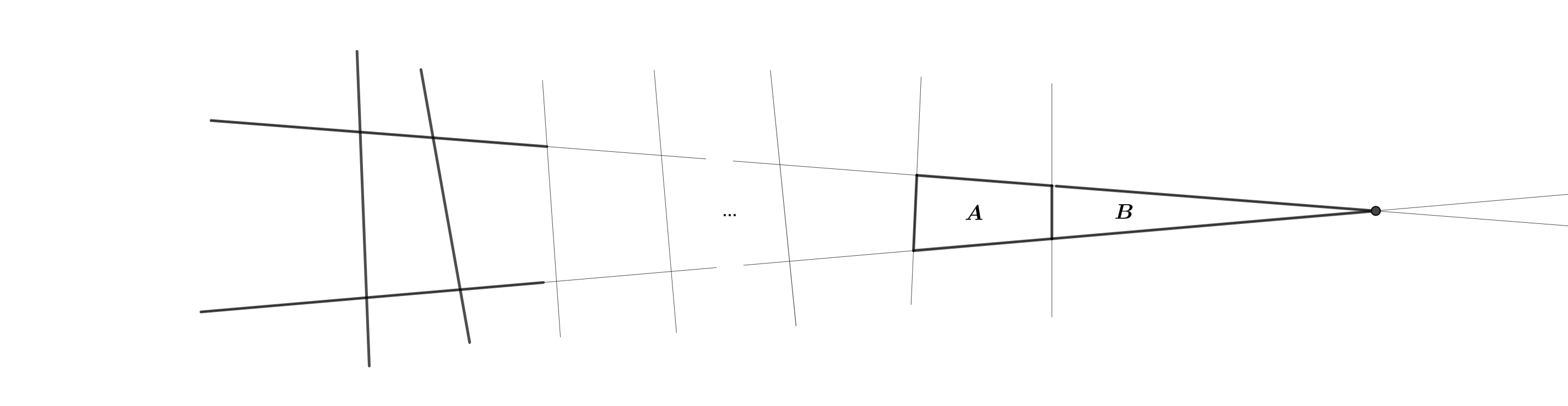
Next consider the other 2 edges of $A$. On a side where they don't converge there can only be a sequence of simple quadrilaterals (by the Lemma again), starting with $C$:
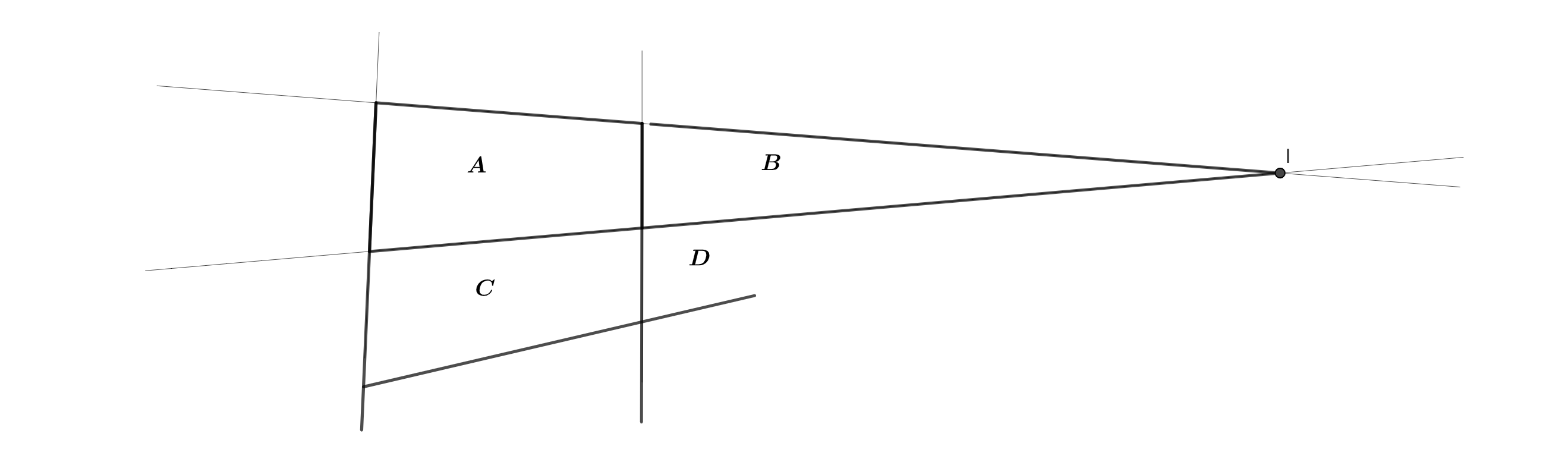
In order for the tile at $D$ to not cut any of $A, B, C$ and to comply with the Lemma, the only possibility is for it to be a triangle, with its incomplete edge reaching exactly I:
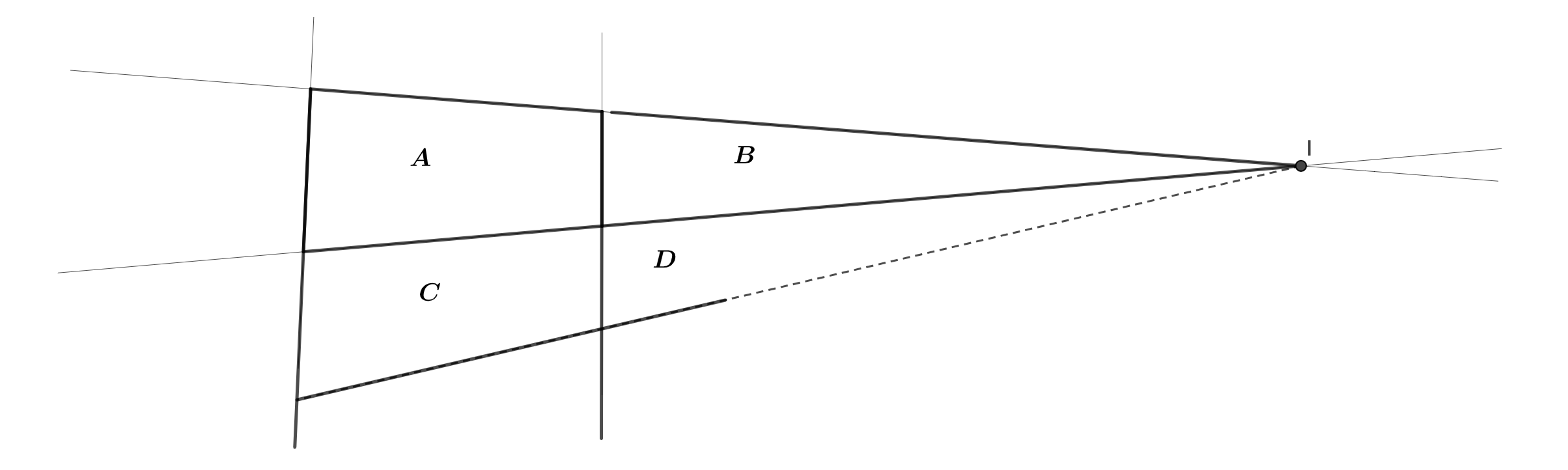
The same reasoning can be repeated with $E$ and $F$, and so on.
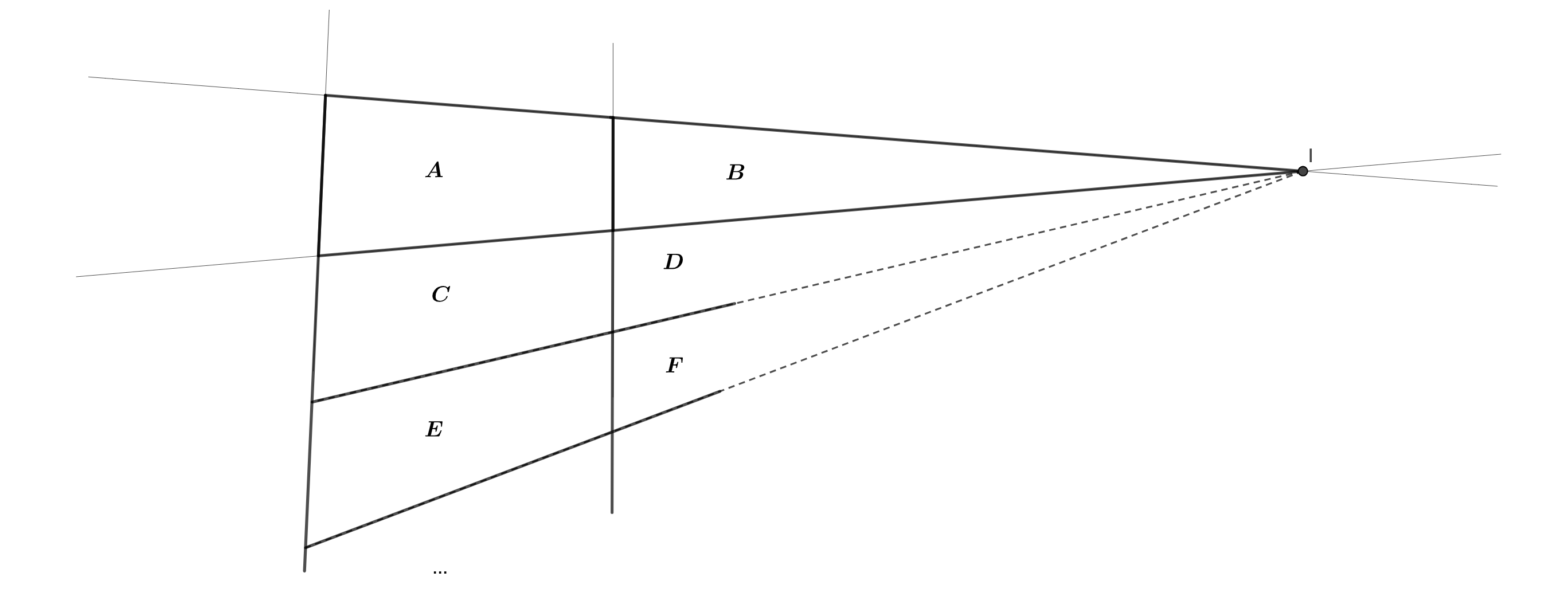
The point I must be then be the intersection of infinitely many lines, contradicting the definition of a fair cutting. This proves that any quadrilateral in a fair cutting must be a simple parallelogram, and from there it's trivial to see that all parallelograms must be identical in order to have the same area. Such cutting is equivalent to the square grid by affine transformation. $\quad\quad\blacksquare$
ORIGINAL ANSWER
This is a negative answer to Question 1.
COROLLARY: a convex $n$-agon has at most three ears with area $\ge$ interior area, and this is also optimal. (Hint: draw the cordchord spanning 2 consecutive ears, one $<$ and one $\ge$ interior area...)
- The critical step: reduce the smallest ear to a point by again rotating one edge around its endpoint, thereby increasing the two ears near the rotation point, and reducing the interior of the pentagon (to a quadrilateral).
This step may make another ear infinite, but that's easily fixed with a repeat of step 1):
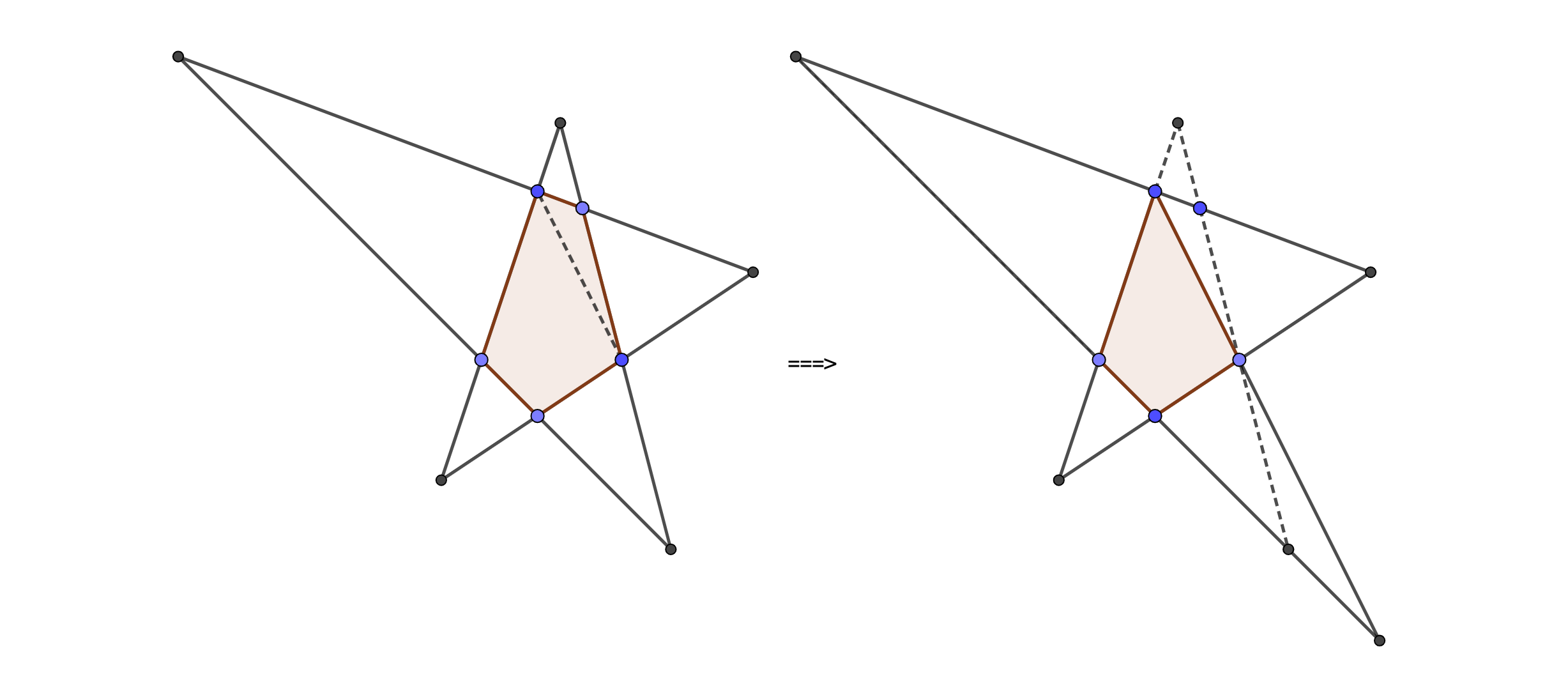 As a result of this step, it suffices now to prove that one of the new 4 non-degenerate ears has area less than the quadrilateral's.
As a result of this step, it suffices now to prove that one of the new 4 non-degenerate ears has area less than the quadrilateral's.
2) The critical step: reduce the *smallest* ear to a point by again rotating one edge around its endpoint, thereby increasing the two ears near the rotation point, and *strictly* reducing the interior of the pentagon to a quadrilateral.(If necessary, repeat step 1) to make all ears finite again.) As a result of this step, it suffices now to prove that one of the new 4 non-degenerate ears has area less than, or equal to, that the quadrilateral's.
As a result of this step, it suffices now to prove that one of the new 4 non-degenerate ears has area less than, or equal to, that the quadrilateral's.
Notice that $ a<b$ follows from $A_1<\infty$, and $c$ can be either $>a$, as in the figure, or $<-1$, or $\infty$.
Case $c<-1$:
$$\displaystyle ab\le 2, a\ge 1 \implies A_3=A_5\frac{b|c+1|}{(1+a)|c-b|}\le A_5\frac{ab}{(1+a)}\cdot \frac{|c+1|}{|c-b|}< A_5$$
and again done.
Case $c=\infty$:
$$\displaystyle ab\le 2, a\ge 1 \implies A_3=A_5\frac{b}{(1+a)}\le A_5\frac{ab}{(1+a)}\le A_5\quad \text{and}\quad A_4=A_5\frac{ab}{(1+a)}\le A_5$$
Here notice that the inequalities are not strict. In fact $A_1=A_2=A_3=A_4=A_5$ if and only if $a=1$, $b=2$. However this is sufficient since step 2) involved a strict decrease of the pentagon's area. $\quad\quad\blacksquare$






