An infinite countable family $\cal{L}$ of straight lines in the plane $\mathbb{R}^2$ forms a fair cutting of the plane if the following conditions are satisfied:
$\bullet$ No circle intersects infinitely many lines from the family $\cal{L}$;
$\bullet$ Let $L$ denote the union of the lines in $\cal{L}$. Each connected component of the complement of $L$ is a bounded set; the closure of each of these sets will be called a cell (observe that each cell is a convex polygon). This condition requires that the diameters of the cells formed by the cutting have a common upper bound;
Finally,
$\bullet$ All cells are of the same area.
We say that two fair cuttings are affinely equivalent if there exists an affine transformation of $\mathbb{R}^2$ sending the lines of one family onto the lines of the other. Also, we say that a fair cutting $\cal{L}$ is extra-fair, if, in at least one of its affine equivalents, all cells are congruent.
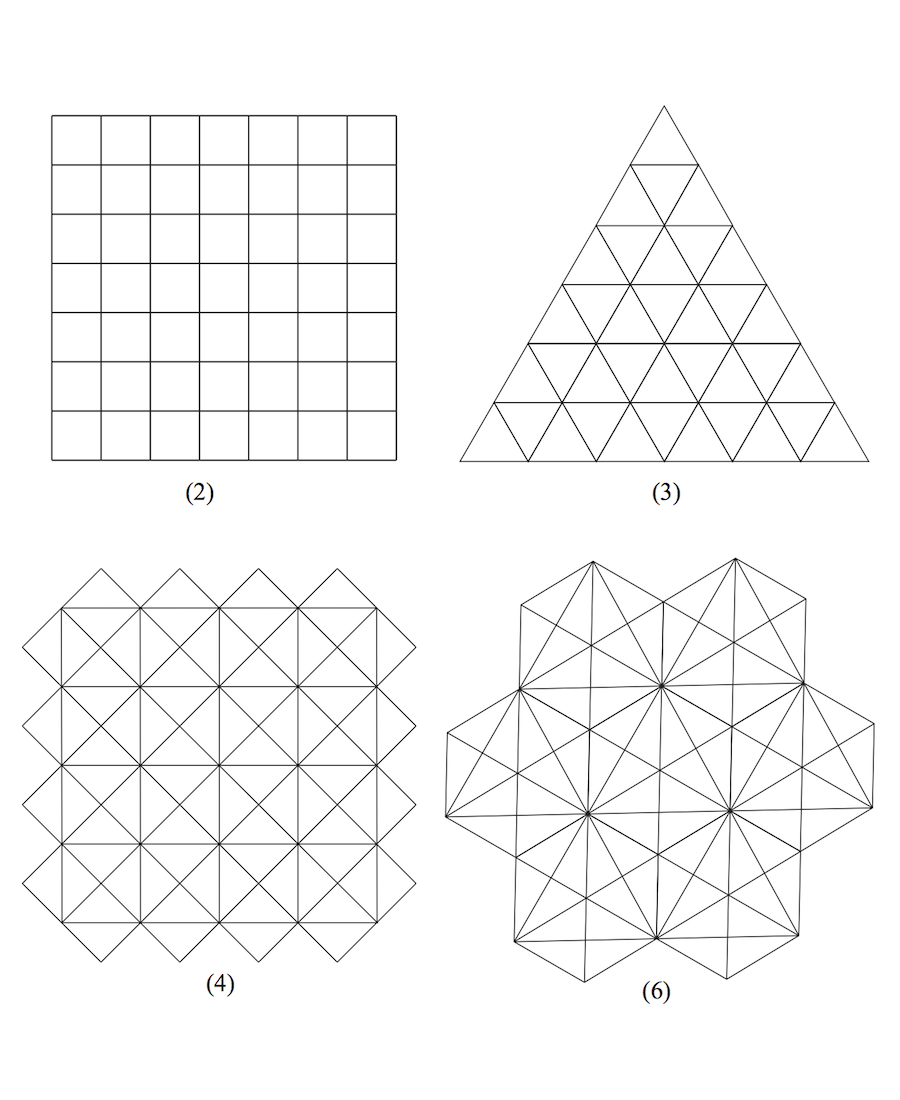
Four examples of mutually non-equivalent extra-fair cuttings are shown below.
Question 1. Is there a fair cutting whose at least one cell has more than four sides?
Question 2. Must all cells of a fair cutting have the same number of sides?
Question 3. Is every fair cutting extra-fair?
Question 4. Does there exist another example of a fair cuting, non-equivalent to any of the four pictured here?