Does this property characterize straight lines in the plane?

Question

Take a plane curve $\gamma$ and a disk of fixed radius whose center moves along $\gamma$. Suppose that $\gamma$ always cuts the disk in two simply connected regions of equal area. Is it true that $\gamma$ must be a straight line?

Answer 1

It seems $\gamma:\mathbb{R}\to\mathbb{R}^2$ (I assume $\gamma$ is injective and continuous) is indeed a line. My argument is very similar to the one by Ilkka Törmä (I thought I could write a shorter one, but it grew longer than expected). As in their answer, I will take the radius from the question to be $1$ and $D(p)$ will denote the disk of radius $1$ centered at $p$.

First suppose that $\gamma$ is bounded. Consider the point $p_M=(x_M,y_M)\in\overline{\gamma(\mathbb{R})}$, with $x_M$ being the maximal $x$ coordinate of all points of $\overline{\gamma(\mathbb{R})}$, and $y_M$ the maximal $y\in\mathbb{R}$ such that $(x_M,y)\in\overline{\gamma(\mathbb{R})}$. Then $D(p_M)\setminus\overline{\gamma(\mathbb{R})}$ has a connected component of area $>\frac{\pi}{2}$, and the same is true of $D(p)\setminus\overline{\gamma(\mathbb{R})}$ for points $p$ in $\gamma$ close to $p_M$, so $\gamma$ cannot be as in the question.

So we can assume that $\gamma$ is not bounded. This implies that for any $p\in\mathbb{R}^2$, $\gamma^{-1}(D(p))$ is a union of bounded intervals, and if $p\in\gamma(\mathbb{R})$, as explained in the other answer, $\gamma^{-1}(D(p))$ is just one bounded open interval, because if not $\gamma$ would divide $D(p)$ into more than $2$ connected components. Using this, it is not hard to prove by contradiction that $\gamma^{-1}(\overline{D(p)})$ is bounded for all $p\in\gamma(\mathbb{R})$.

Lemma 1: For any real numbers $a<b$, $\gamma([a,b])$ is contained in the convex closure of $X:=\gamma([a,b])\cap\left(\overline{D(\gamma(a))}\cup\overline{D(\gamma(b))}\right)$.

Proof: Note first that, for any point $p\in\gamma([a,b])\setminus X$, the interval $\gamma^{-1}(D(p))$ is strictly contained in $[a,b]$, so $D(p)$ only intersects $\gamma(\mathbb{R})$ in $\gamma([a,b])$. Also note that, as $X$ is compact, the convex closure of $X$ is the intersection of all closed half planes containing it.

So if the lemma was not true, there would be some closed half plane $H$ containing $X$ but not containing $\gamma([a,b])$. Composing with an isometry if necessary, we can suppose $H=\{(x,y)\in\mathbb{R}^2;x\leq0\}$. Now consider the point $p_M=(x_M,y_M)\in\gamma([a,b])$ given by $x_M=\max\{x\in\mathbb{R};(x,y)\in\gamma([a,b])\}$ and $y_M=\max\{y\in\mathbb{R};(x_M,y)\in\gamma(a,b)\}$. Then $D(p_M)\setminus\gamma([a,b])$ has a connected component of area $>\frac{\pi}{2}$, but $p_M\not\in H$, so $p_M\not\in X$, so $D(p_M)$ only intersects $\gamma(\mathbb{R})$ in some interval strictly contained in $\gamma([a,b])$. So $D(p_M)\setminus\gamma(\mathbb{R})$ has a connected component of area $>\frac{\pi}{2}$, a contradiction. $\square$

Lemma 2: $\gamma(\mathbb{R})$ is contained between two parallel lines $l,l'$.

Proof: For each $v\in\mathbb{S}^1$ let $R_v$ be the region between the two lines that have direction $v$ and are at distance $2$ of $\gamma(0)$; more precisely, $p\in R_v$ iff $d(\gamma(0),p)^2-\left(\langle v,p-\gamma(0)\rangle\right)^2\leq4$. Consider the directions $v_n=\frac{\gamma(n)-\gamma(-n)}{|\gamma(n)-\gamma(-n)|}$. Note that for all naturals $n$, by the previous lemma $\gamma(0)$ is in the convex hull of $\overline{D(\gamma(-n))}\cup\overline{D(\gamma(n))}$, so $\overline{D(\gamma(-n))}\cup\overline{D(\gamma(n))}$ is contained in $R_{v_n}$, so by the previous lemma again, $\gamma([-n,n])$ is contained in $R_{v_n}$. Then taking a limit point $v$ of the $v_n$, $\gamma(\mathbb{R})$ has to be contained in $R_v$: this is because for any $x\in\mathbb{R}$, $\gamma(x)$ is in $\bigcap_{n\geq|x|}R_{v_n}$, that is, $d(\gamma(0),\gamma(x))^2-\left(\langle v_n,\gamma(x)-\gamma(0)\rangle\right)^2\leq4$ for all $n\geq|x|$, so $d(\gamma(0),\gamma(x))^2-\left(\langle v,\gamma(x)-\gamma(0)\rangle\right)^2\leq4$, that is, $\gamma(x)$ is in $R_v$. $\square$

So, maybe after a rotation, we can suppose that $\gamma(\mathbb{R})$ is contained between two horizontal lines. Now, let $[a,b]$ be the minimal closed interval such that $\gamma$ is contained in the horizontal strip $\{a\leq y\leq b\}$

Lemma 3: There is some $p\in \gamma(\mathbb{R})$ with $y$ coordinate $a$.

If this lemma is true and $\gamma$ satisfies the question, then $\gamma(\mathbb{R})$ has to contain all the line $\{y=a\}$: if not, there would be some $p=(x,a)\in\gamma(\mathbb{R})$ such that the interval $[x-1,x+1]\times\{a\}$ is not contained in $\gamma(\mathbb{R})$, so $D(p)\setminus\gamma(\mathbb{R})$ would have a connected component of area $>\frac{\pi}{2}$, a contradiction.

Proof: We will prove it by contradiction, suppose the lemma is not true. By minimality of the interval $[a,b]$, there is a sequence of points $\gamma(t_n)=(x_n,y_n)$ of $\gamma(\mathbb{R})$ such that $y_n\to a$. The falsehood of lemma 3 implies that $|t_n|\to\infty$, and then, the facts that $|t_n|\to\infty$ and that $\gamma^{-1}(D(\gamma(x)))$ is bounded for all $x$ implies that $|x_n|\to\infty$ (if not, consider an accumulation point of $x_n$). Also note that $\gamma^{-1}\left(\overline{D(\gamma(0))}\right)$ is bounded, so $\gamma(\mathbb{R})\cap\overline{D(\gamma(0))}$ is contained in some rectangle $R=[c,d]\times[a+\varepsilon,b]$ for some $\varepsilon>0$. Now we will prove that $\gamma([0,\infty))\subseteq\{y\geq a+\delta\}$ for some $\delta>0$ by dividing in two cases; the same proof works for $\gamma((-\infty,0])$, contradicting the minimality of $[a,b]$.

• Case $1$: There are no sequences $x_n,y_n,t_n$ as in the paragraph above such that $t_n\to\infty$ (that is, we don't always have $t_n\to-\infty$). Then it is obvious that $\exists\delta>0$ such that $\gamma([0,\infty))\subseteq\{y\geq a+\delta\}$.

• Case $2$: There are sequences $t_n,x_n,y_n$ as above with $t_n\to\infty$. Then by lemma $1$, $\gamma([0,t_n])$ is contained for all $n$ in the convex closure of $\gamma([0,t_n])\cap\left(\overline{D(\gamma(0))}\cup\overline{D(\gamma(t_n))}\right)\subseteq R\cup\overline{D(\gamma(t_n))}$. Note that $R$ is a fixed rectangle with $y$-coordinates in $[a+\varepsilon,b]$, and $\overline{D(\gamma(t_n))}$ is a sequence of disks of radius $1$ centered at points $(x_n,y_n)$, where $y_n\in[a,b]$ $\forall n$ and $|x_n|\to\infty$. So any fixed point $p=(x,y)$ with $y<a+\varepsilon$ cannot be in the convex hull of $R\cup\overline{D(\gamma(t_n))}$ for big enough $n$: if that was the case (see $(*)$ below), then there would be a segment $s$ from some point $r\in R$ to some point $q\in \overline{D(\gamma(t_n))}$ passing through $p$, so the slope of the segments $qp$ and $pr$ would be the same. However, the slopes of segments from $p$ to points of $R$ are bounded below, while the slopes from $p$ to points of $\overline{D(\gamma(t_n))}$ converge to $0$ when $n\to\infty$, so for big enough $n$, the segment $s$ cannot exist. So any point $p=(x,y)\in\gamma([0,\infty))$ has to satisfy $y\geq a+\varepsilon$, as we wanted. $\square$

$(*)$ I have used that if $X,Y$ are convex subsets of $\mathbb{R}^2$, then any point $p$ in the convex closure of $X\cup Y$ is in a segment between a point of $X$ and a point of $Y$: indeed, as $p$ is in the convex hull of $X,Y$, there are points $x_i\in X,y_j\in Y$ and coefficients $a_i,b_j\geq0$ with $\sum a_i+\sum b_j=1$ such that $p=\sum_i a_ix_i+\sum_j b_jy_j$. Suppose $\sum a_i,\sum b_j>0$, if not $p$ is in $X$ or in $Y$. So $p$ is in fact an affine combination of the point $\sum_i \frac{a_i}{\sum_ka_k}x_i$ of $X$ and the point $\sum_j\frac{b_j}{\sum_kb_k}y_j$ of $Y$: $p=\left(\sum_ia_i\right)\left(\sum_i \frac{a_i}{\sum_ka_k}x_i\right)+\left(\sum_jb_j\right)\left(\sum_j\frac{b_j}{\sum_kb_k}y_j\right)$.

Answer 2

This is not a complete answer, but just some extended comments:

---

1. Existence of an inflection point inside every circle

Suppose that $\gamma$ is $C^2$ so that its curvature is everywhere well-defined. Then $\gamma$ must have an inflection point, i.e., a point of vanishing curvature, inside every one of these circles. If not, then the segment $S$ of $\gamma$ contained in one of the circles, say $C$, is locally convex. Since the end points of $S$ lie on $C$, it follows that $S$ is convex. Indeed, by gluing to $S$ one of the segments of $C$ determined by the end points of $S$, we obtain a simple closed locally convex curve. Every such curve must be globally convex, i.e., bound a convex set. So $S$ must lie strictly on one side of the tangent line of $\gamma$ passing through the center of $C$. Hence $S$ cannot bisect the area of the region bounded by $C$.

The above argument shows, in particular, that if the area bisecting property holds for circles of arbitrary small radius, then the curvature of $\gamma$ must vanish identically, which means that $\gamma$ has to be a line.

---

2. A generelized conjecture

It seems that a more general phenomenon could be true. Suppose that $\gamma$ divides the circles (of some fixed radius centered at points of $\gamma$) into regions of constant area. Then one might conjecture that $\gamma$ should be a circle. The radius of the circle is determined by the ratio of the areas of the two regions. When the two regions have equal area, then the radius is infinite or $\gamma$ is a line.

---

3. A local symmetry condition

If $\gamma$ is $C^1$, then one might try to prove the generalized conjecture proposed in item 2 above via a local symmetry condition as follows. Suppose that $\gamma$ passes through the origin $o$ of $R^2$ and is tangent to the $x$-axis at $o$. Further suppose that the circles have radii $1$. So $\gamma$ bisects the area of $S^1$. Let $A$ be the area of one of the regions, say $\Omega$, determined by $\gamma$ inside $S^1$. The rate of change of $A$, as the circle moves along $\gamma$, is determined by the segment $\Omega\cap S^1$. More specifically, the rate of change of $A$ depends on the portion of $\Omega\cap S^1$ in the right semicircle of $S^1$ versus the portion of $\Omega\cap S^1$ in the left semicircle of $S^1$. These portions must have equal length, since the rate of change of $A$ is zero. So if we let $p_+$ and $p_-$ be the intersection points of $\gamma$ with $S^1$, then $p_+$ and $p_-$ will be symmetric with respect to the $y$-axis.

More generally, for any point $p$ of $\gamma$ let $p_+$ and $p_-$ be the intersection of $\gamma$ with the circle centered at $p$. Then the normal line of $\gamma$ at $p$ must be orthogonal to and bisect the segment $p_+p_-$. I think that this condition might characterize circles, and it will force $\gamma$ to be a line when $p$, $p_+$, and $p_-$ are collinear.

Answer 3

Edit: the following argument is incomplete insufficient, since it doesn't prove that the direction of $S_x$ remains the same, or at least has vanishingly small perturbations, when $x$ goes to $\infty$. See the comments below.

Update (January 5th, 2023)

I (OP) take the liberty of updating again this answer after several months, since I realized that defining it "incomplete", as I did, is a bit misleading, letting perhaps one think that there's just some detail to be fixed. In fact, I think that the difficulty described in the Edit above is the key one. I arrived at versions of it through each one of the attempts I made at solving the problem.

---

I believe the implication is true for an arbitrary simple curve $\gamma$ whose both ends escape to infinity. First, let's rescale so that the discs have radius $1$. Denote by $D_r(\vec v)$ the open radius-$r$ disc centered at $\vec v$, where $r=1$ if it's omitted.

Lemma 1. For each $x$, $\gamma^{-1}(D(\gamma(x)))$ is an interval.

Proof. If not, then it has at least two connected components, whose $\gamma$-images split $D(\gamma(x))$ into at least three components. QED.

The following lemma does most of the work.

Lemma 2. Let $a < b < c$ be such that $\gamma(a), \gamma(c) \notin \bar D(\gamma(b))$, and let $\ell$ be a line passing through $\gamma(b)$. If $\gamma([a,c])$ is not contained in $\ell$, then it intersects both components of $\mathbb{R}^2 \setminus \ell$.

Proof. Suppose not, and let $A, B$ be the components with $\gamma([a,c])$ intersecting $B$. Re-orient the plane so that $\ell$ is horizontal. Let $x = \sup \{ t \leq b : \gamma(t) \in B \}$ or $x = \inf \{ t \geq b : \gamma(t) \in B \}$, whichever is finite and minimizes $\| \gamma(x) - \gamma(b) \|$; WLOG assume the latter and that $\gamma(x)$ is on the right of $\gamma(b)$ on $\ell$. Then there exist $t < x$ with $\gamma(t) \in B$ arbitrarily close to $x$. For $r > 0$, let $\ell_r$ be the length-$r$ closed segment of $\ell$ that extends to the right from $\gamma(x)$. Then $\gamma(\mathbb{R})$ cannot contain $\ell_r$ as a subset: since $\gamma$ is a homeomorphism onto its image, $\gamma^{-1}(\ell_r)$ would have to be connected, which contradicts the properties of $x$. If $\gamma(x) \in D(\gamma(b))$, then there exists $\vec v \in (\ell_r \cap D(\gamma(b))) \setminus \gamma(\mathbb{R})$, which has a neighborhood $U$ disjoint from $\gamma([a,b])$, and then $B \cup U$ is contained in one component of $D(\gamma(b))$ and covers more than half of it.

Suppose then $\gamma(x) \notin D(\gamma(b))$ and let $y = \inf \{ y \geq b : x \in D(\gamma(y)) \}$. Note that $S = \gamma(\mathbb{R}) \cap D(\gamma(y))$ is a line segment. If $\| \gamma(c) - \gamma(y) \| > 1$, then $c+\epsilon$ for a small $\epsilon$ works as $b$ above. Otherwise we must have $\gamma(c) \in B$, since $S = \gamma((t,x)) = \ell \cap D(\gamma(y))$ for some $t$ and $\gamma$ is simple. The minimal $d > c$ with $\gamma(d) \in \ell$, if it exists, satisfies $\| \gamma(d) - \gamma(x) \| > 0$, so we have $\gamma(x) \in D(\gamma(y+\epsilon))$ and $\gamma(d) \notin D(\gamma(y+\epsilon))$ for some small $\epsilon > 0$. Then $y+\epsilon$ and $d$ work as $b$ and $c$ above. QED.

In the following $\ell(\vec v, \vec w)$ is the line that passes through $\vec v \neq \vec w$. We say a set $K \subset \mathbb{R}$ is between two parallel lines $\ell$ and $\ell'$, if $K$ is contained in the convex hull of $\ell \cup \ell'$.

Lemma 3. There exist two parallel lines such that $\gamma(\mathbb{R})$ is between them.

Proof. For $x > 0$, consider $C_x = \gamma([-x,x])$. Since $C_x$ is compact, it is between two lines $\ell_x^-, \ell_x^+$ that are parallel to $\ell_x = \ell(\gamma(-x), \gamma(x))$ and have minimal distance among such pairs of lines. Then both lines intersect $C_x$.

We claim that the distance between $\ell_x^-$ and $\ell_x^+$ is at most $2$. If not, one of them, say $\ell_x^+$, has distance $d > 1$ from $\ell_x$. Take any $\gamma(b) \in \ell_x^+ \cap C_x$, which has distance at least $d$ from $\gamma(-x)$ and $\gamma(x)$, since the latter points are in $\ell_x$. Then the numbers $-x < b < x$ and the line $\ell_x^+$ contradict Lemma 2.

We claim that as $x \to \infty$, the direction of the line $\ell_x$ converges to some direction $\delta$. For all $0 < x < y$ we have $\gamma(-x), \gamma(x) \in C_y$, and hence the segment $S_x = \gamma(-x) \gamma(x)$ of $\ell_x$ is between $\ell_y^-$ and $\ell_y^+$. Then every point of $S_x$ is within distance $2$ of $\ell_y$, which constrains the direction of $\ell_y$ to an interval whose length approaches $0$ as the length $\| \gamma(-x) - \gamma(x) \|$ of $S_x$ grows. The intersection of these intervals is a singleton $\{\delta\}$.

Let $\ell^-, \ell^+$ be the two lines with direction $\delta$ that are at distance $3$ from $\gamma(0)$. We claim that $\gamma(\mathbb{R})$ is between these lines. Fix $x > 0$, and consider $r > 0$. For all large enough $y$, the set $D_r(\gamma(0)) \cap (\ell_y^- \cup \ell_y^+)$ is between $\ell^-$ and $\ell^+$ since the directions of $\ell_y^-, \ell_y^+$ converge to $\delta$ and they are at distance at most $2$ from $\gamma(0)$. Since $C_x$ is between $\ell_y^-$ and $\ell_y^+$, if we chose $r$ large enough then this shows that $C_x$ is also between $\ell^-$ and $\ell^+$. Since this holds for all $x$, we are done. QED.

We now fix two parallel lines $\ell^-, \ell^+$ that have $\gamma(\mathbb{R})$ between them and whose distance is minimal.

Lemma 4. $\ell^- = \ell^+$.

Proof. Suppose not. Both lines contain points that are arbitrarily close to $\gamma(\mathbb{R})$ since their distance is minimal. If either line intersects $\gamma(\mathbb{R})$, then the intersection point $\gamma(b)$ and the points $\gamma(-x), \gamma(x)$ for any large enough $x > 0$ contradict Lemma 2. Rotate the plane so the lines are horizontal with $\ell^+$ on top, and suppose WLOG $\gamma(x)$ escapes to the right when $x \to +\infty$ and gets arbitrarily close to $\ell^+$ as it does so. Consider $C_a = \gamma([-a,a])$ for some $a$ with $\gamma(\mathbb{R}) \cap ([-2,2] \times \mathbb{R}) \subset C_a$ and let $\epsilon > 0$ be its minimum distance from $\ell^+$. Take $b, c$ with $\gamma(b)_1 > 2$, $\gamma(c)_1 \gg 4 \cdot \gamma(b)_1$ and $\gamma(b)$ closer to $\ell^+$ than $\epsilon/2$. Consider the minimum-slope line $\ell$ that intersects $\ell^+$ directly above $\gamma(c)$ and intersects $C_a$. The vertical distance from $\gamma(b)$ to $\ell$ is at least $\epsilon/4$, and so is the vertical distance between $\ell$ and the lowest parallel line $\ell'$ that lies above $\gamma([-a,c])$. Let $\gamma(d)$ be an intersection point of $\ell'$ and $\gamma([-a,c])$. Then $\gamma(0), \gamma(c) \notin \bar{D} (\gamma(d))$ (the first because $\ell'$ is disjoint from $C_a$, the second because it intersects $\ell^+$ far to the left of $\gamma(c)$), so that $\ell'$ violates Lemma 2 with $0$, $d$ and $c$. QED.

Now $\gamma$ is the line $\ell^- = \ell^+$.

Answer 4

This is not an answer, but some thoughts using variational calculus. Since the property of dividing a disk in half is somehow non-local, I'm not sure that variational calculus alone will solve your conjecture, but it's not a bad place to start.

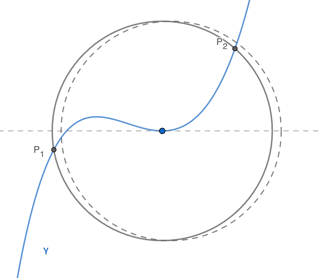
When $\gamma$ is smooth enough (say $C^2$), we can compute the first and second variations of the areas on either side of $\gamma$ as we translate along the curve. This will give geometric equations which constrain the geometry of $\gamma$. We can rescale the problem so that the radius of the circle is 1, that $\gamma(0)=0$ and that $\gamma^\prime(0) = 0$. Suppose that the curve $\gamma$ intersects the circle $S^1$ at points $P_1 = e^{i \theta_1} $ and $P_2= e^{i \theta_2}$, as shown below.

In order for the first variation of the difference of the areas to vanish, we must have that $$\int_{\theta_1}^{\theta_2} \cos(\theta) \, d \theta = \int_{\theta_2}^{2 \pi +\theta_1} \cos(\theta) \, d \theta. $$

Put more simply, this forces $\sin(\theta_1)= \sin(\theta_2)$. This is essentially @Mohammed Ghomi's symmetry argument, which shows that the intersections are symmetric with respect to the y-axis.

When $\gamma$ is not a straight line, since it cannot lie entirely on one side of the x-axis, this forces there to be at least two inflection points within the disk, one in between $P_1$ and the origin and the other in between the origin and $P_2$.

I suspect that the second variation will give a relationship between $\gamma^{\prime \prime}$ at the origin and $\gamma^\prime$ at $P_1$ and $P_2$. The hope would be that it is possible to leverage this into stronger geometric control on $\gamma$. Perhaps when $\gamma$ is assumed to be real analytic, we can use induction on the variations to show that it must be a line segment. However, at present I don't really know how to use this.

Answer 5

I add some further thoughts here, as they will probably take more room than allowed in a comment.

Let us call $\mathcal{P}$ the original problem.

Consider the variation of the problem, which I'll call $\mathcal{V}$, in which you ask that the intersections of the curve $\gamma$ with the circle are always exactly two, and determine two arcs of equal length. This version was considered by M. Winter and Pietro Majer in the comments. As they pointed out, the graph of any $r$-periodic function $f$ (where $r$ is the radius of the circle) is a solution of $\mathcal{V}$ as soon as $f$ does not oscillate too much, so that its graph intersects the circle always in exactly two points.

Consider then the family of problems $\mathcal{P}_t$, corresponding to the original, area version, but this time calculating the area of the two regions after removing from the original disc a concentric disc of radius $t\in [0,r)$. We have $\mathcal{P}=\mathcal{P}_0$. It is reasonable (isn't it?) to think that for $t$ small enough, the solution(s) of the problem $\mathcal{P}_t$ have to be "close" to the solutions of $\mathcal{P}$. On the other hand, when $t$ approaches $r$ the problem becomes "close", in some sense, to $\mathcal{V}$, for which we know that uncountably many solutions exist. So, if the answer to the original question is "yes", i.e. if only a straight line divides a disc that way, there should be a critical value $t_k$ such that for every $t\ge t_k$ the family of solutions starts to have cardinality larger than 1. Obviously this critical value may well be $t_k=0$ or $t_k=r$ (I tend to believe the latter is the case, because when $t=r$ the very concept of measure involved changes).