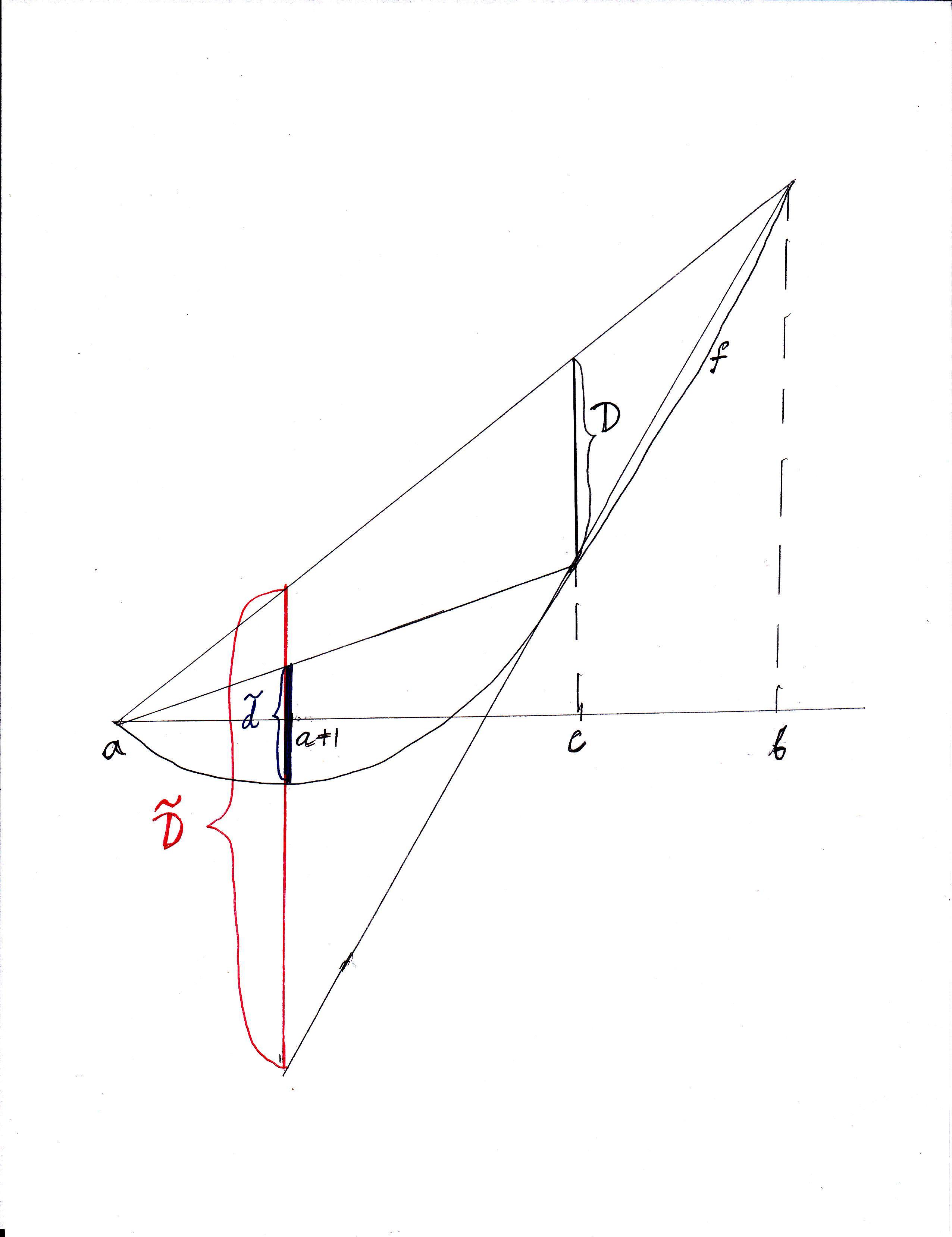
First let's reformulate the question. For $0\leq a\leq c\leq b$, let $\lambda=\lambda(a,c,b)\in[0,1]$ be the number such that $c=\lambda a + (1-\lambda)b$, and for $f\colon \mathbb R_+\to\mathbb R$ define
$$
D_f(a,c,b)= \lambda f(a)+(1-\lambda)f(b)-f(c).
$$
Lemma 1. Let $f\colon \mathbb R_+\to\mathbb R$ be strictly convex and continuously differentiable. Let $0\leq a_n\leq c_n\leq b_n$ be sequences such that $a_n$ is bounded, $c_n-a_n$ is bounded away from 0, and $\limsup \lambda(a_n,c_n,b_n)>0$. Then, $\limsup D_f(a_n,c_n,b_n)>0$.
We first apply a sequence of reduction steps that allow us to assume wlog that $a_n=0$, $c_n\geq 1$, , for all $n$, and $\liminf\lambda(a_n,c_n,b_n)>0$. If you trust that that is possible, you may skip directly to Lemma 5 below.
By taking a sub-sequence $n'$ on which $\liminf \lambda(a_{n'},c_{n'},b_{n'})>0$, Lemma 1 follows from Lemma 2.
Lemma 2. Let $f\colon \mathbb R_+\to\mathbb R$ be strictly convex and continuously differentiable. Let $0\leq a_n\leq c_n\leq b_n$ be sequences such that $a_n$ is bounded, $c_n-a_n$ is bounded away from 0, and $\liminf \lambda(a_n,c_n,b_n)>0$. Then, $\limsup D_f(a_n,c_n,b_n)>0$.
By further taking a sub-sequence $n'$ on which both $a_{n'}$ converges, Lemma 2 follows from Lemma 3.
Lemma 3. Let $f\colon \mathbb R_+\to\mathbb R$ be strictly convex and continuously differentiable. Let $0\leq a_n\leq c_n\leq b_n$ be sequences such that $a_n\to a$, $c_n-a_n$ is bounded away from 0, and $\liminf \lambda(a_n,c_n,b_n)>0$. Then, $\limsup D_f(a_n,c_n,b_n)>0$.
For any fixed $\epsilon>0$, the functions $\lambda(a,c,b)$ is continuous in $a$ uniformly in $c$ and $b$ over the domain $\epsilon\leq a +\epsilon\leq c\leq b$; therefore, under the assumptions of Lemma 3, $0<\liminf\lambda(a_n,c_n,b_n)=\liminf\lambda(\lim a_n,c_n,b_n)$. Furthermore, for $\lim a_n <a<\liminf c_n$ small enough, we have $\liminf\lambda(a,c_n,b_n)>0$. Since $D_f(a,c,b)$ is decreasing in $a$, $\limsup D_f(a_n,c_n,b_n)\geq\limsup D_f(a,c_n,b_n)$.
Therefore, Lemma 3 follows from Lemma 4.
Lemma 4. Let $f\colon \mathbb R_+\to\mathbb R$ be strictly convex and continuously differentiable. Let $0\leq a\leq c_n\leq b_n$ be sequences such that $c_n-a$ is bounded away from 0, and $\liminf \lambda(a,c_n,b_n)>0$. Then, $\limsup D_f(a,c_n,b_n)>0$.
Let $T\colon \mathbb R\to\mathbb R$ be the affine transformation that maps $a$ to $0$ and $\inf c_n$ to $1$. Replacing $f$ by $F=f\circ T^{-1}$, and $a,c_n,b_n$ by $T(a),T(c_n),T(b_n)$ respectively, Lemma 4 follows from Lemma 5.
Lemma 5. Let $F\colon \mathbb R_+\to\mathbb R$ be strictly convex and continuously differentiable. Let $1\leq c_n\leq b_n$ be sequences such that $\liminf \lambda(0,c_n,b_n)>0$. Then, $\limsup D_F(0,c_n,b_n)>0$.
Proof of Lemma 5.
We assume wlog that $F(0)=0$ and denote $\lambda_n=\lambda(0,c_n,b_n)$ and $D_n=D_F(0,c_n,b_n)$.
Define a function $G\colon [1,\infty)\times (1,\infty)\to \mathbb R$ by
$$
G(x,y)=\tfrac 1 y F(xy)-F(x).
$$
Claim 6. $G$ is positive and increasing in both $x$ and $y$.
Proof of Claim 6.
Since $F$ is strictly convex, $F(0)=0$, and $x = 1/y(xy)+(1-1/y)0$, $G(xy)>0$. Since $F'$ is increasing, we have $\frac {d}{dx}G(xy)=F'(xy)-F'(x)>0$, so $G$ increases in $x$. Since $F'$ is increasing and $G(x,y)=1/y\int_0^yF'(xt)x\,dt - F(x)$, $G$ increases in $y$, completing the proof of Claim 6.
Suppose there is $\lambda_0>0$ such that $\lambda_n\geq \lambda_0$ for all $n$. Then,
$$
D_n = G(c_n,1/(1-\lambda_n))\geq G(1,1/(1-\lambda_0)>0, \quad\text{for all $n$.}
$$
QED