Does the sum $$ \lim_{n\to\infty}\sum_{k=0}^{\lfloor\alpha n \rfloor}C_n^k(-1)^k\left(1-\frac{k}{\alpha n}\right) $$ converge, where $C_n^k$ is the binomial coefficient and $0 <\alpha <1$?
The above question has been solved by Iosif Pinelis. A variation is $$ \lim_{n\to \infty}\sum_{k=0}^{\lfloor\alpha n \rfloor}C_n^k(-1)^k\left(1-\frac{k}{\alpha n}\right)^n. $$ How can we handle this sum?
$\newcommand\an{\lfloor a n \rfloor}$ Let $a:=\alpha\in(0,1)$. 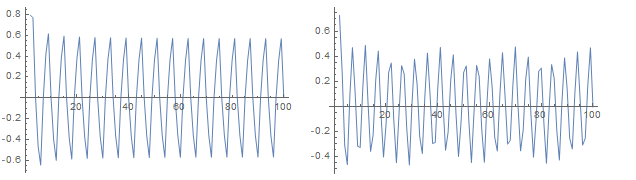By induction on $m=0,1,\dots$, $$\sum_{k=0}^m \binom nk(-1)^k\Big(1-\frac k{a n}\Big) \\ =(-1)^{m+1} (a+m-a n)\frac{m+1}{an (n-1)}\,\binom n{m+1}.$$ So, letting $S_n$ denote the sum in question, we have $$S_n\sim(-1)^{\lfloor a n \rfloor+1}(a-\{a n\}) \,M_n,$$ where $\{a n\}$ is the fractional part of $a n$ and $$M_n:=\frac1n\,\binom n{\an+1}.$$ Let now $n\to\infty$. Depending on the arithmetical properties of $a$, the factor $(-1)^{\lfloor a n \rfloor+1}$ will alternate between $1$ and $-1$ and the factor $a-\{na\}$ will oscillate between $a-1<0$ and $a>0$, whereas $M_n\to\infty$, since eventually, for all large enough $n$, we have $\binom n{\an+1}\ge\min[\binom n2,\binom n{n-2}]=n(n-1)/2$. So, the sum $S_n$ will not converge to any limit.
For an illustration, here are the connected graphs $\{(n,c_a^n n^{3/2}\,S_n)\colon n=1,\dots,100\}$ for $a=1/3$ (left) and $a=\sqrt2-1$ (right), where $c_a:=a^a (1 - a)^{1 - a}\in(0,1)$:
