8
$\begingroup$

For a closed plane curve $C$, define its sequence of winding numbers to be the sorted list of the winding numbers of each of the distinct regions of the plane demarcated by $C$. For example, this curve (if I've calculated correctly) has sequence $001111223 = 0^2 1^4 2^2 3$.

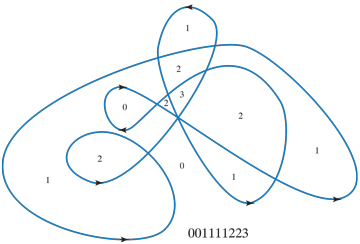
          WindingNumbers
A winding number sequence must include $0$ for the unbounded region of the plane. I am wondering if there are any other restrictions:

Q. Can any winding sequence of consecutive integers that includes $0$ be realized by some curve $C$?

Improve this question
$\endgroup$
3
  • $\begingroup$ Maybe a silly question, but why do the numbers need to be consecutive? You can go around any loop more than once. (Perhaps you are just imposing consecutiveness for simplicity?) $\endgroup$
    – Zach Teitler
    Commented Dec 31, 2017 at 23:39
  • 2
    $\begingroup$ @ZachTeitler: "the winding numbers for any two adjacent regions differ by exactly 1; the region with the larger winding number appears on the left side of the curve." This from Wikipedia. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 31, 2017 at 23:52
  • 2
    $\begingroup$ Ah, the curve is forbidden from retracing itself. Okay. $\endgroup$
    – Zach Teitler
    Commented Jan 1, 2018 at 0:25

1 Answer 1

Reset to default
22
$\begingroup$

Isn't this easy by induction? Delete one of the largest numbers, say $m$, from your sequence, realize the remaining numbers, then in the realization pick any region with winding number $m-1$, and make an extra loop somewhere close to its boundary.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Simple, clean, and convincing! $\endgroup$
    – Joseph O'Rourke
    Commented Dec 31, 2017 at 21:22
  • 1
    $\begingroup$ Also works for the signed version of the question by looping either clockwise or counter-. $\endgroup$
    – Noam D. Elkies
    Commented Dec 31, 2017 at 21:40
  • 3
    $\begingroup$ Naively, how about for a higher-dimensional version, such as in mathoverflow.net/questions/259054/… ? $\endgroup$
    – Zach Teitler
    Commented Dec 31, 2017 at 23:13
  • 2
    $\begingroup$ @ZachTeitler: Thanks, Zach, that's where I was heading next... Perhaps a future question. $\endgroup$
    – Joseph O'Rourke
    Commented Dec 31, 2017 at 23:53
  • 2
    $\begingroup$ I don't see why that would require a different solution, you can just do the same thing. $\endgroup$
    – domotorp
    Commented Jan 1, 2018 at 11:46

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .