Planar linkage that traces a circle from its exterior?

Question

Q. Is there a linkage in the plane that traces out a circle $C$ in such a manner that the interior of the disk bounded by $C$ is never intersected by any link througout the motion?

What I mean by "a linkage in the plane" is best illustrated by the famous Peaucellier linkage, which traces out a straight line segment: _{(Wikipedia image.)}
         
Some vertices are "pinned to the plane." All vertex joints are universal in the sense of allowing full $360^\circ$ motion. All links are rigid segments, which can pass over one another in a physical, layered model, e.g.,
         
          _{(Image from this How Round Is Your Circle web page.)}

---

Of course it is trivial without the restriction that the links not intersect the interior of $C$: One radial link pinned to the center of $C$ suffices. And the challenge in the Peaucellier linkage was to convert the natural circular motions of linkage components into straight-line motion. Here I am seeking a vertex of the linkage to follow a natural circular motion, but with the restriction to not intersect the interior of that circle $C$. Following a subarc of $C$—say, a semicircle—without intersecting the interior of $C$, would also be quite interesting.

It may be that the 19th-century masters (Peaucellier, Lipkin, Watt, Chebyshev, et al.) did not investigate this question. But perhaps there is an easy construction I am not seeing...?

---

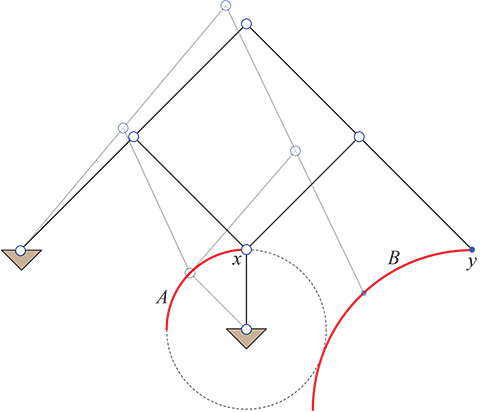
Here is a version of TMA's idea. The pantograph shown scales by $\times 2$: As joint $x$ traces arc $A$, endpoint $y$ traces arc $B$, from the exterior of $B$'s disk:
         

Answer 1

I don't have a facility with graphics; hopefully the verbal description below will work.

There is a linkage that magnifies: place a tracing stylus at point P, a marking stylus at point Q, and this linkage M will allow one to trace a figure and replicate it with a growth factor of (say) 3.

Now take M and attach it to a pivoting link with the free end fixed to P in such a way that Q traces an arc. One can get at least 120 degrees of arc this way, and arrange it so the arc is convex from the side of the combined linkage. For a full circle, a link arm has to be transported around a point and be rotated at least 180 degrees during this journey. I am confident a linkage can be designed, but have no ideas for it at present.