A necessary and sufficient condition for three diagonals of a hexagon to be concurrent

Question

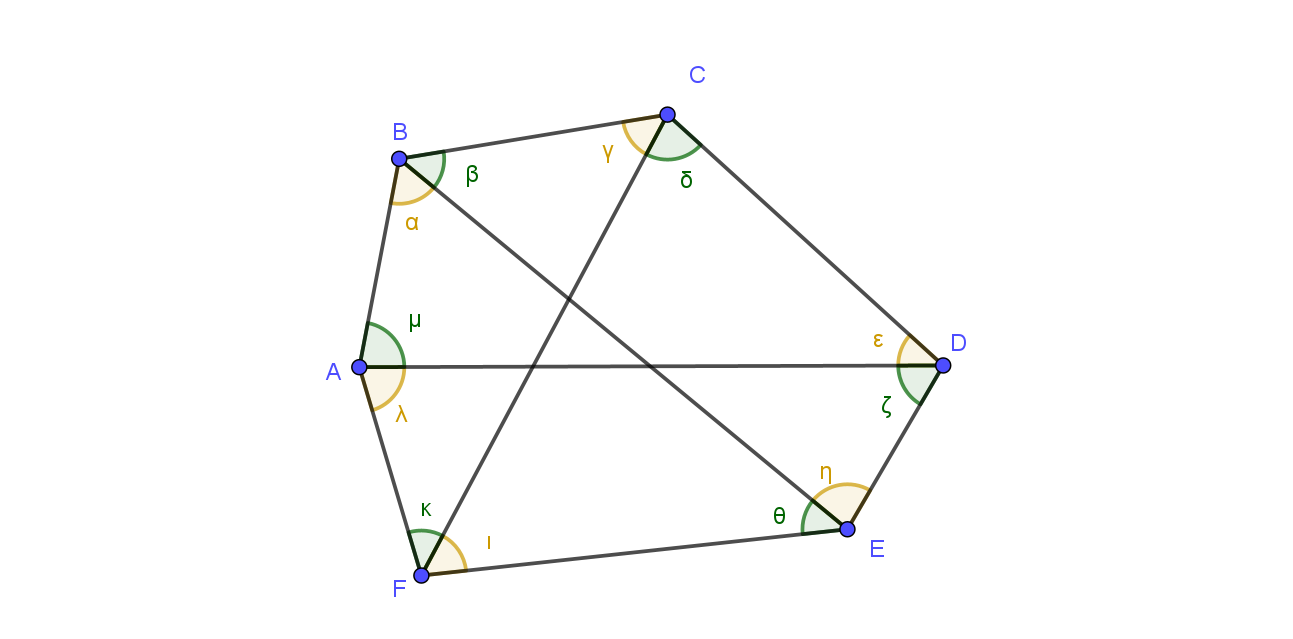
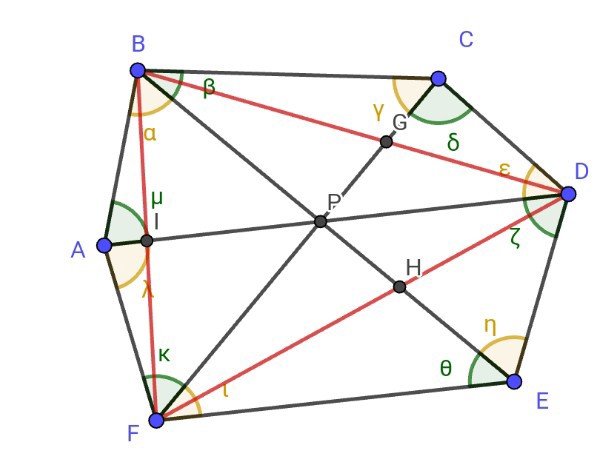
When talking about the condition for the three diagonals of a hexagon to be concurrent, we will think of Brianchon's theorem. Using software, I discovered a necessary and sufficient trigonometric relation for the three diagonals to be concurrent, similar to Cava's sine theorem. But I have never seen it anywhere.

Consider $ABCDEF$ be a hexagon, then $AD, BE, CF$ are concurrent if only if

$$\frac{\sin(α)} {\sin(β)} \frac{\sin(γ)} {\sin(δ)}\frac{\sin(ε)}{\sin(ζ)}\frac{\sin(η)}{\sin(θ)}\frac{\sin(ι)}{\sin(κ)}\frac{\sin(λ)}{\sin(μ)}=1$$

Question: Have you seen this result anywhere?

Answer 1

Ok here is the comment with a figure.

First consider the identity where $C$ is on $(BD)$, $A$ on $(BF)$ and $E$ on $(FD)$. This reads for example as $$\frac{\sin(α')} {\sin(β')} \frac{\sin(γ')} {\sin(\pi-\gamma')}\frac{\sin(ε')}{\sin(ζ')}\frac{\sin(η')}{\sin(\pi-η')}\frac{\sin(ι')}{\sin(κ')}\frac{\sin(λ')}{\sin(\pi-\lambda')}=1$$ Then applying sine rule $$\frac{\sin{(κ')}}{BG}=\frac{\sin{(\gamma')}}{BF}$$ also $$\frac{\sin{(ι')}}{GD}=\frac{\sin{(\pi-\gamma')}}{FD}$$ So $$\frac{\sin(ι')}{\sin(κ')}=\dfrac{DG}{GB}\dfrac{BF}{FD};$$ likewise for the other angles the product simplifies to one by Ceva theorem. The diagonals intersect at $P$. When $C$ moves from $G$ on $(FP)$ the ratio remains one by the sine rule also as $$\dfrac{\sin{(\beta')}}{\sin{(\epsilon')}}=\dfrac{\sin{(\beta)}\sin{(\delta)}}{\sin{(\epsilon)}\sin{(\gamma)}}$$ From $\frac{\sin{(\beta')}}{PD}=\frac{\sin{(\epsilon')}}{BP}$, $\frac{\sin{(\gamma)}}{BP}=\frac{\sin{(\beta)}}{PC}$, and $\frac{\sin{(\epsilon)}}{PC}=\frac{\sin{(\delta)}}{PD}$.

Answer 2

One can state the original conjecture in terms of loci as follows: fix the first five vertices and denote the sixth by $P=(x,y)$. Consider the two loci

1. those $P$ for which the concurrency holds;

2. those $P$ for which the sine condition holds.

The conjecture that these two loci coincide is, as shown above, false--the first locus is a proper subset of the second. One can make this result more precise as follows: the first locus is a straight line (the one through $A_3$ and the intersection of $A_1A_4$ and $A_2A_5$). The second is the union of this line and a conic section.

The proof is computational. One calculates the area of the triangle carved out by the three main diagonals (it is a linear form in $x,y$) and the difference of the two sine products (after simplification, this is a quartic). The latter quartic is the product of the square of the above linear form and a quadratic form. The required conic is the zero set of the latter.