I'm trying to determine the nature of this series $ \sum_{n \geq 1} \frac{ \cos(n) \sin(n+1) }{n} $, but I'm not getting anywhere. I've tried using the Abel and trigonometric formulas, but I can't find it. Does anyone have any ideas? Or even a solution? Please let me know. Thank you very much and good evening.
$\begingroup$
$\endgroup$
6
-
3$\begingroup$ Average value of $\cos(x)\sin(x+1)$ is $\sin(1)/2 \approx 0.42\ldots \neq 0$, so the sum should diverge. I think it wouldn't be too hard to prove using some sort of equidistribution (and, of course, irrationality of $\pi$), but I'll leave it to someone else. $\endgroup$– Aleksei KulikovCommented Nov 9, 2023 at 18:55
-
12$\begingroup$ Indeed, $\cos(n) \sin(n+1) = \frac{\sin(2n+1)}{2} + \frac{\sin(1)}{2}$. The sum of a convergent series and a divergent series is divergent. $\endgroup$– Robert IsraelCommented Nov 9, 2023 at 19:14
-
$\begingroup$ Thanks, I forgot my trigonomical formulas. $\endgroup$– user516435Commented Nov 9, 2023 at 20:57
-
$\begingroup$ @Aleksei Irrationality of $\pi$ is not needed, by the way, only $1/\pi \notin \mathbb{Z} $ $\endgroup$– Fedor PetrovCommented Nov 9, 2023 at 21:19
-
1$\begingroup$ @IosifPinelis A bit more general series $\sum \frac{\cos a n \sin(an+b)}n$ (in our situation $a=b=1$) is convergent if and only if $b/\pi \notin \mathbb{Z}$. $\endgroup$– Fedor PetrovCommented Nov 10, 2023 at 5:39
|
Show 1 more comment
1 Answer
$\begingroup$
 $\endgroup$
$\endgroup$
3
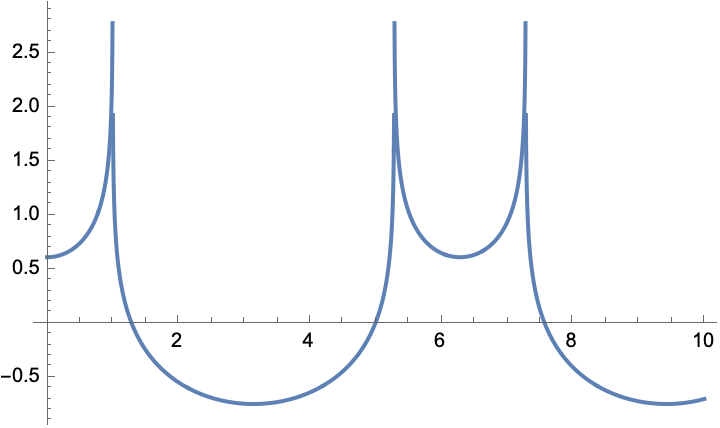
You might try to regularize the sum, $$S(\alpha)=\sum_{n=1}^\infty \frac{ \cos(\alpha n) \sin(n+1) }{n}= -\tfrac{1}{4} i \left[e^{-i} \ln \left(1-e^{i (\alpha-1)}\right)+e^{-i} \ln \left(1-e^{-i (\alpha+1)}\right)-e^{ i} \ln \left(1-e^{-i (\alpha-1)}\right)-e^{ i}\ln \left(1-e^{i (\alpha+1)}\right)\right]$$ $$=\text{Re}\, \biggl(\tfrac{1}{2} i e^i \ln \left[2 e^i (\cos 1-\cos \alpha)\right]\biggr),\;\;\alpha\neq\pm 1\;\;\text{mod}\;(2\pi).$$
Here is a plot of $S(\alpha)$.

-
$\begingroup$ Do you have a proof of this result? $\endgroup$ Commented Nov 9, 2023 at 21:34
-
$\begingroup$ it's a geometric series, $\sum_{m=0}^{ \infty} {z^n/ n}=-\ln(1-z)$ for any $z$ on the unit circle unequal to 1, see for example math.stackexchange.com/questions/118686/… $\endgroup$ Commented Nov 9, 2023 at 22:25
-
$\begingroup$ No, this is not a geometric series. $\endgroup$ Commented Nov 10, 2023 at 4:53
