An application of ping-pong lemma

Question

Let $F_2$ be free group of rank two with generators $a$ and $b$. If $H$ is a subgroup of $F_2$ generated by $d\geq 2$ elements with $$H=\langle a,b^{-k}ab^k, k=1,2,...,d-1\rangle,$$ I was trying to use ping-pong lemma to show that $H$ is a free group of rank $d$. I was looking at the action of $H$ on $F_2$ via conjugation and trying to find $d$ disjoints subsets $X_1,X_2,\dots, X_d$ of $F_2$ such that if we set $g_1=a, g_k=b^{-k}ab^k, k=1,2,\dots, d-1$ and show that $g_jX_ig_j^{-1}\subseteq X_j.$ I am doing it by hit and trial but nothing seems to be working even for $d=2$.

Answer 1

As another version of HJRW’s answer: free groups are (isomorphic to) fundamental groups of graphs. The rank is then captured by the number of “independent” loops in the graph. For example $F_2$ is isomorphic to the fundamental group of the rose with two petals. Now use the correspondence between subgroups and covering spaces.

Answer 2

Fact. In a free product $A\ast B$, the groups $aBa^{-1}$ for $a\in A$ generate their free product. In other words, the homomorphism $j:B^{\ast A}\to A\ast B$ mapping $b$ in the $a$-th copy to $aba^{-1}$, is injective.

One proof only uses the universal property of free products (already used to defined $j$). Indeed, let $A$ act in $B^{\ast A}$ by shifting and consider the semidirect product (free version of the wreath product) $A\ltimes B^{\ast A}$.

Then since $j$ is $A$-equivariant, it extends to a homomorphism $J:A\ltimes B^{\ast A}\to A\ast B$, mapping $A$ identically to the free factor $A$.

Also, by the free product universal property, there is a unique homomorphism $p:A\ast B\to A\ltimes B^{\ast A}$ which is the "identity" map on both factor ($B$ mapping onto the copy indexed by $1_A$). Then one directly sees that $p\circ J=\mathrm{id}$. So $J$ is injective. In particular $j$ is injective, which is what we had to prove. [Of course $J$ is surjective too, so $p=J^{-1}$.]

(This makes this simpler, than, for instance, the fact that subgroups of free groups are free, for which the simplest proofs are topological.)

Answer 3

(This is an elaboration of HJRW’s comments on the question and on Sam Nead’s answer.)

This can be seen using the representation of free groups as fundamental groups of graphs, and subgroups as immersions of graphs, as developed in the very readable paper Topology of finite graphs (Stallings, 1983, Inventiones, doi:10.1007/BF02095993). Only a few facts are needed.

Fact 1. The fundamental group $\pi_1(G,x)$ of a graph $G$ at a vertex $x$ can be represented as the reduced cycles at $x$ (i.e. paths from $x$ to itself not containing any adjacent pair of an edge and its inverse).

Fact 2. This fundamental group is free on the “non-trivial cycles”. Explicitly, given a maximal tree $T$ in $G$, a free basis of $\pi_1(G,x)$ is given by the cycles $x \to_T y \overset{e}{\to} z \to_T x$ for each edge $e$ not in $T$, where $x \to_T y$ denotes the unique path from $x$ to $y$ in $T$.

Definition. A graph homomorphism $f : G \to H$ is an immersion if it is injective on the star of each vertex of $G$ (i.e. the set of half-edges incident to that vertex).

Fact 3. An immersion induces an injection on fundamental groups.

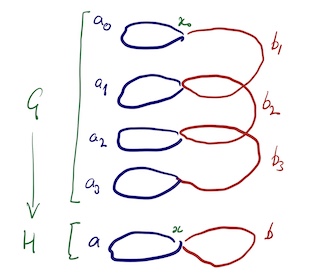
Now we can draw a picture:

Stallings graph immersion

This is evidently an immersion $f : G \to H$. Fact 2 above says that $\pi_1(H,x)$ is free on the two generators $\{a,b\}$, while $\pi_1(G,x_0)$ is free on the four generators $\{a_0,\, b_1^{-1}a_1 b_1,\, \ldots,\, b_1^{-1}b_2^{-1}b_3^{-1}a_3 b_3 b_2 b_1\}$. Those generators map $f$ to the cycles $b^{-n}ab$ for $n = 0,1,2,3$, so the image of $f$ is the subgroup generated by these elements; so by Fact 3, that subgroup of $\langle a, b \rangle$ is free. All this easily generalises to the case with more generators.

Stallings’ notion of core graphs, and the associated folding algorithm, explain how to find representations like this in general — given an explicit finitely-generated subgroup of a free group, the folding algorithm produces a presentation of it as an immersion from a finite graph, and hence read off a free basis.

Answer 4

While there have been many good proofs suggested in answers or comments, that are definitely the right way to do this for more general subgroups, this particular example is sufficiently straightforward that an elementary argument seems easiest. Put $c_i=b^{-i}ab^i$ for $i\leq 0\leq d-1$. Then $c_0,\ldots, c_{d-1}$ generate your subgroup. I claim by induction on length that if $w$ is a nontrivial reduced word in the free group on $d$ generators $x_0,\ldots, x_{d-1}$, then evaluating that word on $c_0,\ldots, c_{d-1}$ is nontrivial. More precisely, I claim that if the last letter of $w$ is $x_i^{\epsilon}$ with $\epsilon=\pm 1$, then $w(c_0,\ldots, c_{d-1})$ ends in $a^{\epsilon}b^{i}$.

This is clear if $w$ is a single letter. Otherwise, by induction $w=ux_i^{\epsilon}$ where $u$ is reduced. Since the last letter of $u$ is not $x_i^{-\epsilon}$, we deduce by induction that $u(c_0,\ldots, c_{d-1})$ ends in $a^{\pm 1}b^{j}$ with $j\neq i$ unless the last letter is $x_i^{\epsilon}$, in which case the reduced form ends in $a^{\epsilon}b^i$. Therefore, when we multiply $u(c_0,\ldots, c_{d-1})$ by $c_i^{\epsilon}$, we either get something ending in $b^{j-i}a^{\epsilon}b^i$ with $j\neq i$ or the reduced form of $u(c_0,\ldots, c_{d_1})c_i^\epsilon$ will end in $a^{\epsilon }a^{\epsilon}b^{i}$ as required.

Answer 5

Here is a proof which relies on a straighforward generalization of the Ping Pong Lemma.

Claim 1 Let $a$ and $b$ be the transformations of the Riemann sphere $\hat{\mathbb{C}} = \mathbb{C} \cup \{\infty\}$ defined by $a(z) = \frac{1}{z} + 2,\quad b(z) = z + 4$. Let $d \in \mathbb{N}_{> 0} \cup \{\infty\}$. Then the set $\{b^{k}ab^{-k} \,\vert\, 0 \le k < d\}$ is the basis of a free subgroup of $\operatorname{PGL}(2,\mathbb{Z})$.

Claim 1 and its proof are a trivial adaptation of [1, Example II.B.26] which makes use of Schottky groups.

Proof of Claim 1. Let $\mathbb{D} = \{ z \in \mathbb{C} \, \vert \, \vert z \vert \le 1\}$, $\Omega_k = (\mathbb{D} + 4k) \cup (\mathbb{D} + 4k + 2)$ for $0 \le k < d$. Observe that $\gamma_k = b^ka b^{-k}$ maps

• the exterior of the disk $\mathbb{D} + 4k$ onto the interior of the disk $\mathbb{D} + 4k + 2$, and
• the interior of the disk $\mathbb{D} + 4k$ onto the exterior of the disk $\mathbb{D} + 4k + 2$.

Thus $\gamma_k^m (\Omega_l) \subseteq \Omega_k$ for every $k \neq l$ and every $m \in \mathbb{Z} \setminus \{0\}$. Clearly $\Omega_k \nsubseteq \Omega_l$ if $k \neq l$. A straightforward generalization of the Table-Tennis Lemma yields the result.

Here is another way to answer OP's question by means of a generalized Table-Tennis Lemma. The following claim is a geometric abstraction wrapping the solution that HJRW has outlined in comments.

Claim 2 [1, Solution of Exercise II.b.36.i]. Let $T$ be a tree and let $\gamma_1, \dots,\gamma_d$ be hyperbolic automorphisms of $T$ with pairwise disjoint axes. Then the subgroup of $\operatorname{Aut}(T)$ generated by $\gamma_1, \dots, \gamma_d$ is free on these elements.

An automorphism $\gamma$ of a tree $T$ is hyperbolic if it acts on $T$ without inversion, i.e, it doesn't swap the ends of an edge of $T$, and if $\tau(\gamma) = \min_{x \in V(T)} {\bf d}(x, \gamma(x)) > 0$ where $\bf{d}$ is the usual combinatorial distance on $T$ (i.e., the reduced edge-path length) and $V(T)$ denotes the set of vertices of $T$. If $\gamma$ is hyperbolic, then the vertices $x$ of $T$ satisfying ${\bf d}(x, \gamma(x)) = \tau(\gamma)$ are the vertices of a subgraph of $T$ which is a geodesic line called the axis of $\gamma$. We denote this line by $\operatorname{axis}(\gamma)$.

Proof of Claim 2. Re-indexing the elements $\gamma_i$ if need be, we can find $x_i \in \operatorname{axis}(\gamma_i)$ for every $i \in \{1, \dots, d\}$ such that the geodesic segment joining $\gamma_1$ to $\gamma_d$ passes through the vertices $x_1, \dots, x_d$, in this order. Let $\Omega_i$ be the set of vertices of $T$ such that the geodesic segment joining $x$ to $x_i$ passes through the $\gamma_i(x_i)$ or $\gamma_i^{-1}(x_i)$. It is not difficult to check that $\gamma_i^m(\Omega_j) \subseteq \Omega_i$ for every $m \in \mathbb{Z} \setminus{0}$ and $\Omega_i \nsubseteq \Omega_j$, provided that $i \neq j$. Thus the generalized Table-Tennis Lemma applies.

Let us observe that Claim 2 also answers OP's question. Indeed, multiplying the elements of $F_2$ on the left by $\gamma_i = b^i a b^{-i}$ defines a hyperbolic automorphism of the Cayley graph of $F_2$. As $V(\operatorname{axis}(\gamma_i)) = \{b^i a^k \, \vert \, k \in \mathbb{Z}\}$, Claim 2 applies.

---

[1] P. de la Harpe, "Topics in Geometric Group Theory", 2000.

Answer 6

Here is a direct approach using the ping-pong lemma as OP originally requests, which has been gestured at in several comments but not yet offered as an answer.

Consider the action of $F = \langle a,b\rangle$ on itself by left multiplication. Let $X_k \subseteq F$ be the set of elements which start with $b^ka$ or $b^ka^{-1}$, in their reduced word representation. As the ping-pong lemma requires, these are disjoint; and for $j \neq k$ and $n \neq 0$, we can see that $(b^k a b^{-k})^n X_j \subseteq X_k$ by the standard multiplication of reduced words. The claim follows.

Under the hood this is essentially the same proof as Benjamin Steinberg’s answer, but the ping-pong lemma helps organise the required analysis of reduced words.