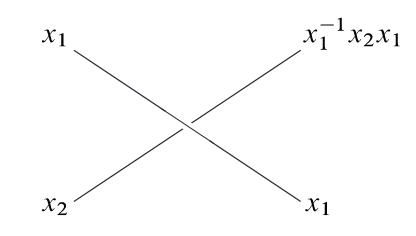
Suppose I have a knot $K$ in $S^3$. Given a diagram $D$ of $K$ I get the Wirtinger presentation $\langle x_1, \dots, x_a \mid r_1, \dots, r_c\rangle$ of its knot group $\pi(K) = \pi_1(S^3 \setminus K)$. There is a generator $x_i$ for each arc of $D$ and a relation $r_j$ for each crossing of $D$. (Arc means over-arc: an arc starts at an under-crossing and continues through over-crossings until the next under-crossing.) The generators represent meridians of $K$ and the relations all say that the generators of the two under-arcs at a crossing are conjugate via the over-arc.
Suppose $x_1$ and $x_2$ are two arcs related at a crossing as below:
and that $x_1$ and $x_2$ correspond to distinct arcs (if I follow the knot from $x_1$ to $x_2$ I travel under at least one crossing). Is it possible for $x_1$ and $x_2$ to commute? More formally, is there a knot $K$ for which the map $$\phi : \pi(K) \to \pi(K)/\langle x_1 x_2 x_1^{-1} x_2^{-1}\rangle$$ is injective?
I'm interested in this question in the context of hyperbolic knots, so a more specific question is if there's a hyperbolic knot $K$ for which $\phi$ is injective.