In Theorem 1 of this paper Segal stablish a relation betwenbetween states and generatingenerating functionals. He assert that in order to $\mu$ be a generating functional must satisfy
Then $$ \sum_{j,k\in F} \mu (z_j-z_k)e^{iB(z_j\cdot z_k)}\bar{\alpha}_k\alpha_j\ge 0 $$ Then, as an example he show the functional
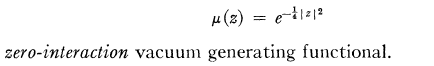 $$
\mu(z)= e^{-\frac{1}{4}|z|^2}
$$
is the zero-interaction vacuum generating functional.
$$
\mu(z)= e^{-\frac{1}{4}|z|^2}
$$
is the zero-interaction vacuum generating functional.
The question is.The question is: Why this functional satisfies the desidered condition?

