I've tagged this as reference request as surely this question must be very well investigated, I just don't know how to look for it. Most likely the perfect answer will be in form of a keyword for googling :)
I would like to know about dynamics of relative frequencies of prime factorizations. More precisely, let $[k_1,k_2,...]$ be any multiset of natural numbers, and for $x>0$ let $f_x[k_1,k_2,...]$ be the number of those natural $n\le x$ with prime factorization of the form $p_1^{k_1}p_2^{k_2}\cdots$, divided by $x$. How does the "champion" (i. e. $[k_1,k_2,...]$ with largest $f_x[k_1,k_2,...]$) change with $x$? Do all "champions" have some common features? (Say, are they all of the form $[1,1,...]$?) At which $x$es do "champion changes" occur?
More generally, how does the list of all multisets $[k_1,k_2,...]$ ordered according to $f_x[k_1,k_2,...]$ vary with $x$?
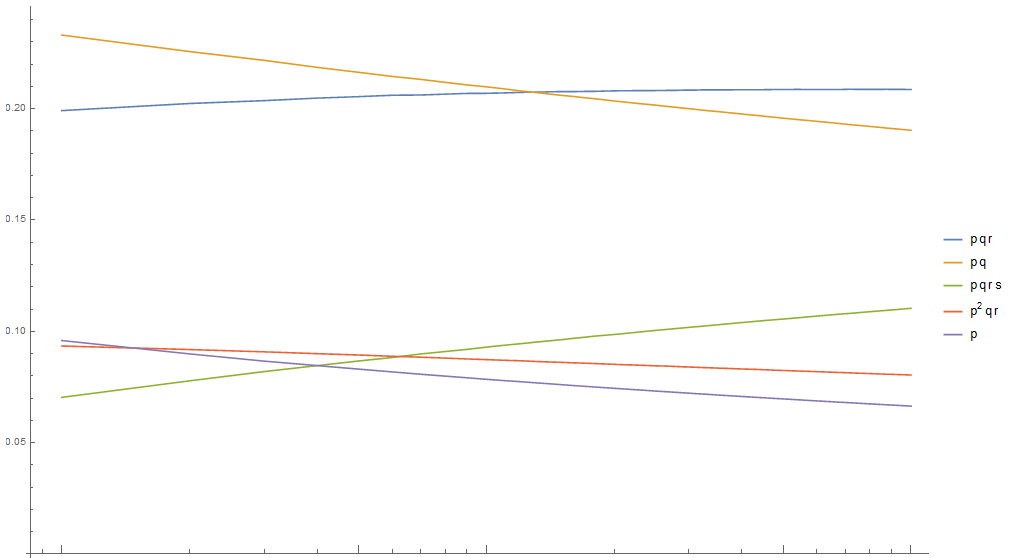
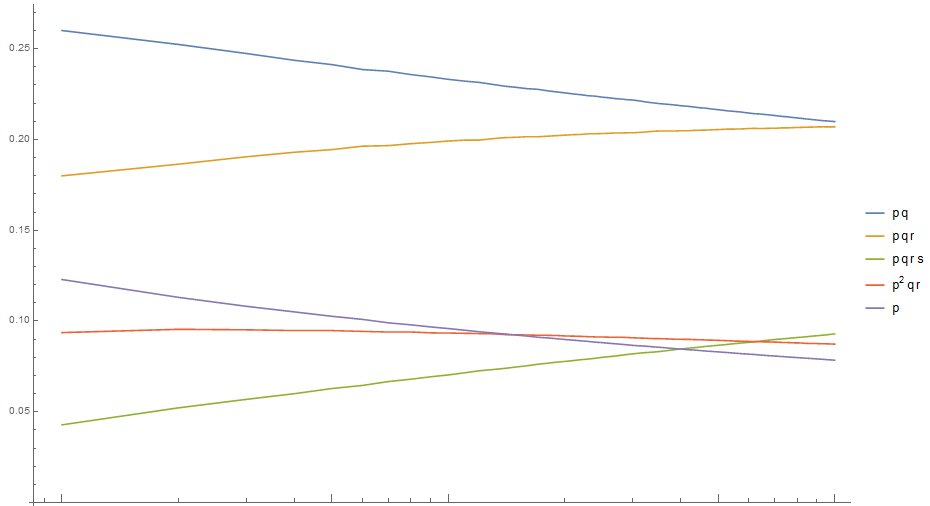
Here is the plot of $f_x$ for $x$ from $100000$ to up to ten million of the $f_x[k_1,k_2,...]$ for five most frequent (at ten million) $[k_1,k_2,...]$, namely, for $[1,1,1]$, $[1,1]$, $[1,1,1,1]$, $[1,1,2]$ and $[1]$.