Suppose the points of two random walks in $\mathbb{R}^2$ are given the
step number (or time) as a third coordinate, so that they become paths in $\mathbb{R}^3$.
Here are several pairs of walks of $n=100$ steps, both starting at the origin,
with steps normally distributed with $\sigma=1$:
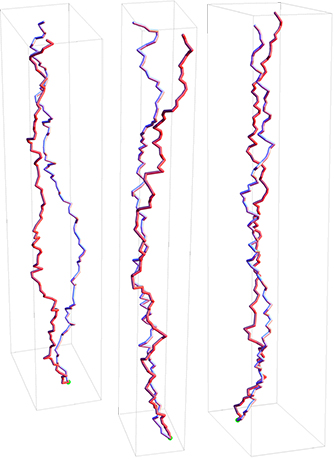
I would like to know the expected number of times that one path winds about the other,
as a function of $n$, the number of steps.
I believe for the three pairs illustrated, there is zero winding by $n=100$.
Experiments indicate winding becomes less likely as $n$ grows.
This is a bit counterintuitive to me.
The winding number of path $b(t)$ about path $a(t)$ up to $t=T$ could be defined by counting the number of times (the normalization of) the vector $b(t)-a(t)$ turns around the origin for $t\in[0,T]$.
