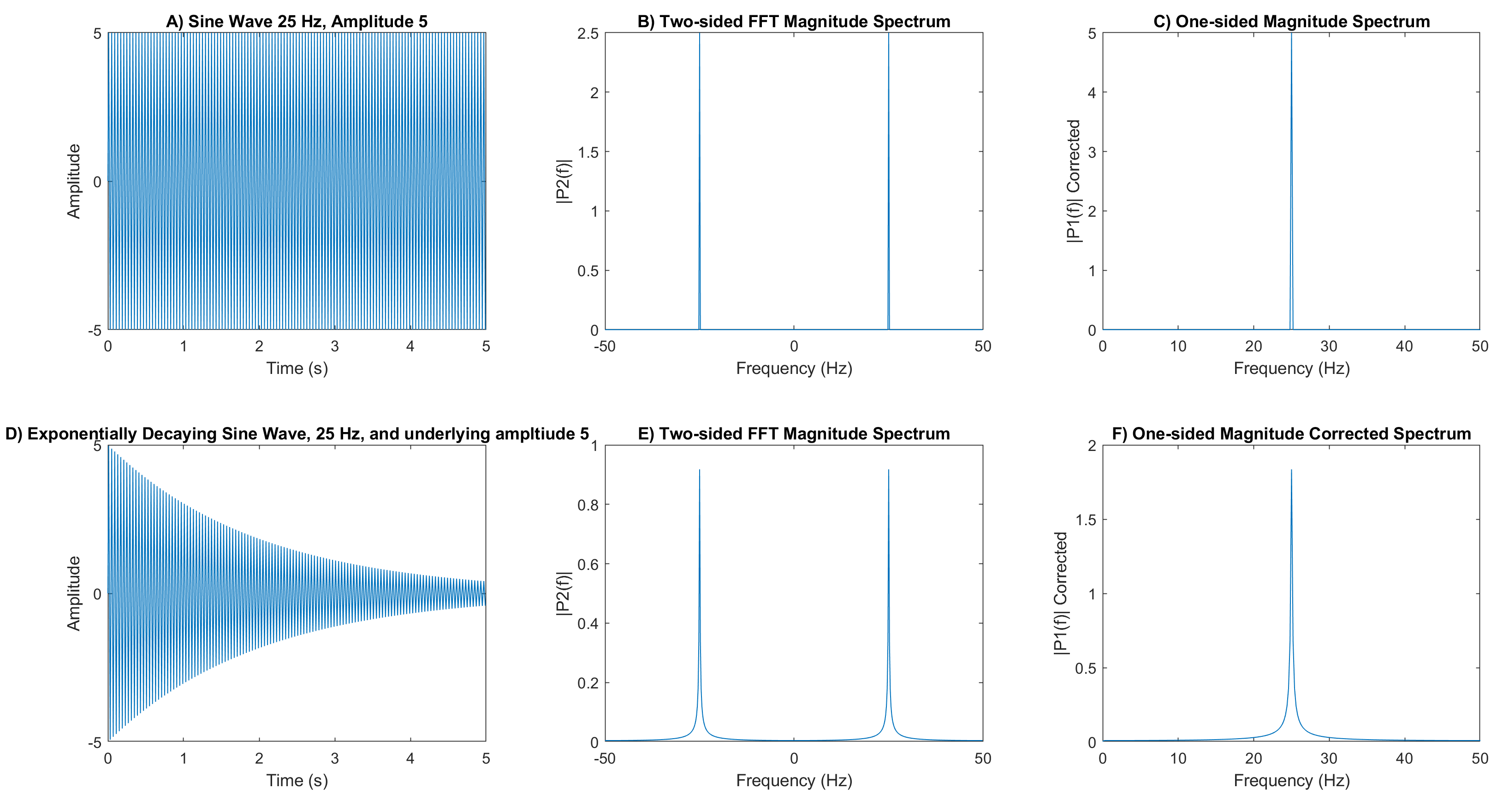
In nuclear magnetic resonance experiments, the raw data is an exponentially decaying sinuoid(s) as a function of time (t $\geq$ 0). In order to see the frequency spectrum the standard protocol is to do a Fast Fourier Transform (FFT) and display one-sided frequency spectrum 1.

a) Simple scenario: We have a non-decaying sine wave of frequency $\omega_0$ and amplitude $A$. FFT of this signal is two peaks (one corresponding to positive frequency and the other one at negative frequency situated at $\omega_0$ and amplitude $A/2$. Note that we have to normalize the FFT with the number of sampled points in order to get the right amplitudes in the frequency spectrum.

b) Real experimental scernaio: Let us say we have an exponentially decaying sine wave $x(t)$, then,

$$x(t) = A \sin(\omega_0 t) e^{-\alpha t} \, u(t)$$

where $u(t)$ is the Heaviside unit step function, $\alpha$ is positive and real. Now the key question is that in (a), we were able to predict the amplitude of the peaks in the frequency spectrum. It was $A/2$. In this exponentially decaying case, is there a way to predict the amplitude of the resulting peak in frequency spectrum?

An annotated figure will clarify the question. Starting from (a) a sine wave with 25 Hz and amplitude of 5 will show up as a single peak in FFT spectrum of amplitude 5/2 (two sided version) or 5 in the one-sided spectrum.

Now the same sinusoid is multiplied by an exponential with $\alpha$= 0.5. We get a much less peak height in the frequency spectrum. In short, is there a way to predict this peak height if we know the decay constant and initial amplitude of the sine wave in FFT. Thanks.