_{Simplified repost of Are these continued fractions of integrals known? on MSE}

EDIT: The period of the oscillations of $$f(s)=\dfrac1{1+\dfrac s{1+\dfrac{s^2/2!}{1+\dfrac{s^3/3!}{1+\cdots}}}}$$ appears to converge to $$\dfrac4e=1.4715\cdots$$ as @user42355 commented. Can this be proven?

Empirically, the argmins are

2.12897, 3.73409, 5.26940, 6.78494, 8.29119, 9.79168, 11.2881, 12.7814, 14.2723,
15.7613, 17.2487, 18.7348, 20.2198, 21.7038, 23.1870, 24.6695, 26.1513, 27.6326,
29.1133, 30.5937, 32.0736, 33.5531, 35.0323, 36.5111, 37.9897, 39.4680, 40.9461,
42.4239, 43.9015, 45.3790, 46.8562, 48.3332, 49.8101, 51.2869, 52.7635, 54.2399,
55.7162, 57.1924, 58.6685, 60.1445, 61.6203, 63.0961, 64.5717, 66.0473, 67.5228, ...

with consecutive differences (note insufficient precision beyond fourth decimal)

         1.60512, 1.53531, 1.51554, 1.50625, 1.50049, 1.49642, 1.49330, 1.49090, 
1.48900, 1.48740, 1.48610, 1.48500, 1.48400, 1.48320, 1.48250, 1.48180, 1.48130, 
1.48070, 1.48040, 1.47990, 1.47950, 1.47920, 1.47880, 1.47860, 1.47830, 1.47810, 
1.47780, 1.47760, 1.47750, 1.47720, 1.47700, 1.47690, 1.47680, 1.47660, 1.47640, 
1.47630, 1.47620, 1.47610, 1.47600, 1.47580, 1.47580, 1.47560, 1.47560, 1.47550, ...

Define the continued fraction integral transform $$\{\mathcal If(t)\}(s)=\dfrac{f(s)}{1+\dfrac{\int_0^s f(x)\,dx}{1+\dfrac{\int_0^s\int_0^u f(x)\,dx\,du}{1+\cdots}}}$$ where $f\in C^\infty(\Bbb R)$. Has this been studied?

Elementary properties include $\{\mathcal I(0)\}(s)=0$, $\{\mathcal If\}(0)=f(0)$ and $1+\{\mathcal If\}(s)=f'(s)/\{\mathcal If'\}(s)$ for all non-constant $f$ such that $f(0)=0$.

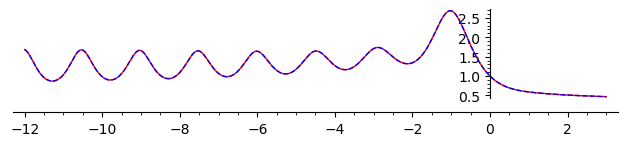
When $f(t)\equiv1$, the function $\{\mathcal I(1)\}(s)$, mentioned in A319173 with no further information, has the following graph

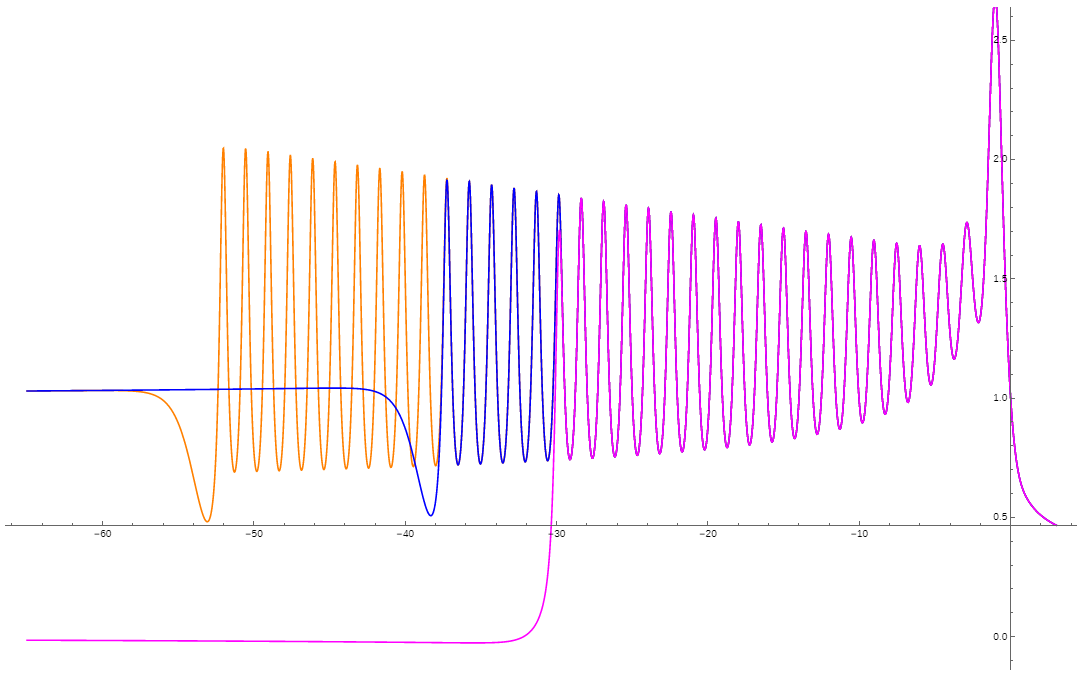
Zooming out (orange with 140 terms; blue with 100 terms; magenta with 79 terms), we observe excellent convergence, until the plots branch off to either 0 or 1 depending on the parity of the number of terms.

How do we explain the regular oscillating behaviour over the negative reals?