_{Simplified repost of Are these continued fractions of integrals known? on MSE}

Define the continued fraction integral transform $$\{\mathcal If(t)\}(s)=\dfrac{f(s)}{1+\dfrac{\int_0^s f(x)\,dx}{1+\dfrac{\int_0^s\int_0^u f(x)\,dx\,du}{1+\cdots}}}$$ where $f\in C^\infty(\Bbb R)$. Has this been studied?

Elementary properties include $\{\mathcal I(0)\}(s)=0$, $\{\mathcal If\}(0)=f(0)$ and $1+\{\mathcal If\}(s)=f'(s)/\{\mathcal If'\}(s)$ for all non-constant $f$ such that $f(0)=0$.

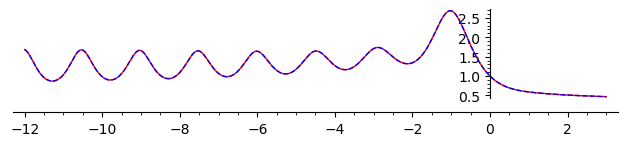
When $f(t)\equiv1$, the function $\{\mathcal I(1)\}(s)$, mentioned in A319173 with no further information, has the following graph

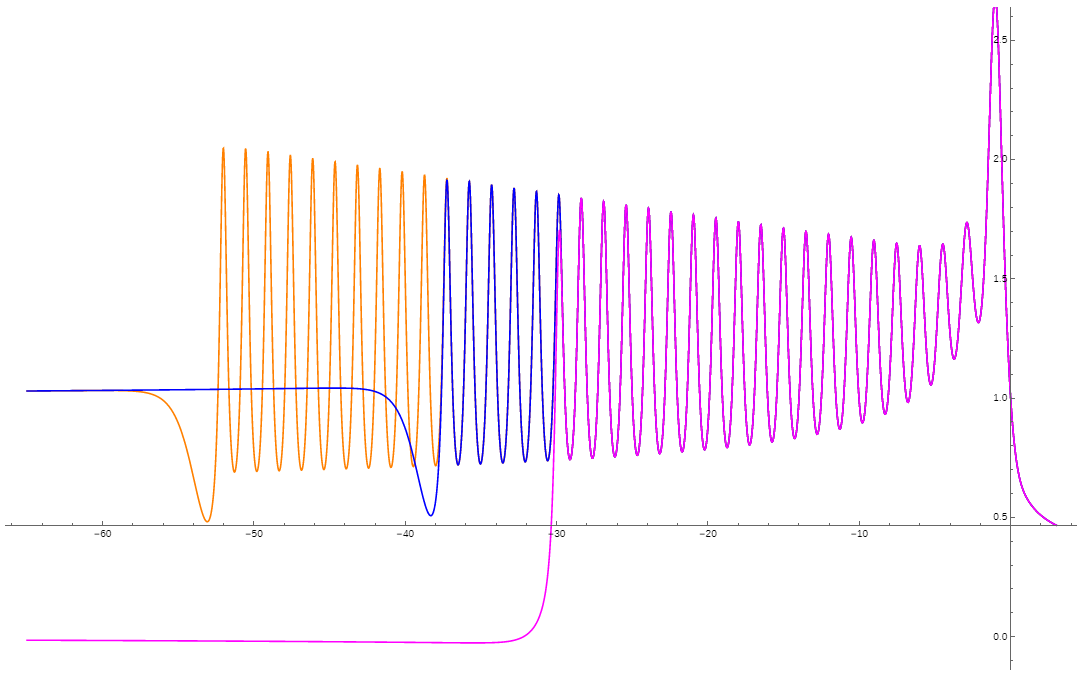
Zooming out (orange with 140 terms; blue with 100 terms; magenta with 79 terms), we observe excellent convergence, until the plots branch off to either 0 or 1 depending on the parity of the number of terms.

How do we explain the regular, wave-like phenomenon over the negative reals?