Suppose that we have $2n$ points $v_i$ in $\mathbf{R}^n$, with equal distance $|v_i|$ from the origin. Suppose that the convex hull of these points contains the unit ball. Is it known that $|v_i|\geq\sqrt{n}$? Presumably, the optimal configuration is given by the vertices of a cross polytope, i.e., when $v_i$ are placed on the coordinate axis with $|v_i|=\sqrt{n}$.
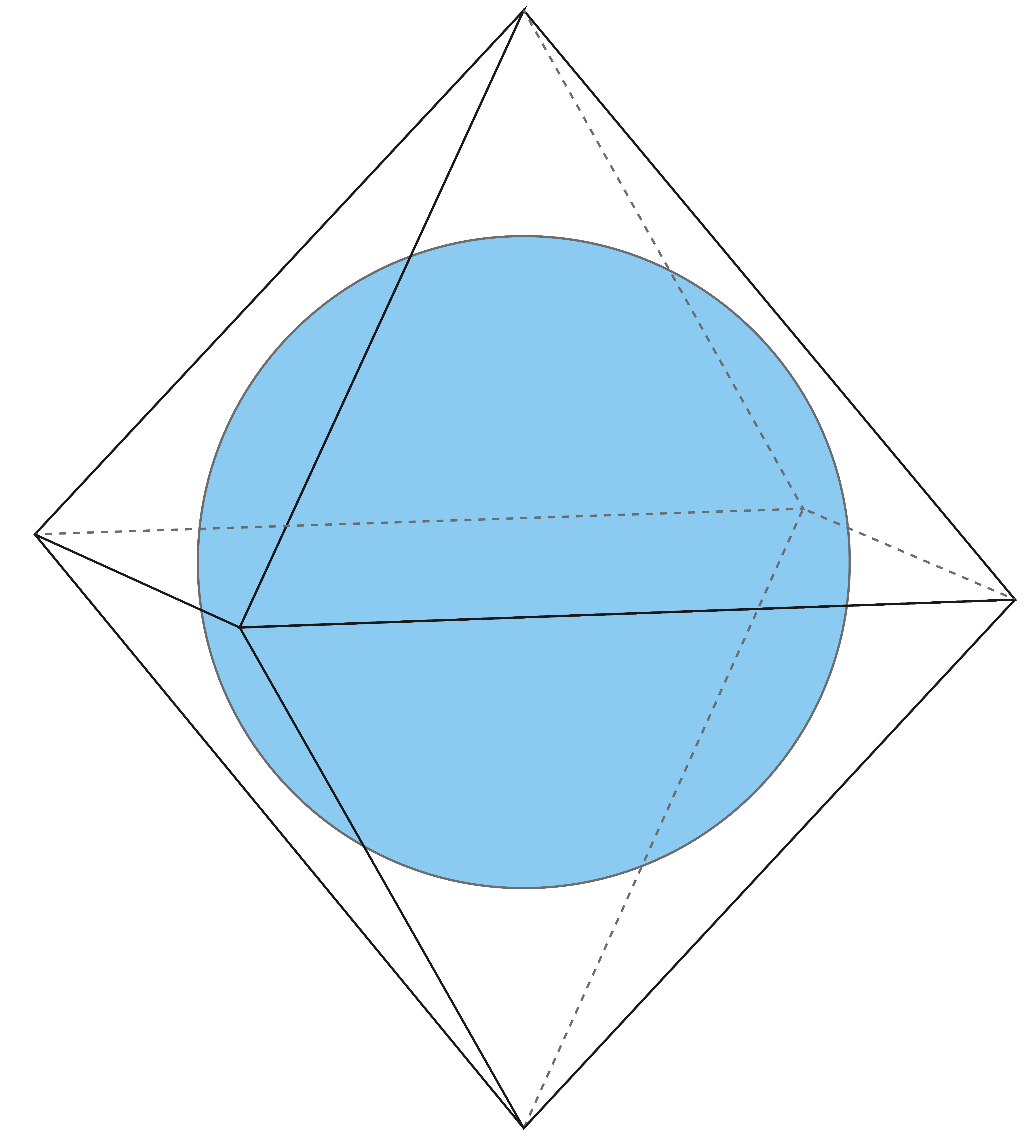
The problem may also be phrased in terms of the smallest radius for $2n$ spherical disks of the same size to cover the unit sphere $\mathbf{S}^{n-1}$. So I imagine that either the answer is known, or else this is a well-known problem in sphere packing. References would be appreciated.
