(Crossposted from MSE due to no replies)

I'm trying to understand the proof that (c) implies (a) here in the following proposition (here, $\mathcal{V} = L^2(0,T;V)$). See the very last line in the image for that part: $$$$

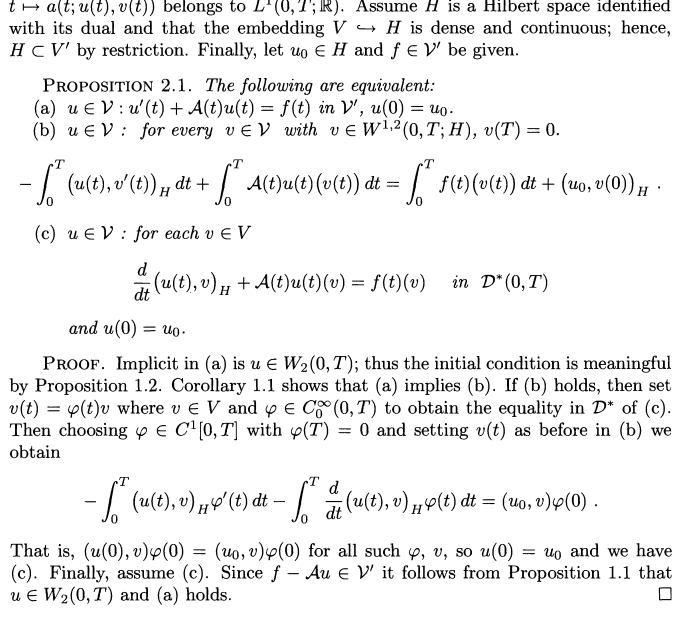$$$$ I give here Proposition 1.1 which the proof uses. $$$$

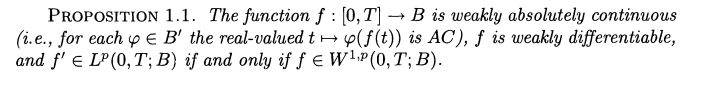
I do not understand how it's used in the proof. I'd appreciate an explanation. Thank you.

Here, $$W_2(0,T) = \{u \in \mathcal V : u' \in \mathcal V'\}$$ and

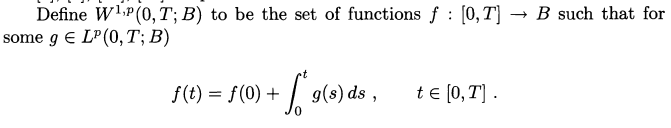
(All images cut from Showalter's book Monotone Operators in Banach Space)