6
$\begingroup$

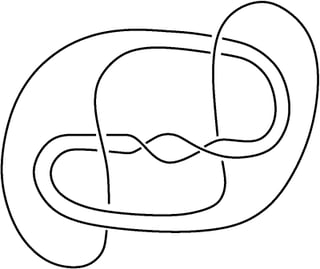
Is there a source in the literature for ribbon diagrams for the knot-table knots known to be ribbon knots?

For example, I'm interested in doing a computation which needs as input a ribbon diagram for the knot $8_{20}$ (Rolfsen knot table notation). This knot is known to be ribbon, but I don't know a ribbon diagram for the knot.

Usually when I encounter a claim of the sort "knot X is ribbon" either the author supplies the ribbon diagram, or nothing. Citations to information of this sort seem kind of sparse. Or am I just unaware of a standard source for this type of information?

Improve this question
$\endgroup$
1

4 Answers 4

Reset to default
7
$\begingroup$

I think Kawauchi's book has tables that include ribbon diagrams, but I don't have a copy with me. Look at Livingston and Cha . It is not hard to get a ribbon disk from this diagram: add a handle between the ears on the top and bottom right.

Generally, I check Livingston/Cha , Bar-Natan, and Saito for various information.

@ears: there are a pair of symmetric clasps on the top and bottom of the diagram. Pull the top-most and bottom-most arc to the right, and then attach a band. The vertical arc that forms a triangle, and the right vertical arc from the band forms an obvious embedded circle.

Improve this answer
$\endgroup$
2
  • $\begingroup$ I have Kawauchi's book in my office. Maybe I missed the ribbon diagrams? "add a handle between the ears..." I'm not so sure what this means. $\endgroup$
    – Ryan Budney
    Commented Dec 13, 2009 at 1:40
  • $\begingroup$ Oh, thanks. Yes, it's in Kawauchi. Google books indexes the ribbon diagrams, too! Merry Christmas from Google books, saves me a trip to the office. $\endgroup$
    – Ryan Budney
    Commented Dec 13, 2009 at 1:53
3
$\begingroup$

The Knot Atlas seems like it would be a good home for ribbon diagrams. At present, it has exactly one: for the knot 61.

The Knot Atlas is a wiki, it's user editable, and you can even upload new images.

Improve this answer
$\endgroup$
3
  • $\begingroup$ I'd be happy to do that but editing that wiki appears very complicated as everything is auto-generated -- using the "edit" link would likely mean my edit would be auto-erased the next time the page is generated? $\endgroup$
    – Ryan Budney
    Commented Dec 13, 2009 at 21:05
  • 2
    $\begingroup$ It is a bit evil. The right thing to do is to find the link [edit Notes for 6_1's three dimensional invariants]. That takes you to a sub-page which is not automatically generated, but which is 'transcluded' into the main page for 6_1. $\endgroup$
    – Kim Morrison
    Commented Dec 13, 2009 at 22:20
  • $\begingroup$ Okidokie, I think I may have done the right thing. ? $\endgroup$
    – Ryan Budney
    Commented Dec 13, 2009 at 22:38
3
$\begingroup$

    alt text (source)

tada: link text

Improve this answer
$\endgroup$
1
  • 2
    $\begingroup$ Very pretty indeed! $\endgroup$
    – Sam Nead
    Commented Dec 13, 2009 at 23:31
3
$\begingroup$

See also 'A refined Jones polynomial for symmetric unions', Michael Eisermann and Christoph Lamm, Osaka J. Math. (2011), https://projecteuclid.org/euclid.ojm/1315318344

All prime ribbon knots up to 10 crossings are given as symmetric diagrams (examples 1.14, 6.6, 6.7 and 6.8, see especially the table on page 363) which are simpler than the diagrams in Kawauchi's book.

Update 2022: My paper "The search for nonsymmetric ribbon knots" contains ribbon diagrams for all 11 and 12 crossing (prime, ribbon) knots.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .