The "Dzhanibekov effect" - an exercise in mechanics or fiction? Explain mathematically a video from a space station

Question

The question briefly:

Can one explain the "Dzhanibekov effect" (see youtube videos from space station or comments below) on the basis of the standard rigid body dynamics using Euler's equations? (Or explain that this is impossible and that would be yet another focus ... )

Here are more details.

See these curious videos from a space station:

https://www.youtube.com/watch?v=L2o9eBl_Gzw

Or in the original form which is more striking (~30 seconds after the start): https://www.youtube.com/watch?v=dL6Pt1O_gSE

A mathematical description of the model is the standard rigid body motion in empty space (since we are in outer space), which (as is well-known) can be decomposed into a center of mass motion and a rotation part described by Euler's equations. (Euler's equations can be seen as a geodesic flow for some left-invariant metric on SO(3) - as V.I. Arnold taught.) Let us forget about the center of mass motion.

The phenomena shown in the videos is the following - a rigid body is rotating around the axis and then SUDDENLY the rotation axis CHANGES ITS POSITION by 180 degrees. And this happens periodically with some time period.

I am a little puzzled how to explain this.

If we admit that the motion of this rigid body is EXACTLY the rotation around the axis this is for sure IMPOSSIBLE - Euler's equations predict that such a motion will continue forever. However in the real world there is nothing exact so it might be related to some instability effect - that we are around an unstable equilibrium - and go away from it after some time...

Can it be so?

If it so, it is however not clear why it happens so quickly and we get exactly the rotation axis changing its position by 180 degrees...

---

Vladimir Dzhanibekov who observed this in 1985 is a famous Russian cosmonaut https://en.wikipedia.org/wiki/Vladimir_Dzhanibekov He was in space 5 times (as far as I know he is the champion in this). In 1985 his "mission impossible" was saving the Soviet space station "Salut-7" which due to some problems had run out of control...

---

If this can be explained I think it can be a beautiful illustration for students in lectures on rigid body mechanics... Also if one would want to add some humor in such a lecture one may add that our Earth is this kind of finger nut so it might also do such things ... (Maybe it really can?)

Answer 1

One can see this effect qualitatively from Newtonian first principles such as $F=ma$ (as opposed to Hamiltonian or Lagrangian principles, such as conservation of energy and angular momentum) by looking at a degenerate case, when one moment of inertia is very small and the other two are very close to each other.

More specifically, consider a thin rigid unit disk, initially oriented in the $xy$ plane and centred at the origin $(0,0,0)$. We make the "spherical cow" hypotheses that this disk has infinitesimal thickness and mass, but infinite rigidity. On this disk, we place heavy point masses of equal mass $M$ at the points $(1,0,0)$ and $(-1,0,0)$ on the $x$ axis, and light point masses of equal mass $m$ at the points $(0,1,0)$ and $(0,-1,0)$ on the $y$ axis. Here $0 < m \ll M$, i.e. $m$ should be viewed as negligible with respect to $M$. (The moments of inertia are then $2m, 2M, 2(m+M)$, though we will not explicitly use these moments in the analysis below.)

We now set up the unstable equilibrium by rotating the disk around the $y$ axis. Thus, the light $m$-masses stay fixed on the $y$-axis, while the heavy $M$-masses rotate in the $xz$-plane. This is in equilibrium: there are no net forces on the $m$-masses, while the rigid disk exerts a centripetal force on the $M$-masses that keeps them moving in a circular motion on the $xz$-plane.

We can view this equilibrium in rotating coordinates, matching the motion of the $M$-masses. (Imagine a camera viewing the disk, rotating around the $y$-axis at exactly the same rate as the disk is rotating.) In this rotating frame, the disk is now stationary (so the $m$-masses are stuck on the $y$-axis at $(0,\pm 1,0)$ and the $M$-masses are stuck on the $x$-axis at $(\pm 1,0,0)$), but there is a centrifugal force exerted on all bodies proportional to the distance to the $y$-axis. The $m$-masses are on the $y$-axis and thus experience no centrifugal force; but the $M$-masses are away from the $y$-axis and thus experience a centrifugal force, which is then balanced out by the centripetal forces of the rigid disk.

Now let us perturb the disk a bit, so that the $m$-masses and $M$-masses are knocked a little bit out of position (but keeping the centre of mass fixed at $(0,0,0)$). In particular, the $m$-masses are knocked away from the $y$-axis and now experience a little bit of centrifugal force. On the other hand, the rigid disk forces the light $m$-masses to remain orthogonal to the heavy $M$-masses, by exerting tension forces between the masses. In the regime where $m$ is negligible compared to $M$, these tension forces will barely budge the heavy $M$ masses (which therefore remain essentially fixed at $(\pm 1,0,0)$ in the rotating frame), so the effect of these tension forces is to constrain the $m$-masses to lie in the $yz$-plane (up to negligible errors which we now ignore). Rigidity also keeps the $m$-masses at a unit distance from the origin, and antipodal to each other, so the $m$-masses are now constrained to be antipodal points on the unit circle in the $yz$-plane. However, other than this, rigidity imposes no further constraints on the location of the $m$-masses, which can then move freely as antipodal points in this unit circle.

The effect of centrifugal force in the rotating frame is now clear: if an $m$-mass (and its antipode) is perturbed to be a little bit off the $y$-axis in this unit circle with no initial velocity, then centrifugal force will nudge it a little further off the $y$-axis, slowly at first but with inexorable acceleration. Eventually it will shoot across the unit circle and then approach the antipode of its previous position. At this point the centrifugal forces act to slow the $m$-masses down, reversing all the previous acceleration, until one ends up with no velocity at a small distance from the antipode. The process then repeats itself (imagine a marble rolling frictionlessly between two equally tall hills, starting from a position very close to the peak of one of the hills).

UPDATE, September 2019: Due to renewed interest in this question, I will expand upon my 2014 comment regarding why the above analysis appears at first glance to also lead to the incorrect conclusion that the disk rotation is also unstable if it one instead rotates around the $x$-axis (so that it is now the $M$-masses that are stationary and the $m$-masses that are rotating), or equivalently if one swaps the location of the $m$-masses and $M$-masses (which we will not do here to try to reduce confusion).

The reason for this is that centrifugal force $F_{\mathrm{Cent}} = -m \Omega \times \Omega \times r$ is only one of two inertial forces that are introduced when one is in a steadily rotating frame. The other inertial force introduced is the Coriolis force $F_{\mathrm{Cor}} = -2 m \Omega \times v$, which acts on moving bodies in the rotating reference frame in a direction orthogonal to the motion (and to the axis of rotation). Strictly speaking, one has to take into account the effect of both inertial forces when performing Newtonian mechanics in a steadily rotating frame. As it turns out, the Coriolis force has a negligible impact on the dynamics when rotating around the $y$-axis, but dominates the dynamics when rotating around the $x$-axis, which is why the preceding discussion is accurate in the former case but not the latter.

In more detail: suppose we are rotating around the $y$-axis as in the above discussion. In the rotating frame of reference, and starting with a configuration slightly out of equilibrium, we have as before that the $m$-masses experience a little bit of centrifugal force and begin to slide away from the $y$-axis and into the rest of the $yz$-plane. When doing so, they will then experience some Coriolis force in a direction parallel to the $x$-axis (which direction it is depends on the orientation of the rotation, as per the right hand rule formula for the cross product). However, due to the rigidity of the disk, as mediated by tension forces within the disk, it is not possible for the $m$-masses to actually move in the $x$-direction without also moving the much heavier $M$-masses. But the magnitude of the Coriolis force is proportional to the small mass $m$ rather than the large mass $M$, so by Newton's law $F=Ma$ for the $M$-masses, the Coriolis force (or more precisely, the tension force produced in response to the Coriolis force) actually barely affects the motion of the $M$-masses, which basically stay put on the $x$-axis, and the $m$-masses therefore remain essentially constrained to the $yz$-plane and cannot actually experience any significant motion in the direction of the Coriolis force. (This is what the sentence in the original explanation regarding how tension forces "barely budge" the $M$-masses was referring too, albeit somewhat obliquely.) The analysis of the original explanation now proceeds as before.

In contrast, suppose that the disk is close to the stable equilibrium state when it rotates around the $x$-axis. Working in a rotating frame around this axis, the $M$-masses are near the $x$-axis of rotation, while the $m$-masses lie near the $y$-axis. As before, the $M$-masses experience centrifugal force and thus begin drifting slightly away from the $x$-axis into the $xz$-plane. But then the Coriolis force on these masses kicks in, which is now proportional to the heavy mass $M$ rather than the light mass $m$. As such, the rigid tension forces connecting the $M$-masses to the much lighter $m$-masses offer very little resistance to the Coriolis force, and the motion of the $M$-mass begins to rotate out of the $xz$-plane, constantly experiencing Coriolis acceleration in a direction orthogonal to its motion. This effect tends to make the $M$-mass rotate in a tight circle, and basically neutralises the net effect of the centrifugal force by rotating any outward motion away from the $x$-axis back into inward motion. The end result is that in the rotating frame, the disk wobbles a bit around its equilibrium state, but does not dramatically depart from it, which is what one would expect from a stable equilbrium (imagine now a marble rolling frictionlessly around the trough of a valley).

Answer 2

In English-speaking physics classes, this is usually called the "Tennis Racket Theorem", because a tennis racket is a good example of a physical object with three widely separated moments of inertia. If you look in this video, you can see the 180 degree flip you describe taking place here on Earth. The interesting difference between Earth and space is that, on Earth, it is difficult to watch an object in free-fall for more than a second, so you only see a fraction of the orbits described in Marcos and Victor's answers; the video I link to looks like about half an orbit. In space, you can see the cycle happen several times.

There are tons of proofs of the tennis racket theorem online, here is one (Wayback Machine).

This question was also asked on physics.SE; the answer there linked to this nice paper.

Answer 3

Take a look at "Rigid Body Stability Theorem" in chapter 15 of Marsden and Ratiu's "Introduction to Mechanics and Symmetry", which basically states that in situations like the one in the video (where, judging by the shape of the rotating object, the principal moments of inertia are mutually distinct), rotation about the middle principal axis is unstable. While the angular momentum of the rigid body is constant in its spatial representation, it is nonconstant with respect to the body's frame. There is a figure following the aforementioned theorem which shows the flow lines for the angular momentum with respect to the body's frame. There are three pairs of antipodal fixed points corresponding to rotation exactly about the principal axes. The "long" and "short" axes' critical points are stable (in the sense of Liapunov), while the "middle" critical points are saddle points, and therefore unstable. Furthermore, there are flow lines coming arbitrarily close to both of the antipodal "middle" critical points. I believe the solutions associated with these flow lines are what you seek.

Answer 4

I started adding comments to Victor's answer, but they finally outgrew to this:

From an elementary point of view, the energy is conserved, and also the angular momentum vector $L$ (measured with respect to an inertial frame).

From a Lagrangian-mechanics-on-manifolds point of view, we have to solve a problem of movement in $SO_3$, that can be parametrized using Euler angles. Since there is only kinetic energy (no potential), it is equivalent to a problem of geodesic flow in a Riemannian manifold, and affine reparametrizations of solutions will again be solutions. This simply means that every solution can be sped up at wish.

Only the first angle in the composition will be a cyclic coordinate, but this is an artifact of the parametrization, since we see that there is $SO_3$ symmetry, i.e., the whole "position component" should be cyclic, and we would expect to reduce the second order o.d.e. on "position" to a (first order) problem on "speed".

(Is this intuition correct? Can it be done in other problems?)

To do this, we go to the frame of reference of the body. We try to find the motion of the angular speed vector $\omega$ with respect to it . This is a first order three dimensional problem, governed by the Euler equations $I_1\dot\omega_1=(I_2-I_3)\omega_2\omega_3$ (1)

$I_2\dot\omega_2=(I_3-I_1)\omega_3\omega_1$ (2)

$I_3\dot\omega_3=(I_1-I_2)\omega_1\omega_2$ (3),

which are expressed in principal axes coordinates.

(I understand this way of changing reference frames as an abuse of notation: the two reference frames $body$ and $space$ are different (Euclidean) spaces (even before coordinatizing them), and they are related by an isometry $body\to space$ (the attitude of the body) that changes with time (according to a function that we are trying to find). So every vector is two vectors: we actually have two angular speed vectors $\omega_{space}$ and $\omega_{body}$. This is relevant when we derivate them with respect to time. For example, $L_{space}$ is constant but $L_{body}$ isn't. This idea is simply a coordinate-free formalization of the notion of measuring speed "with respect to a reference frame".)

We can also express the problem in terms of the angular moment vector $L$, which is linearly equivalent to $\omega$ via the equation $L=I\omega$. I will switch between both versions when convenient.

Using the conservation of energy $2E=\langle\omega,I\omega\rangle$, we see the movement is constrained to an ellipsoid. So we have a two dimensional first order problem. You can search "Poinsot ellipsoid" in Google Images to see it with the flow lines drawn on it. The only equilibrium points are (from looking at the Euler equations) at the principal axes of the ellipsoid, which are the lines along which uniform rotation is possible.

Assuming $I_1>I_2>I_3$ we find only six equilibrium points, for example: $(\sqrt{2E/I_1},0,0)$. Near it we can express $\omega_1=\sqrt{(2E-I_2\omega_2^2-I_3\omega_3^2)/I_1}$ and write the second and third Euler equations for $\omega_2$ and $\omega_3$. Linearizing at $(0,0)$ we get

$I_2\dot\omega_2=(I_3-I_1)\sqrt{2E/I_1}\omega_3$ (2')

$I_3\dot\omega_3=(I_1-I_2)\sqrt{2E/I_1}\omega_2$ (3')

and since $I_3-I_1<0$ and $I_1-I_2>0$, we get imaginary eigenvalues, which means the equilibrium point is possibly neutrally stable. We confirm that the trajectories around it are closed by observing the reflection symmetry $(\omega_2,\omega_3)\to(-\omega_2,\omega_3)$. This happens at the equilibriums associated to the smallest moment of inertia $I_3$. At $(0,\sqrt{2E/I_2},0,0)$ we have two real opposite eigenvalues, which means the equilibrium point is unstable. We can determine the topology of the flow lines completely by analyzing the signs in the Euler equation in each octant, but it is easier to use another constant of movement.

The length of $L$ is constant, so the trajectory will be the intersection of the Poinsot ellipsoid with a sphere (or with an ellipsoid having the same principal axes, to phrase everything in terms of $\omega$ instead of $L$, but what follows is most clearly seen in the $L$ picture).

If the sphere is smallest, we get a pair of opposite points on the first axis.

If the sphere is small, we get a pair of closed curves around the first axis.

If the sphere is large, we get a pair of closed curves around the third axis.

If the sphere is largest, we get a pair of points on the third axis.

The middle case, corresponding to a medium sphere, contains the equilibrium points of the second axis (lets call them $A$ and $B$), joined by four curves (lets call them special). Each special curve is topologically equivalent to an open interval, and travel along it requires infinite time (since from unicity of solutions, we can't expect to reach an equilibrium point in finite time).

If we attempt to make a body rotate along an unstable axis in practice, we will miss, and obtain a closed curve around the first or third axis that will pass periodically near $A$ and $B$. Since motion near an equilibrium point is slow, the curve will spend most time near them, and will be similar to a pair of special curves. If we speed this up by giving a high initial energy, we get this periodic, almost instantaneous switch from $A$ to $B$.

With respect to an inertial frame, since $L_{space}$ is constant, we see that the body always rotates in the same direction, switching its position to the opposite after a period $T$. The movement is quasi-periodic: after time $T$, the movement restarts but with a possible angular shift around the $L_{space}$ axis (whose magnitude is always the same for a certain trajectory and can't be calculated easily (as far as I know)).

Answer 5

Perhaps I'm too late here, but there's a simple treatment which does not refer to Euler's equations.

Let $A$ denote the inertia tensor of the body, that is, if $\omega_b$ is its angular velocity in the frame of the body, then $M_b:=A\omega_b$ is its angular momentum and $E:=(A\omega_b;\omega_b)$ is its kinetic energy. The position of the body at each moment $t$ is described by an orthogonal matrix $C_t$; if $\omega$ and $M$ denote its angular velocity and momentum in the laboratory frame, then $$ M=C^{-1}_tAC_t\omega, $$ and the kinetic energy is given by $$ E=(M;w)=(C_tM;A^{-1}C_t M). $$ However we know that both $M$ and $E$ are conserved. Hence, if we draw level lines of the quadratic form $v\mapsto (v;A^{-1}v)$ on the sphere $|v|=|M|$, then $C_t$ maps the fixed vector $M$ to some other vector on the same level line. Near maximum an minimum, these level lines are small closed loops, but the intermediate eigenvalue of $A$ corresponds to a saddle point; the nearby level line goes all the way around the sphere to the opposite saddle point. This proves that the former rotations are stable and explains why the latter is not. If you just know that there are some first-order dynamics for $\omega$ in the body frame and that the eigenvalues of $A$ are its only fixed points, then you get a complete qualitative picture.

Answer 6

This isn't in any way a complete answer but I do find it illuminating to see this behaviour in a numerical simulation. In particular we see the periodic flips that seem to appear out of nowhere. Note how angular momentum is temporarily stored in the $\omega_1$ and $\omega_3$ components of the angular velocity during the flips.

Answer 7

It seems that the universally accepted interpretation of the Dzhanibekov effect is that it's nothing but a case of the intermediate axis theorem, which has been known in classical mechanics for at least 150 years.

The tennis racket effect is verified easily with simple experiments, so there shall be no doubt in its validity. However, Dzhanibekov effect cannot be reduced to the intermediate axis theorem – a point that seems has not been pointed out yet.

To be specific, it will be demonstrated below that there are cases of the Dzhanibekov effect which clearly contradict the intermediate axis theorem. (The theorem states: rotation of a rigid body with three distinct principal moments of inertia, $I_1 > I_2 > I_3$, about the intermediate axis is unstable, while rotation about two others is stable).

Now consider the following two axisymmetric cases:

(1) $I_1 = I_2 > I_3$ with a spin about the axis of least moment of inertia;

(2) $I_1 > I_2 = I_3$ with a spin about the axis of maximal moment of inertia.

The intermediate axis theorem has nothing to say about these borderline cases. But it can be proved directly that spin in both cases is still stable, so there shall be no flipping of the body. Call it an extension of the intermediate axis theorem, if you wish.

Proof: In torque-free conditions, the equations of rotation of asymmetric top around its center of inertia in the frame of reference which is rigidly fixed to the body, axes $x_1, x_2, x_3$ being directed along the body’s three principal axes of inertia, are:

$$ I_1\dot{\omega}_1 = (I_2 - I_3) \omega_2\omega_3, $$ $$ I_2\dot{\omega}_2 = (I_3 - I_1) \omega_3\omega_1, $$ $$ I_3\dot{\omega}_3 = (I_1 - I_2) \omega_1\omega_2. $$

Here $I_1, I_2, I_3$ denote the body's principal moments of inertia while the angular velocities around the corresponding axes are denoted by $\omega_1, \omega _2, \omega _3$.

In the case of axisymmetric top, say $I_1 = I_2$ (both being equal to some $I$), we have Euler’s equations reduced to:

$$ I\dot{\omega}_1 = (I - I_3)\omega_2\omega_3, $$ $$ I\dot{\omega}_2 = (I_3 - I)\omega_3\omega_1, $$ $$ I_3\dot{\omega}_3 = 0. $$

From the third equation we have $\omega_3 =$ const, so the other two are greatly simplified:

$$ \dot{\omega}_1 = +\Omega \omega_2, $$ $$ \dot{\omega}_2 = -\Omega \omega_1, $$

where $\Omega \equiv (I - I_3)\omega_3/I$.

Therefore, we have an analytical solution with a fixed angular velocity of precession $\Omega$, which prevents the axis of rotation from flipping over:

$$ \omega_1(t) = \omega \sin(\Omega t), $$ $$ \omega_2(t) = \omega \cos(\Omega t), $$ $$ \omega_3(t) = \omega_3 = \text{const}. $$

The constants of integration $\omega$ and $\omega_3$ are determined uniquely by the angular momentum $M$ and the angle of precession $\theta$: $$ \omega = M\sin(\theta)/I, $$ $$ \omega_3 = M\cos(\theta)/I_3. $$ End of proof.

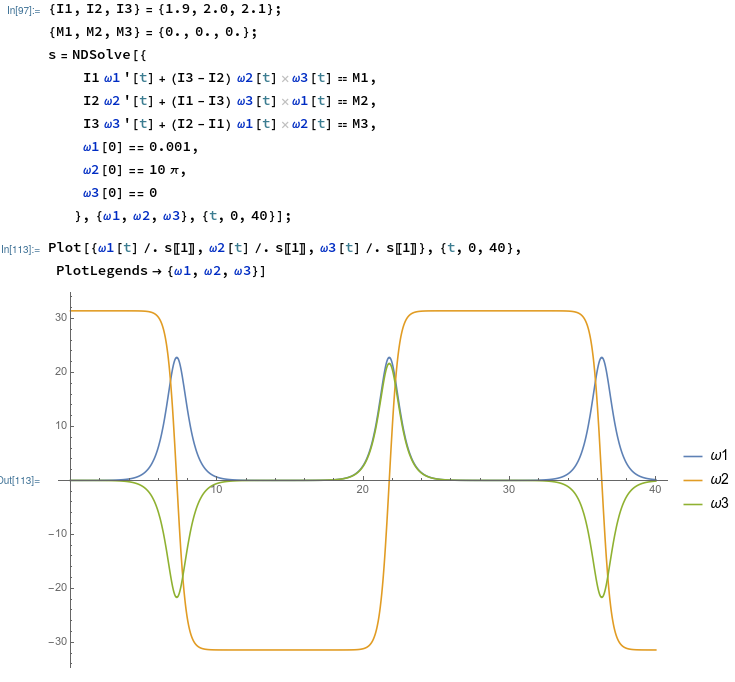
Now we proceed to the key point: Dzhanibekov has observed flipping not only for a wing nut (asymmetric object with $I_1 > I_2 > I_3$ spinning about the intermediate axis), which is consistent with the tennis racket theorem, but also for a nearly axisymmetric body (a regular hexagon nut attached to a ball of modelling clay $-$ a body with $I_1 \gtrapprox I_2 > I_3$ spinning about the least-inertia axis) in clear violation of the intermediate axis theorem:

The Bizarre Behavior of Rotating Bodies by Veritasium.

(Note that the density of iron is higher than the density of plasticine, so there can be no doubt that we have here an object with $I_1 \gtrapprox I_2 > I_3$ spinning about the least-inertia axis. For brevity, let us call this case the Dzhanibekov top.)

Conclusion: Equating Dzhanibekov effect with the tennis racket instability is a blunder. So, the real physical cause for the instability of the Dzhanibekov top needs to be identified.

It is certain that dissipation of kinetic energy, which is due to internal forces, cannot be the physical cause of this effect. Indeed, even if we allow for this mechanism, all it can do is to increase the angle of precession to its maximum possible value of $\theta = \pi/2$, at which point the dissipation of kinetic energy must stop. So, the flipping back and forth of the Dzhanibekov top cannot be accounted for by the energy dissipation mechanism.

Therefore remains only one credible explanation for the behavior of the Dzhanibekov top: it is due to an external torque, aerodynamic interaction with the surrounding air being the most likely candidate for the source of the external torque. This can be tested easily. If the Dzhanibekov top stops flipping in a vacuum (say, outside the ISS), we can be certain that aerodynamic interaction with the air inside the ISS was the real culprit for the effect.

From the viewpoint of the physical cause of flipping, the Dzhanibekov top is a cousin of the Thomson top (tippe top), rather than the tennis racket. Indeed, flipping of the tennis racket will persist in vacuum conditions; on the other hand, flipping of the Dzhanibekov top requires the assistance of external torques, that is – just like the tippe top – it cannot take place in a torque-free environment.

P.S. Take a look at this picture of Wolfgang Pauli and Niels Bohr – two old guys spellbound and observing with childish elation the weird pattern of motion of a fast spinning tippe top:

http://www.fysikbasen.dk/Images/Figurer/pauli_bohr_tippetop.jpg

Isn't it lovely?

The picture is taken at the opening of the new institute of physics at the University of Lund on May 31 1951. Credit: Photograph by Erik Gustafson, courtesy AIP Emilio Segre Visual Archives, Margrethe Bohr Collection.

Answer 8

I don't think there is an "intuitive" explanation to the effect. As an ex-physicist I don't find rotational movement intuitive, maybe evolutionary we don't encounter them that often to develop senses around them. So, the answer really is in equations and breaking the symmetry of sorts: the magnitudes of inertia moments are ordered, so the rotation around one in the middle is different than around the other ones.

One thing to note is that people mostly pay attention to the case where the main rotation is around the second principal moment of inertia, where the seemingly sudden and violent change in direction is startling to an observer. However, in order to grasp the effect one must understand that regardless of initial state rotation direction the rotation along the second moment is always periodic, i.e. it always changes the direction! It's just sometimes it is not noticeable.

Here's the details. The main set of equation where we start is in Wikipedia: $$I_1\dot\omega_1=(I_{3}-I_{2})\omega_{3}\omega_{2}$$ $$I_2\dot\omega_2=(I_{1}-I_{3})\omega_{1}\omega_{3}$$ $$I_3\dot\omega_3=(I_{2}-I_{1})\omega_{2}\omega_{1}$$ Where $I_i$ are moments of inertia and $\omega_i$ are rotation velocities along these moments. so the overall rotation of the body can be represented by rotations around these three principal moments.

Now, let's take one more derivative with respect to time: $$I_1\ddot\omega_1=(I_{2}-I_{3})\left(\frac{I_1-I_2}{I_3}\omega_2^2-\frac{I_1-I_3}{I_2}\omega_3^2\right)\omega_1$$ $$I_2\ddot\omega_2=-(I_{1}-I_{3})\left(\frac{I_2-I_3}{I_1}\omega_1^2+\frac{I_1-I_2}{I_3}\omega_3^2\right)\omega_2$$ $$I_1\ddot\omega_3=(I_{1}-I_{2})\left(-\frac{I_1-I_3}{I_2}\omega_2^2+\frac{I_2-I_3}{I_1}\omega_1^2\right)\omega_3$$

I re-arranged the terms in such a way that all fractions and subtractions in parentheses are positive, so that it becomes very evident that only the rotation velocity along the second moment $\omega_2$ is always periodic: $\ddot\omega\sim-\omega$. This velocity always oscillates. This is the most important aspect of the rotational movement.

It is only when you start the rotation with a significant velocity $\omega_2(0)\gg 0$ the effect is so spectacular, where it violently rotates the body and changes the direction, but it is always there!

You can see that the dynamics of other angular velocities $\omega_1,\omega_2$ can vary depending on what's going on with other velocities which is determined by the initial speeds. Sometimes you have $\ddot\omega_i\sim\omega_i$ which corresponds to either exponential decay $\omega_i(t)\sim e^{-t}$ or explosion $\omega_i(t)\sim e^{t}$. At other times it becomes periodic too $\ddot\omega_i\sim-\omega_i$.

So, when you start with $\omega_2\gg 0$ it is more likely that other two rotations $\omega_1,\omega_3$ are likely to go through this changes of dynamics that I described above because $\omega_2$ will be smaller or larger in relation to other rotations, changing the signs of $\left(-\frac{I_1-I_3}{I_2}\omega_2^2+\frac{I_2-I_3}{I_1}\omega_1^2\right)$, for example, which will be changing the dynamics of the $\omega_3$ in this case. All while $\omega_2$ is always doing its periodic dynamics, albeit with always changing periodicity.

When $\omega_2(0)\approx 0$ then it's likely that the sign and the amplitude in $\left(-\frac{I_1-I_3}{I_2}\omega_2^2+\frac{I_2-I_3}{I_1}\omega_1^2\right)$ term and its equivalent for $\omega_1$ stable, which makes overall rotation of the body rather stable too.