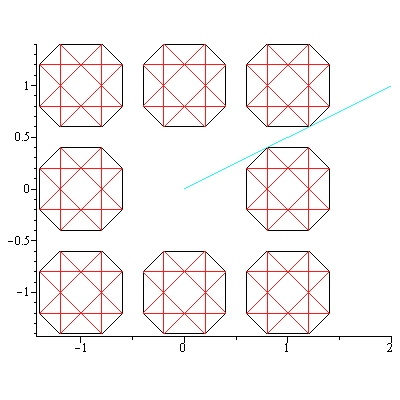
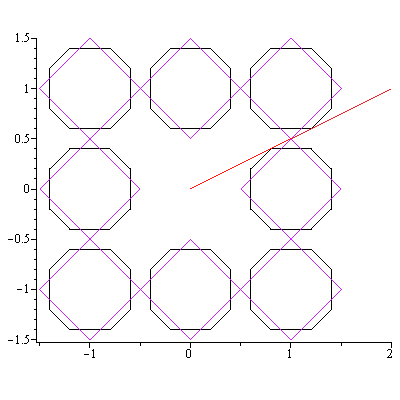
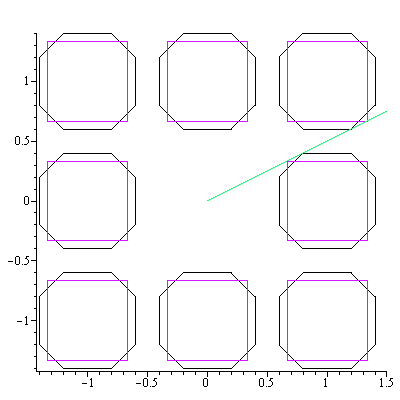
Pólya's orchard problem asks for which radius $\rho$ of trees at each lattice point within a distance $R$ of the origin block all lines of sight to the exterior of the orchard.
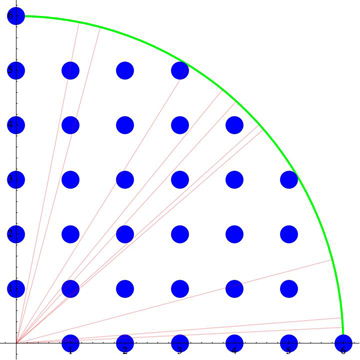
It has been established that rays to infinity are completely blocked iff
$\rho \ge 1/\sqrt{R^2 + 1}$, when $R$ is an integer.
(T.T. Allen, "Polya's orchard problem,"
The American Mathematical Monthly
93(2): 98-104 (1986).)
The above shows a quarter of an orchard with $R=6$, $\rho=1/\sqrt{37}=0.164$,
and some random rays.
I am wondering if disks are the most efficient blockers in terms of area. More precisely:
For a given $R$, is there a centrally symmetric convex body $K$ of area less than $\pi \rho^2$ which when translated to all lattice points within distance $R$ of the origin, block all rays from the origin to the outside?
My guess is that the answer is Yes, in which case it would be interesting to know the shape of the area-optimal blockers. In particular, are they polygons? The same question may be posed in $\mathbb{R}^d$: are they polytopes?
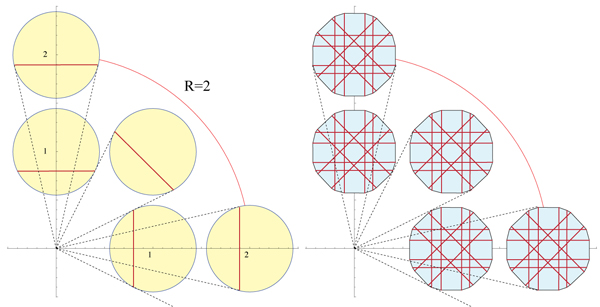
Edit. Here is the chord construction for $R=2$ from the first paragraph of Douglas's construction, as I understand it: