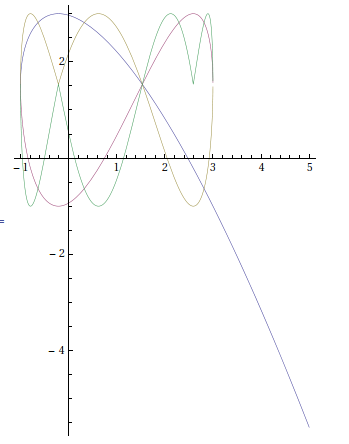
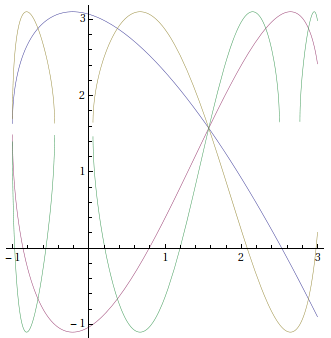
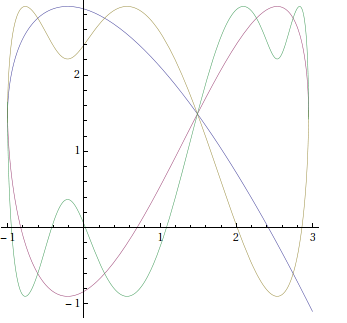
The following function
$$f(x)=-2 \cos \left(\sqrt{2} \arccos \left(\frac{x-1}{2}\right)\right)+1$$
has interesting property to form a continuous curve with its own integer iterations. The following image illustrates this property:
Here blue is $f(x)$, red is $f(f(x))$, yellow is $f(f(f(x)))$ and green is $f(f(f(f(x))))$. It seems that all these functions form a continuous, and, probably, smooth curve.
The question is what is the general criterion for a function to have such property. Can you point some more examples of functions with such property?
P.S. If to use the following function $$f(x)=-2 \cos \left(\sqrt{2} \arccos \left(\frac{x-1}{2}\right)\right)+1.1$$ the curve becomes as below with one continuous branch and numerous closed circular branches.
For function $$f(x)=-2 \cos \left(\sqrt{2} \arccos \left(\frac{x-1}{2}\right)\right)+0.9$$ the curve is completely continuous and seems to be smooth.