Exotic series for some mathematical constants from String Theory

Question

Following these MO posts from Timothy Chow and Henri Cohen and their comments, based on Saha & Sinha's String Theory article, I have found three series for $\pi$, Apéry's constant $\zeta(3)$ and the Lemniscate constant $2\varpi$. Their summands have non-linear (rational or algebraic) Gamma Function or Pochhammer's arguments which makes them very atypical.

All series are slowly convergent but can be accelerated applying $\Delta$-Euler-MacLaurin Method by Henri Cohen which is implemented in PARI-GP script sumnumdelta() as it is seen below.

Will it be possible to prove them?

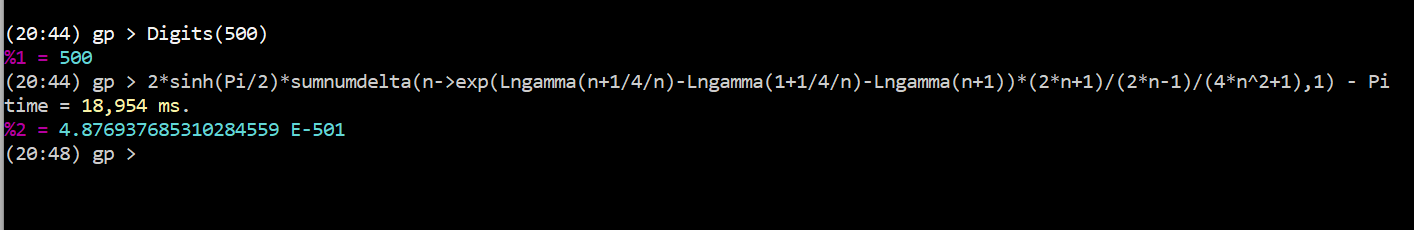
I.- $\pi$ series $$\pi=\small{2\sinh\small{\left(\frac{\pi}{2}\right)}\cdot\sum_{n=1}^\infty\frac{1}{n!}\cdot\small{\left(1+\frac{1}{4n}\right)_{n-1}}\frac{2n+1}{(2n-1)(4n^2+1)}}$$

II.- Apéry's constant series with a free parameter. For $\phi_n(t)=-\frac{n\,t}{n+t}$ with $t\in\mathbb{C}$ and $n\in\mathbb{N}$ $$\small{\zeta(3)}=\small{\sum_{n=1}^\infty\frac{\small{\left(\phi_n(t)+1\right)_n}}{n^3\,n!}\cdot\small{\left(\frac{n+t}{n}+t\cdot\frac{(n+2t)\,\left[\psi\left(\phi_n(t)+n\right)-\psi\left(\phi_n(t)+1\right)\right]}{n+t}\right)}}$$ where $\small{\psi(x)}$ is the Digamma function and the numerator difference represents a harmonic function with rational arguments. Note that $t=0$ gives the classical $\zeta(3)$ series.

III.- The Lemniscate constant, a trascendental number, see Section 6.1 in

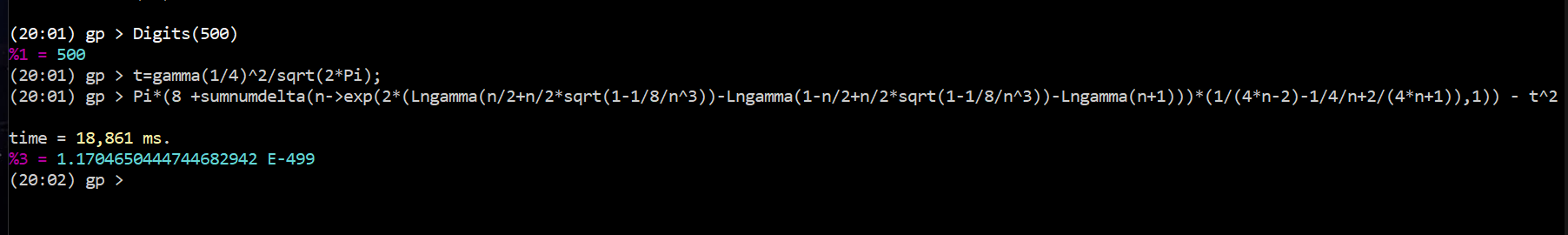
Finch, Steven R., Mathematical constants, Encyclopedia of Mathematics and Its Applications 94. Cambridge: Cambridge University Press (ISBN 0-521-81805-2/hbk). xx, 602 p. (2003). ZBL1054.00001.$$\small{2\varpi} =\small{\frac{\;\Gamma(\frac{1}{4})^2}{\sqrt{2\pi}}=5.2441151085842396209296791797822388273655...}$$ has the following strange series with algebraic Pochhammer's arguments for its squared value $$\small{(2\varpi)^2}=\small{\pi\cdot\left(8+\sum_{n=1}^\infty\small{\left(\frac{1}{4n-2}-\frac{1}{4n}+\frac{2}{4n+1}\right)}\cdot\frac{\left(1-\frac{n}{2}+\frac{n}{2}\sqrt{1-\frac{1}{8n^3}}\right)_{n-1}^2}{n!^2}\right)}$$

Q: It is unknown if there is any proof of these identities. Can a variation of Rosengren's proof be applied for these cases?

Answer 1

My paper on these identities is now available, see https://arxiv.org/abs/2409.06658 .

Your identity III is the case $x_1=x_2=1/4$, $x_3=-1/2$, $\lambda=0$ and $u=1$ of Cor. 5.1. I didn't look at your identity II. It is not quite obvious how to get I so I thought it could be worthwhile to write it down here.

The case $a=1$ of Cor. 3.1 is $$\frac{\Gamma(x_1)\Gamma(x_2)}{\Gamma(x_1+x_2+1)} =\sum_{k=0}^\infty \frac{\left(1-\lambda+\frac{(\lambda-x_1)(\lambda-x_2)}{\lambda+k}\right)_{k-1}}{k!}\left(-\frac 1{x_1+k}-\frac 1{x_2+k}+\frac 1{\lambda+k}\right).$$ We want to take $\lambda=0$. Then the term with $k=0$ needs special treatment, so we move it to the left-hand side and then take the limit $\lambda\rightarrow 0$. This gives $$\frac{\Gamma(x_1)\Gamma(x_2)}{\Gamma(x_1+x_2+1)}-\frac 1{x_1x_2} =\sum_{k=1}^\infty \frac{\left(1+\frac{x_1x_2}{k}\right)_{k-1}}{k!}\left(-\frac 1{x_1+k}-\frac 1{x_2+k}+\frac 1{k}\right).$$ Consider the case $x_1=x_2=-1/2$. Then, $\Gamma(x_1+x_2+1)$ has a pole, so we get $$4 =\sum_{k=1}^\infty \frac{\left(1+\frac{1}{4k}\right)_{k-1}}{k!}\left(\frac 2{k-1/2}-\frac 1{k}\right).$$ Then we take $x_1=i/2$, $x_2=-i/2$ (where $i^2=-1)$. This gives $$\frac{2\pi}{\sinh(\pi)}-4 =\sum_{k=1}^\infty \frac{\left(1+\frac{1}{4k}\right)_{k-1}}{k!}\left(-\frac {8k}{4k^2+1}+\frac 1{k}\right).$$ Adding these two expansions gives $$\frac{2\pi}{\sinh(\pi)} =\sum_{k=1}^\infty \frac{\left(1+\frac{1}{4k}\right)_{k-1}}{k!}\left(-\frac {8k}{4k^2+1}+\frac 2{k-1/2}\right),$$ which is the same as your identity I.

Answer 2

Eq. III., the Lemniscate constant series, is proven using Virasoro Shapiro closed string amplitude Eq.(1.6) in arXiv:2409.06658v1 [math.CA] 10 Sep 2024 "String theory amplitudes and partial fractions" by HJALMAR ROSENGREN, taking parameters $\lambda=s=0$ and $x_1=x_2=\frac14$, leaving out the series first term ($n=0$) and using Pochhammer $(a)_{-1}=\frac1{a-1}$.

Note that by Eq.(1.5) series terms are rational values even if Pochhammers have algebraic arguments.

Eq. II., Apery constant, this is the sketch of the proof. Assume that the Beta function has a generic expansion in terms of the sequence of functions $G_n(x,y)$ of the kind $$B(x,y)=\frac{\Gamma(x)\Gamma(y)}{\Gamma(x+y)}=\sum_{n=0}^\infty\,G_n(x,y)$$ differentiating and naming $\psi(x)$ the digamma function, $$B(x,y)\cdot\left[\psi(y)-\psi(x+y)\right]=\sum_{n=0}^\infty\frac{\partial}{\partial y}G_n(x,y)$$ By setting $y=1$ and $\gamma$ the Euler constant, we get $$\psi(x+1)+\gamma=-x\cdot\sum_{n=0}^\infty\frac{\partial}{\partial y}G_n(x,y)\Big|_{y=1}$$ Expanding the summands in Taylor series $$\frac{\partial}{\partial y}G_n(x,y)\Big|_{y=1}=\sum_{m=0}^\infty\,\frac{x^m}{m!}\cdot g_n^{(m)}$$ We get $$\psi(x+1)+\gamma=-x\cdot\sum_{m=0}^\infty\frac{x^m}{m!}\,\sum_{n=0}^\infty g_n^{(m)}$$ but $\psi(x+1)+\gamma$ is a GF for $\zeta(n)$ as $$\psi(x+1)+\gamma=-x\cdot\sum_{m=0}^\infty(-1)^{m+1}x^m\,\zeta(m+2)$$ So for $m\in \mathbb{Z}_{\ge0}$ $$\zeta(m+2)=\frac{(-1)^{m+1}}{m!}\cdot\sum_{n=0}^\infty\,g_n^{(m)}$$ The proven identity Eq.(1.3) in Rosengren's paper gives the summand sequence $G_n(x,y)$ by taking $x=x_1$, $y=x_2$ and the inert parameter $t=\lambda$. Apery's constant series II is obtained computing for $m=1$ the Taylor coefficients $g_n^{(1)}$. This is cumbersome but it is accomplished with the help of some CAS code. $$\zeta(3)=\sum_{n=0}^\infty\,g_n^{(1)}$$ where $g_n^{(1)}$ are the summands in Eq. II after shifting the sum index.

Eq. I. is proven in the @Hjalmar_Rosengren's answer below.