5
$\begingroup$

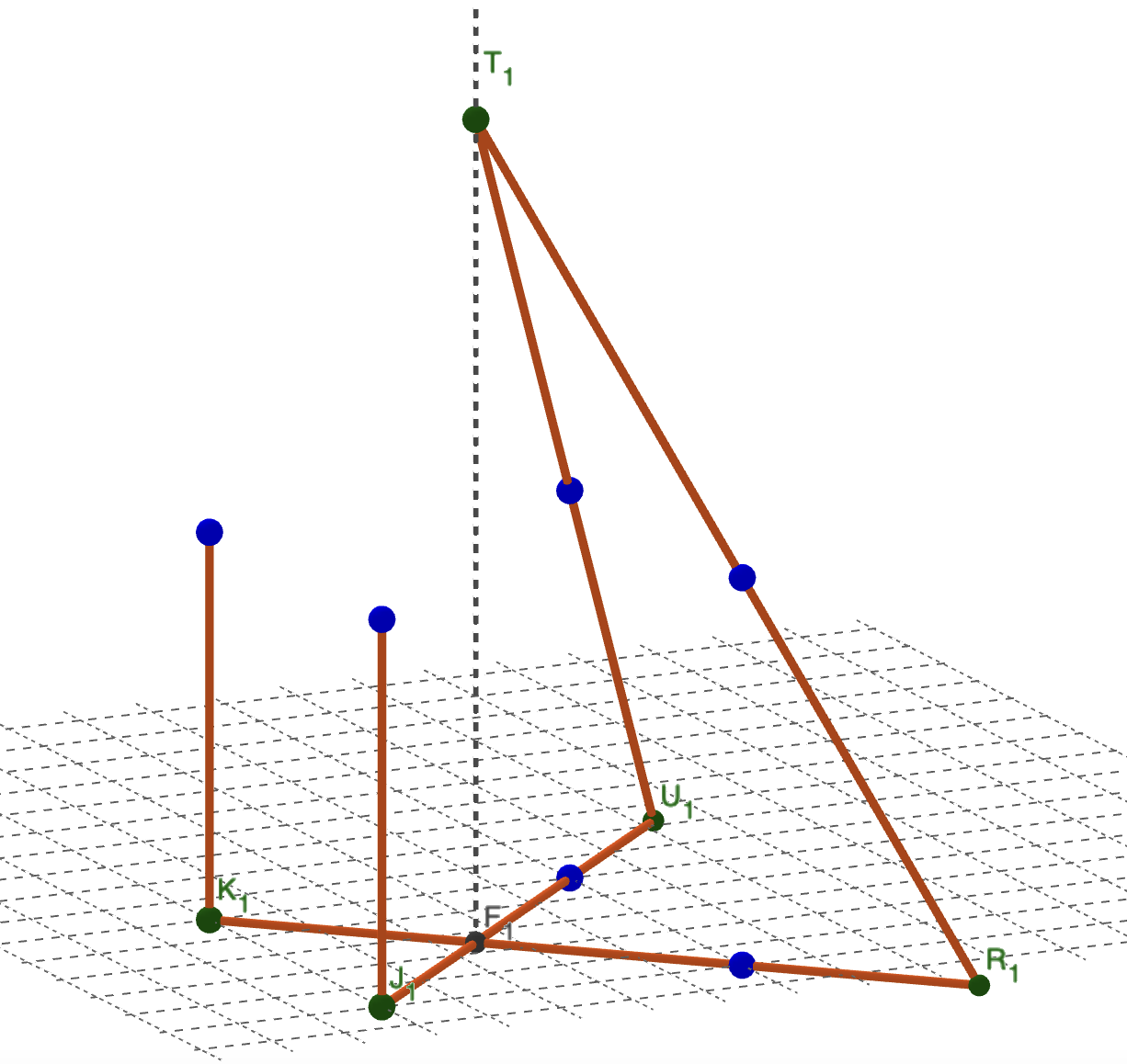
I am trying to find which is the minimum total Euclidean length of all the edges of a minimum-link polygonal chain joining the $8$ vertices of a given cube, located in the Euclidean space. In detail, let the vertices of the mentioned cube be $(0,0,0)$, $(1,0,0)$, $(0,1,0)$, $(0,0,1)$, $(1,1,0)$, $(1,0,1)$, $(0,1,1)$, and $(1,1,1)$.
Now, we know that a minimum-link polygonal chain visiting all the vertices of a cube consists of $6$ (connected) line segments, so I have finally found a valid configuration leading to a total length of $11.105\ldots$ (see Figure below).
Question: Can we prove that the minimum total length achievable (under the given constraints) cannot fall below $11.105$?

Polygonal Chain with 6 edges and a total length of 11.105...

Improve this question
$\endgroup$
7
  • $\begingroup$ "a minimum-link polygonal chain visiting all the vertices of a cube consists of 6 (connected) line segments" - is this proved somewhere? $\endgroup$
    – Max Alekseyev
    Commented Jun 4 at 3:20
  • $\begingroup$ @MaxAlekseyev Yes, it is... for any $k$-dimensional (Euclidean) space, if $k>1$, then the minimum-link poligonal chains have $3 \cdot 2^{k-2}$ edges, as follows by Theorems 2.2 and 2.3 of arxiv.org/pdf/2212.11216 $\endgroup$
    – Marco Ripà
    Commented Jun 4 at 6:43
  • $\begingroup$ What happened? Has the proposed solution posted here been retired or deleted? It has just disappeared... $\endgroup$
    – Marco Ripà
    Commented Jun 6 at 8:04
  • $\begingroup$ That answer was deleted by CommunityBot. I'm not sure what was the reason, but it looks like something happened with its author (banned?). I've cast an undelete vote - hope it'll help. $\endgroup$
    – Max Alekseyev
    Commented Jun 6 at 23:37
  • $\begingroup$ That user was "destroyed". mathoverflow.net/posts/472634/timeline $\endgroup$
    – Gerry Myerson
    Commented Jun 19 at 13:01

1 Answer 1

Reset to default
2
$\begingroup$

My solution for the above-stated problem is provided by the $6$-link polygonal chain $(0,0,1)-(0,0,0)-\big(1+\frac{x+2+\sqrt{2}}{2\cdot\sqrt{2}\cdot(x+\sqrt{2})},1+\frac{x+2+\sqrt{2}}{2\cdot\sqrt{2}\cdot(x+\sqrt{2})},0\big)-\big(\frac{1}{2},\frac{1}{2},1+\frac{x}{\sqrt{2}}\big)-\big(- \frac{x+2+\sqrt{2}}{2\cdot\sqrt{2}\cdot(x+\sqrt{2})},1+\frac{x+2+\sqrt{2}}{2\cdot\sqrt{2}\cdot(x+\sqrt{2})},0 \big)-(1,0,0)-(1,0,1),$
where $x = \frac{1}{2}\cdot \sqrt{\sqrt[3]{\frac{4}{9}} \cdot \sqrt[3]{9+\sqrt{177}}-4 \cdot \sqrt[3]{\frac{2}{27+3\cdot \sqrt{177}}}} + \frac{1}{2}\cdot \sqrt{4 \cdot \sqrt[3]{\frac{2}{27+3\cdot\sqrt{177}}} - \sqrt[3]{\frac{4}{9}}\cdot \sqrt[3]{9+\sqrt{177}} + 4 \cdot \sqrt{\frac{2}{\sqrt[3]{\frac{4}{9}}\cdot\sqrt[3]{9+\sqrt{177}} - 4\cdot \sqrt[3]{\frac{2}{27+3\cdot\sqrt{177}}}}}} = 1.59792093355003207476470535078046555882788360 \ldots$
whose total Euclidean length is equal to $2\cdot \big(1+\frac{1}{\sqrt{2}}+\frac{2+\sqrt{2} \cdot x}{2} \cdot \big( \frac{1}{x} + \sqrt{1+\frac{1}{x^2}}\big) \big) \approx 11.105251123065331797359171121524195.$

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .