In the 1980's, Fintushel-Stern and Fickle independently proved that Brieskorn spheres $\Sigma(2,3,25)$ and $\Sigma(3,5,19)$ bound some Mazur type contractible 4-manifolds with a single $0$-, $1$, and $2$-handle.
I would like to ask that are the 4D Kirby diagrams of these $4$-manifolds known?
The paper of Fickle (not the paper of Gordon to which you link) has an explicit construction for $\Sigma(3,5,19)$. It's behind a paywall but maybe you can get it from interlibrary loan or some kind soul will send you a pdf. Fickle attributes $\Sigma(2,3,25)$ to Casson-Harer, Some homology lens spaces which bound rational homology balls. Since Fickle was Stern's student, I assume he got the attribution right. I think that $\Sigma(2,3,25)$ should appear on the list at the beginning of Fickle's paper but I haven't done the arithmetic. If it's on that list, then it's not hard to write down the desired handle diagram.
The method is the same in both cases; you do surgery on a well-chosen knot in your surgery diagram of the homology sphere to get $S^1 \times S^2$, to which you attach $S^1 \times B^3$. Turning this @Upside Down gives the desired handlebody picture of the Mazur-type manifold. See also the paper of Şavk, Classical and new plumbed homology spheres bounding contractible manifolds and his survey.
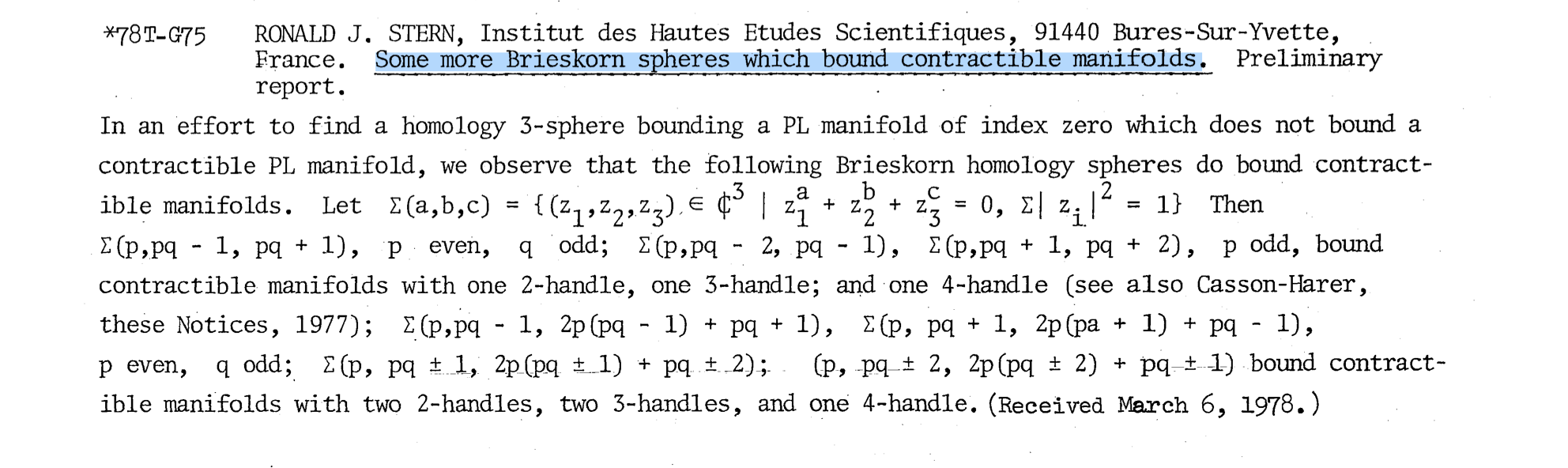
Since it's not that easy to come by, I've attached a screenshot of Stern's announcement about Brieskorn homology spheres from the Notices of the AMS (these days it would be Abstracts). I'm pretty sure it never appeared but Fickle wrote up the results in the aforementioned paper.
