The Klein configuration consists of $60$ points and $60$ planes in $\mathbb C\mathbf P^3$, each point lying on $15$ of the planes and each plane containing $15$ of the points. It appears, among many other places, as the configuration of all reflecting hyperplanes for the unitary reflection group denoted by $G_{31}$ in the Shephard-Todd classification.
A subquestion: I remember having seen mentioned somewhere that this configuration cannot be realized over the reals, does anybody know precise reference?
I am interested in a much smaller configuration, namely, the configuration of all lines and planes of the Klein configuration that pass through one of its 60 points. This is the configuration of all reflection hyperplanes of another Shephard-Todd group, namely the group $G(4,2,3)$ of order $192$, with a presentation $x^4=y^4=z^4=(xyz)^2=1$.
Reducing dimension by one in an obvious way, we are dealing with a configuration in $\mathbb C\mathbf P^2$ consisting of $15$ lines and $31$ points, each line containing $6$ of the points. The points can be subdivided into three classes: there are $12$ "ordinary" points, belonging to $2$ of the $15$ lines only; $16$ triple points, belonging to $3$ of the $15$ lines; and $3$ sixtuple points, belonging to $6$ of the lines each. Also the lines in fact fall into two symmetry classes. There are $3$ "special" lines that do not contain any of the triple points, and $12$ remaining "ordinary" lines.
Question: can this configuration be realized (either in $\mathbb R\mathbf P^2$ or in $\mathbb R\mathbf P^3$) over the reals?
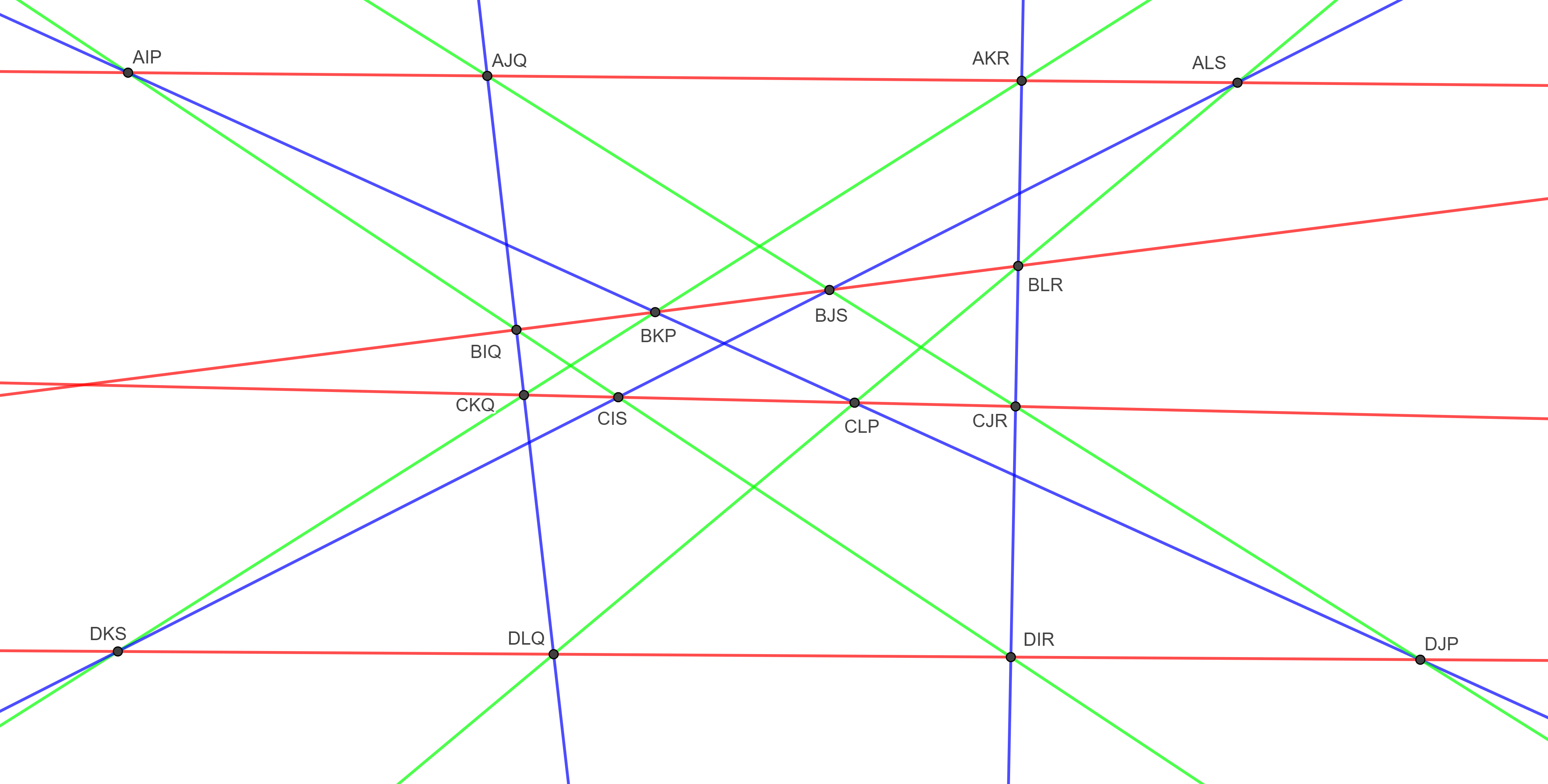
My attempts: I noticed that the $16$ triple points and $12$ ordinary lines form the configuration $16_312_4$, dual to the Reye configuration. This one is surely realizable:
In these terms, a realization of "my" configuration would be achieved if I could arrange the dual Reye configuration in such a way that each of the three quadruples of lines that are of the same color in the picture become concurrent. Indeed, then their intersection points would serve as the remaining three sixtuple points, and three more lines through these points would fulfil what I need. However so far I only managed to make one of the three quadruples (in the picture below, the red one) concurrent:
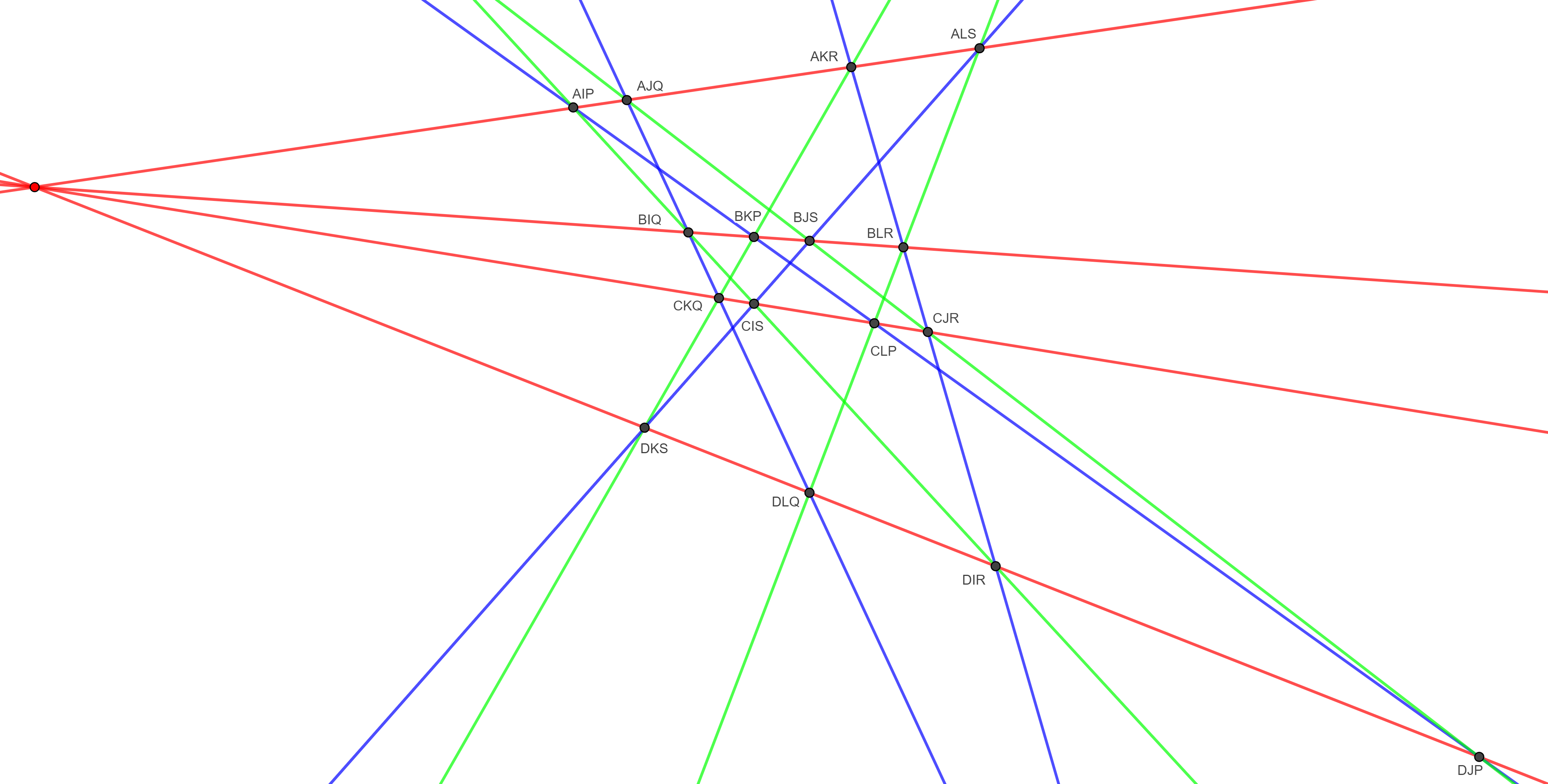
Alternatively, I can start from two quadruples of concurrent lines, but then I can make only two of the four lines from the third quadruple: some of the points that should form the remaining two lines of the third quadruple are not even collinear (below they are depicted by green dashed and dotted pairs of lines, which in fact should form a single dashed and a single dotted line):
After these attempts I became increasingly convinced that no realization is actually possible. I then tried brute force approach, writing down the equations describing the whole thing. However what I got is a huge messy system of polynomial equations in several variables and I have no idea how to find out whether it admits any real solutions.
So...?
Just in case, here is one possible complex version of the configuration. The $3$ special lines are those $[x:y:z]\in\mathbb C\mathbf P^2$ with $x=0$, $y=0$ and $z=0$ respectively, while the remaining $12$ lines have equations $y=x$, $y=-x$, $y=ix$, $y=-ix$, $z=y$, $z=-y$, $z=iy$, $z=-iy$, $z=x$, $z=-x$, $z=ix$, $z=-ix$. All possible pairwise intersections of these $15$ lines form the $31$ points of the configuration.