Does there exist a shot in ideal pocket billiards?

Question

Assume you have one shot with the cue ball in pocket billiards (a.k.a. pool), with the game idealized in that no spin is placed on the cue ball in the initial shot, all collisions between billiard balls, and balls with the cushions, are perfectly elastic, and there is arbitrarily small felt-ball rolling friction so that reflections may be assumed to continue as long as necessary.

Q1. Is there always some direction in which to shoot the cue ball so that some ball goes into a pocket (is sunk), and although other balls also may be sunk, the cue ball itself is not (so there is no scratch)?

My sense is that the answer should be 'Yes' because irrationally angled paths will eventually visit pockets. But the presence of up to 15 balls, and the requirement that the cue ball not scratch, seems to leave open the possibility that there is some initial configuration of the balls that thwarts every possible shot. It might be necessary to assume that the cue ball is initially not touching any other ball?

Two other variations:

Q2. Is there always a shot to sink a particular ball $B^*$?

Q3. Is there always a shot to sink a particular ball $B^*$ and no other ball?

The latter might be too strong, as all six pockets could conceivably be "protected" by non-$B^*$ balls.

Answer 1

How about the following? It suggests the answer to Q1 is no.

The 2,15,6,9 and cueball are in a horizontal row and touching (or very nearly touching). The 14,cueball and 4 are in a vertical row and (very nearly) touching.

When the cueball is hit, one or both of the following will happen (depending on which direction the cueball is hit).

• The cueball moves horizontally to the right.
• The 4 moves vertically downwards, hitting the 8-ball, which travels down the table, off the bottom cushion and back up the table, off the left cushion and hits the 15 head on and slightly from the left (the angle in my illustration is probably not accurate though). The 8 and 15 will come back down the table, while the cueball is knocked horizontally to the right along with the 9 and, possibly, the 6.

In any case, the white should go in the corner pocket off the 3.

[Edit: The layout has been modified since the initial revision, but it is still the same idea.]

We need to be careful here and check that something unintended doesn't happen, like the 8,15 or 4 going in a pocket before the white. This seems unlikely and, in any case, we can put extra balls on the table to block their path if necessary.

There is still one problem though. If the cueball is hit at a specific angle, the white will start moving horizontally towards the 3, and the 9 will follow it shortly afterwards. What happens if, just after the cueball deflects off the 3, the 9 catches up and hits it? Maybe this is enough to deflect it away from the pocket? I'm not sure about this. Maybe the setup can be simplified a bit.

[The pool table above was copied from Wikimedia commons]

---

Here's a simpler example. The white ball is hemmed in by the top-right corner pocket. All you can do is to either hit it straight into the pocket, or against the 7 or 14. If the 7 is hit, it rolls along the top cushion into the 3, which bounces off the left cushion into the 12, which rolls towards towards the top right corner, pocketing the white. Similarly, hitting the 14 will knock the 9 towards the bottom cushion and back up into the 8, which again pockets the white.

Answer 2

This isn't the answer you seek, but let me observe merely that if you allow extra balls and if the table width is an integer number of balls in each direction, then we may imagine a cross pattern with the cue ball at the intersection.

/------------------\
|         O        |
|         O        |
|         O        |
|         O        |
|OOOOOOOOOCOOOOOOOO|
|         O        |
(         O        )
|         O        |
|         O        |
|         O        |
|         O        |
|         O        |
\------------------/

Under your idealized physical interactions, it seems that the cue ball cannot move, since the forces acting on the other balls are all transverse.

Probably one can also imagine other highly-packed arrangements.

Answer 3

I think that the answer to Q1 is "no" if we allow the problem to be generalized sufficiently, (e.g. we allow large pockets, and small balls) even if no balls are touching in the initial configuration. Consider the following "billiard table": the entire rectangular wall consists of "pocket" except for a single point at the midpoint of one of the walls.

Now place a single red ball in the very center of the square, and place the cue ball on the line connecting the ball and the "wall point," on the opposite side of the ball from the "wall point."

Then we have no opportunity to ricochet, as red ball blocks the cue's path to the "wall point." So we must hit the red ball immediately--but it is easy to see that for balls of small diameter, whatever shot we attempt will result in a scratch.

Now, it seems to me that the condition of "scratching" is an open condition in the configuration space of the problem (e.g. parametrize by wall endpoints, ball positions, felt damping coefficient, shot angle, etc.--here I assume walls are possibly empty closed intervals and balls are closed balls) so this counterexample gives infinitely many (slightly less trivial) counterexamples.

EDIT: George Lowther correctly points out that this construction does not work for all sizes of billiard tables, so I'll be a bit more explicit about what I have in mind. Let the diameter of the balls be $d$ and let the table be of size $R\times r$ where $r<2d$. Put one corner of the table at $(0,0)$ with the sides of the table parallel to the coordinate axes and the side of length $R$ along the $y$-axis. Put the red ball at $(r/2, R/2)$ and the cue ball at, say, $(r/2, R/4)$. Let the wall be "all pocket" except for a point at $(r/2, R)$, which is made of "wall material".

Say a ball goes into the pocket if the entire ball crosses the boundary of the pocket. Now to sink the red ball it must move at least $r/2+d/2$ to the right or left (one cannot sink it along the $x$-axis, as it would have to pass through the cue ball to do so). But then by conservation of momentum, the cue would have to move at least $r/2+d/2$ in the opposite direction, which would be a scratch. (Indeed, the cue actually scratches before the red ball goes in, I believe).

Answer 4

edit 1 $\to$ 2 ** This might or might not be the answer... (but I believe the answer is **no. to your Q1) :

Is there always some direction in which to shoot the cue ball so that some ball goes into a pocket (is sunk), and although other balls also may be sunk, the cue ball itself is not (so there is no scratch)?

This is also not an answer you seek, however this is a furtherance of a comment I made above, where I agree with Daniel Litt that with enough pockets, the cue ball will ultimately scratch. In fact, as long as there is more than zero pockets, it is always very likely that the cue ball will ultimately scratch, except for precisely finicky situations. I shall construct such an example.

Also, with Joseph's conditions, no momentum is lost with each ball-to-ball contact, thus the white ball will always have to keep moving. In fact, it's almost an analog (real, in $\mathbb{R}^2$) analogue to a discrete stochastic matrix: if there are any elements of the Markov chain which are absorbing states, then ultimately everything will be absorbed. Even if there is only one pocket (or just the standard six), the cue ball will ultimately end up in one of the pockets for this Q's criteria, unless you set it up just right.

The pockets are analogous to the aborbing states in the stochastic matrix of the Markov chain.

The exception to the absorbing states' always absorbing all possibilities occurs if there is a cycle in the Markov chain. Here is an example where you end up with an infinite loop, equivalent to a cycle in a Markov chain.

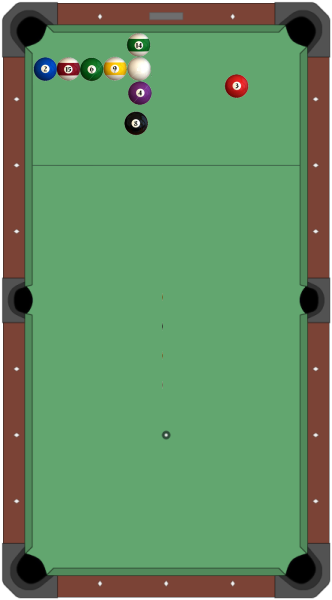
• Your pool table is deterministic with length $y_t$ (along the long axis of the table) and width $x_t$, with pockets at the four corners and along the sides at $x=0$ and $x=x_t$

• Set the cue ball up at $(x_c, y_c)$, with $x_c$ greater than half-the diameter of the ball + the width of the corner pockets $\times \sqrt(2)$

• set one more ball $B$ at $(x_c, y_b)$ such that the cue ball and this ball are aligned at their x-position and non-overlapping in their y-position ($y_c - y_b \gt$ diameter of balls $+ \varepsilon$ for very tiny $\varepsilon$)

• place all other balls at $(x_d, y_{something})$ such that $x_c - x_d \gt$ diameter of balls $+ \varepsilon$, and these other balls are not overlapping, e.g. $y_i - y_j \gt$ diameter of balls $+\varepsilon$ for each different $i,j$ pair of two balls in the remaining balls.

• give the cue ball an impulse such that it moves with $v_x = 0$ and $v_y \gt 0$

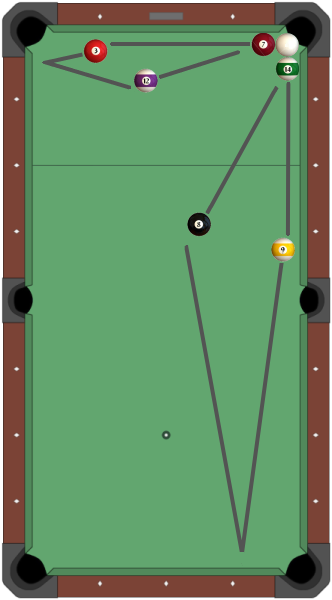
• (infinite loop starts here)

• now the cue ball will move up until it hits the ball $B$, which occurs for the first time at $(x_c, y_b - \textrm{diameter} \div 2)$ as the point of contact for the two balls

• momentum is transferred to the ball $B$ which moves with no motion along the x-axis, and positive along the y-axis until it hits the far end of the table

• $B$ reflects back until it hits the cue ball, imparting a negative y-velocity to it

• cue ball will hit the near wall and reflects back to hit ball $B$

• ad infinitum

So this doesn't answer your question, but does prove that a scenario exists in a deterministic perfect momentum-conserving billiards game such that an oscillatory cycle is entered and never ends.

So with your conditions that we can make the rolling friction arbitrarily small, we'd end up scratching even if a configuration were found that was capable of sinking all fifteen balls and then the cue ball would be alone on a table moving until it fell into one of the pockets. Unless we could adjust $\mu$ for each starting configuration so that after sinking all fifteen balls, the cue ball glides to a stop. But that's just setting up too many parameters and free variables. This is why physicists recommend that we not live in a world without friction.

(edit 2 bold claim retracted by strikethroughs) Actually, I've reread your revised question, and I think that this actually is the answer you seek, and the answer is no. There is no shot that you can make in the game on the table you have defined such you will sink the specified ball and also not scratch. Your conditions make it such that if you do not enter an infinite loop, the cue ball will keep moving. To hit a ball into a pocket, the cue ball will cycle around and find a changed world, and will most likely end up scratching ultimately. and if you allow rolling friction $\mu_R$ to be a specifiably small value, perhaps the answer is yes. But the condition of allowing 14 other balls in the mix turns it into an extended type of problem like the 3-body problem for gravitational attraction, with wacky chaotic dynamics that are not easily tractably gotten around. ...